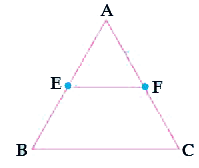
Draw a triangle ABC and mark the midpoints E and F of two sides of triangle. ¯¯¯¯¯¯ABand¯¯¯¯¯¯AC respectively. Join the point E and F as shown in the figure.
Measure EF and the third side BC of the triangle. Also measure ∠AEFand∠ABC.
We find ∠AEF=∠ABCand¯¯¯¯¯¯EF=12¯¯¯¯¯¯BC
As these are corresponding angles made by the transversal AB with lines EF and BC, we say EF||BC.
Solution in Telugu
Step by step video & image solution for Draw a triangle ABC and mark the midpoints E and F of two sides of triangle. bar(AB) and bar(AC) respectively. Join the point E and F as shown in the figure. Measure EF and the third side BC of the triangle. Also measure angleAEF and angleABC. We find angleAEF = angleABC and bar(EF)=(1)/(2)bar(BC) As these are corresponding angles made by the transversal AB with lines EF and BC, we say EF"||"BC. by Maths experts to help you in doubts & scoring excellent marks in Class 9 exams.
Topper's Solved these Questions
- BOOK - NCERT TELUGUCHAPTER - QUADRILATERALSEXERCISE - EXERCISE - 8.110videos
QUADRILATERALS
- BOOK - NCERT TELUGUCHAPTER - QUADRILATERALSEXERCISE - EXERCISE - 8.23videos
QUADRILATERALS
- BOOK - NCERT TELUGUCHAPTER - QUADRILATERALSEXERCISE - THINK, DISCUSS AND WRITE2videos
QUADRILATERALS
- BOOK - NCERT TELUGUCHAPTER - PROOFS IN MATHEMATICSEXERCISE - EXERCISE - 15.49videos
PROOFS IN MATHEMATICS
- BOOK - NCERT TELUGUCHAPTER - REAL NUMBERSEXERCISE - Exercise 1.411videos
REAL NUMBERS
Similar Questions
State whether the statements are True or False.
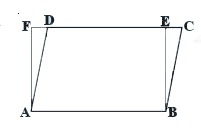
(i) Every parallelogram is a trapezium ( )03:37View SolutionIn the adjacent figure ABCD is a parallelogram ABEF is a rectangle show that ΔAFD≅ΔBEC.
07:49View SolutionShow that the diagonals of a square are equal and right bisectors of each other.
02:29View SolutionState which of the following are mathematical statements and which are not? Give reason.
She has blue rays01:47View SolutionSimplify the following expressions .
i) (5+√7)(2+√5)
ii) (5+√5)(5−√5)
iii) (√3+√7)2
iv) (√11−√7)(√11+√7)06:45View SolutionABC is an equilateral triangle of side ‘a'. Then bar(AB).bar(BC) +bar(BC).bar(CA)+ bar(CA).bar(AB) =
02:39View SolutionLet ABC be a triangle. Three points D, E and F are on the sides AB, AC and BC respectively so that BDEF is a parallelogram. M and N are mid points of the sides DE and EF. If vector ¯¯¯¯¯¯¯¯MN=14¯¯¯¯¯¯AC. Then find the ratio in which D and F divides the sides AB and BC.
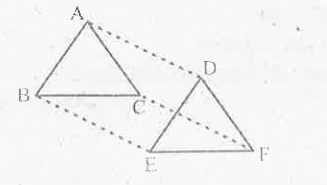
04:44View Solution△ ABC ಮತ್ತು △ DEF ಗಳಲ್ಲಿ AB =DE, AB∣∣DE, BC = EF ಮತ್ತು BC∣∣ EF ಆಗಿದೆ. A, B ಮತ್ತು C ಶೃಂಗಗಳನ್ನು ಕ್ರಮವಾಗಿ D,E ಮತ್ತು Fಶೃಂಗಗಳಿಗೆ ಸೇರಿಸಿದೆ (ಚಿತ್ರ ಗಮನಿಸಿ). ಚತುರ್ಭುಜ ABED ಒಂದು ಸಮಾಂತರ ಚತುರ್ಭುಜ.
. 05:43View SolutionDelta ABC Draw a triangle. bar AB And bar AC Mark E and F as midpoints. Combine E and F as shown in the diagram. Measure the EF measure in the triangle and the BC in the third shoulder. In the same way /_AEF And /_ABC Combine angles. To us /_AEF = /_ABC And bar 1/2 bar BC That comes. We can say EF // BC because these angles are identical angles formed by the transverse line AB on the lines EF and BC. Draw a few more triangles and check the results.
03:04View SolutionDraw a triangle ABC and mark the midpoints E and F of two sides of triangle. ¯¯¯¯¯¯ABand¯¯¯¯¯¯AC respectively. Join the point E and F as shown in the figure.
Measure EF and the third side BC of the triangle. Also measure ∠AEFand∠ABC.05:14View SolutionIf D is the midpoint of the side BC of a triangle ABC then ¯¯¯¯¯¯AB2+¯¯¯¯¯¯AC2=
03:36View SolutionABC is an equitateral triangle of side 'a'. Then ¯¯¯¯¯¯AB.¯¯¯¯¯¯BC+¯¯¯¯¯¯BC.¯¯¯¯¯¯CA+¯¯¯¯¯¯CA.¯¯¯¯¯¯AB=
03:02View SolutionThe points D, E, F are the midpoints of the sides ¯¯¯¯¯¯BC,¯¯¯¯¯¯CA,¯¯¯¯¯¯AB of ΔABC respectively. If A=(-2,3,4), D =(1,-4,2), E =(-5,2,-3) then F=
01:59View SolutionIn ΔABC and ΔDEF , AB = DE, AB∣∣DE, BC = EF and BC∣∣EF. Vertices A, B and C are joined to vertices D, E and F respectively (See fig.)
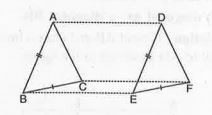Show that quadrilateral ABED is a parallelogram.03:26View SolutionIn ΔABC and ΔDEF , AB = DE, AB∣∣DE, BC = EF and BC∣∣EF. Vertices A, B and C are joined to vertices D, E and F respectively (See fig.)
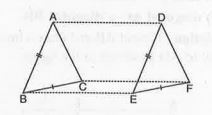Show that AC=DF.02:53View Solution