Question

Let $T$
be the line
passing through the points $P(-2,7)$
and $Q(2,-5)$
. Let $F_1$
be the set
of all pairs of circles $(S_1,S_2)$
such that $T$
is tangent
to $S_1$
at $P$
and tangent
to $S_2$
at $Q$
, and also
such that $S_1$
and $S_2$
touch each
other at a point, say, $M$
. Let $E_1$
be the set
representing the locus of $M$
as the pair
$(S_1,S_2)$
varies in $F_1$
. Let the
set of all straight lines segments joining a pair of distinct points of $E_1$
and passing
through the point $R(1,1)$
be $F_2$
. Let $E_2$
be the set
of the mid-points of the line segments in the set $F_2$
. Then,
which of the following statement(s) is (are) TRUE?
The point $(-2,7)$
lies in $E_1$

(b) The
point $(\frac{4}{5},\frac{7}{5})$
does NOT lie
in $E_2$

(c) The
point $(\frac{1}{2},1)$
lies in $E_2$

(d) The
point $(0,\frac{3}{2})$
does NOT lie
in $E_1$

Options

A. The point `(-2,7)` lies in `E_(1)`
B. The point `(4//5,7//5)` does NOT lie in `E_(2)`
C. The point `(1//2,1)` lie in `E_(2)`
D. The point `(0,3//2)` does NOT lie in `E_(1)`

Answer

2,4

Explanation

According to the question , we have the following figure `:`

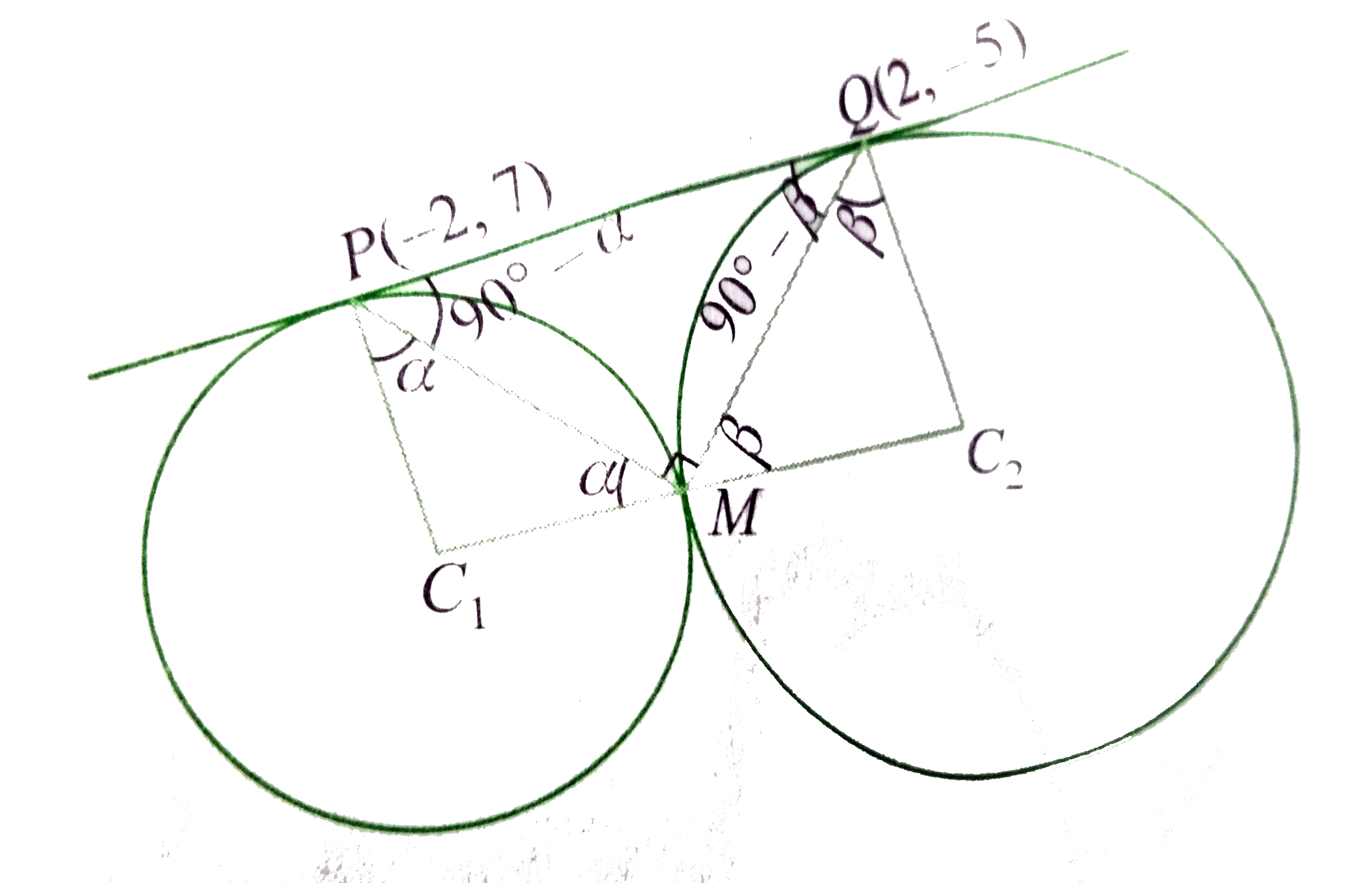
Clearly, `/_ PMQ =(pi)/(2)`.
Hence, locus of M is
`(x+2)(x-2)+(y-7)(y+5)=0`
or `E _(1) -= x^(2)+y ^(2)-2y-39=0` (i)
Clearly, `E_(2)` is the locus of mid-points of chords of `E_(1)` passing through (1,1).
Let the mid-pioint of chord of `E_(1)` be (h,k) .
Its equation is
`h+k- (1+k) -39= h^(2)+k^(2)-2k-39`
or `h^(2)+k^(2)-2k-h+1=0`
Hence, `E_(2) -= x^(2)+y^(2)-x-2y+1=0` (ii) ltbrlt Operation (A) is incorrect, although, it satisfies eq. (i) otherwise the line T would touch the second circle at two points.
Als, `(4//5,7//5)` satisfies eq. (ii) but again in this case one end of the chord would be `(-2,7)` which is not included in `E_(1)`.Therefore, `(4//5, 7//5)` does not lie in `E_(2)`
`(1/2,1)` does not satisfy eq. (ii), therefore, it does not lie in `E_(2)`.
`(0,3//2)` does not satisfy eq. (i), so it does not lie in` E_(1)`.