A circle whose diameter is major aixs of ellipe x2a2+y2b2=1(a>b>0) meets axis at point P. Ifthe orthocentre of ΔPF1F2 lies on ellipse where F1andF2 are foci of ellipse , then find the eccenricity of the ellipse
The correct Answer is:√√5−12
In the figure, B is orthocentre.
Slope of altitude SB,m1=bae
Slope of SA,m2=aae
Since m1×m2=1, we have
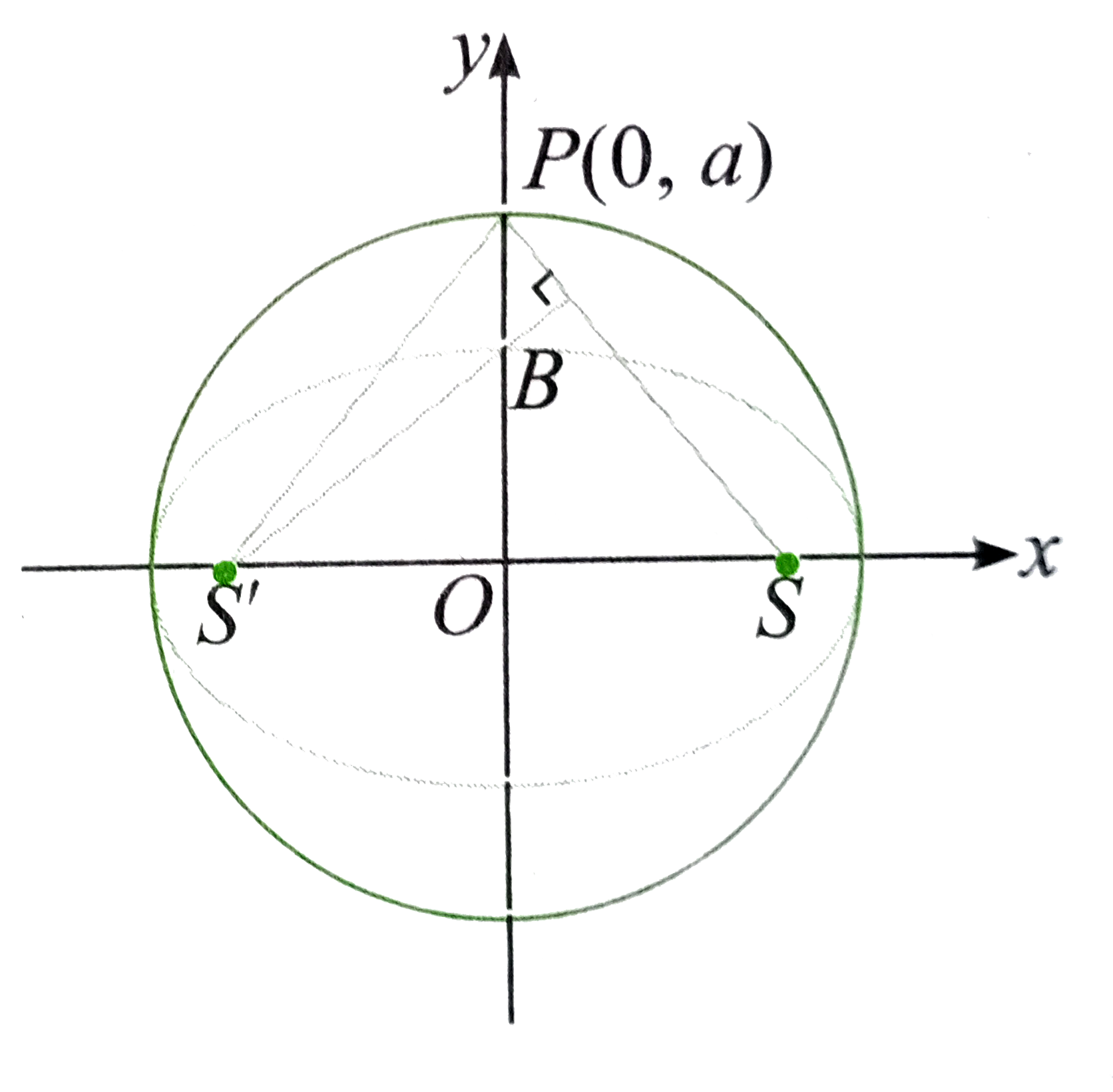
bbe(1−e)=−1
⇒1−e2=e4
⇒e4+e2−1==0
⇒e2=√5−12
⇒e=√√5−12
Similar Questions
A circle whose diameter is major aixs of ellipe x2a2+y2b2=1(a>b>0) meets minor axis at point P. Ifthe orthocentre of ΔPF1F2 lies on ellipse where F1andF2 are foci of ellipse , then find the eccenricity of the ellipse
View SolutionEllipse x2a2+y2b2=1(a>b) Equation of the circle described on major axis as diameter is
View Solution
Knowledge Check
If the circle whose diameter is the major axis of the ellipse x2a2+y2b2=1(a>b>0) meets the minor axis at point P and the orthocentre of ΔPF1F2 lies on the ellipse, where F1andF2 are foci of the ellipse, then the square of the eccentricity of the ellipse is
A√5−12B√3−1C1√2D√32If F1(-3, 4) and F2 (2, 5) are the foci of an ellipse passing through the origin, then the eccentricity of the ellipse is
A√295+√29B√215+√29C√265+√29D√295+√21F1andF2 are the two foci of the ellipse x29+y24=1. Let P be a point on the ellipse such that |PF1|=2|PF2|, where F1andF2 are the two foci of the ellipse . The area of △PF1F2 is :
A3B4C√5D√132
If normal at any poin P on the ellipse x2a2+y2b2=1(a>b>0) meets the major and minor axes at Q and R, respectively, so that 3PQ=2PR, then find the eccentricity of ellipse
View SolutionIf normal at any poin P on the ellipse x2a2+y2b2=1(a>b>0) meets the major and minor axes at Q and R, respectively, so that 3PQ=2PR, then find the eccentricity of ellipse
View SolutionF1andF2 are the two foci of the ellipse x29+y24=1. Let P be a point on the ellipse such that |PF1|=2|PF2|, where F1andF2 are the two foci of the ellipse . The area of △PF1F2 is :
View SolutionF1andF2 are the two foci of the ellipse x29+y24=1. Let P be a point on the ellipse such that |PF1|=2|PF2|, where F1andF2 are the two foci of the ellipse . The area of △PF1F2 is :
View SolutionIf normal at any point P to the ellipse x2a2+y2b2=1(a>b) meets the axes at A and B such that PA:PB=1:2, then the equation of the director circle of the ellipse is
View Solution
