The side of a square is 10 cm. Find the area of circumscribed and inscribed circles.
The correct Answer is:(i) 78.5cm2 (ii) 157cm2
Diameter of the inscribed circle = side of the square =10cm
∴ radius of the inscribed circle =5cm
Diameter of the circumscribed circle = diagonal of the square
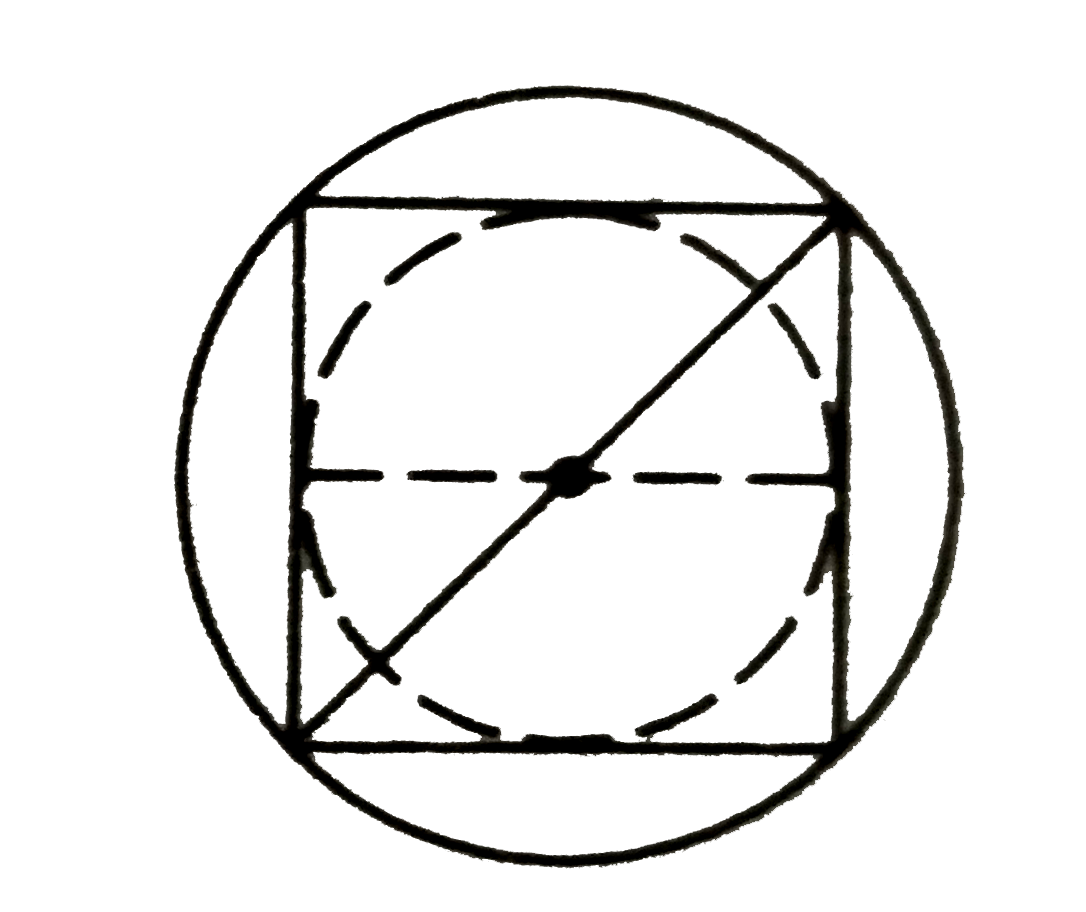
=(√2×10)cm
∴ radius of the circumscribed circle =5√2cm
Topper's Solved these Questions
- AREA OF CIRCLE, SECTOR AND SEGMENT BOOK - RS AGGARWALCHAPTER - AREA OF CIRCLE, SECTOR AND SEGMENT EXERCISE - EXERCISE
1 Videos
- AREA OF CIRCLE, SECTOR AND SEGMENT BOOK - RS AGGARWALCHAPTER - AREA OF CIRCLE, SECTOR AND SEGMENT EXERCISE - Exercise 16B
51 Videos
- AREA OF CIRCLE, SECTOR AND SEGMENT BOOK - RS AGGARWALCHAPTER - AREA OF CIRCLE, SECTOR AND SEGMENT EXERCISE - SOLVED EXAMPLE_TYPE
1 Videos
- ARITHMETIC PROGRESSIONBOOK - RS AGGARWALCHAPTER - ARITHMETIC PROGRESSIONEXERCISE - Multiple Choice Questions (Mcq)
30 Videos
Similar Questions
Knowledge Check
The length of the side of a square is 14 cm. Find out the ratio of the radii of the inscribed and circumscribed circle of the square.
AA)√2:1BB)1:√2CC)√2:3DD)2:1If the side of a square is 24 cm, then the circumference of its circumscribed circle ( in cm ) is :
A24√3πB24√2πC12√2πD24πIf the side of a square is 24 cm, then the circumference of its circumscribed circle ( in cm ) is :
A24√3πB24√2πC12√2πD24π
If side of a square is 10 cm. Find ratio of area of circumcircle and In circle
View SolutionA square circumscribes a circle and another square is inscribed in this circle with one vertex at the point of contact. The ratio of the areas of the circumscribed and the inscribed squares is (a) 1 (b) 2 : 1 (c) 3 (d) 4
View SolutionThe circumference of a circle is 50 cm. Find the side of the square inscribed in the circle.
View SolutionThe difference of the area of the circumscribed and the inscribed square of a circle is 35 sq. cm. Find the area of the circle.
View SolutionA square circumscribes a circle and another square is inscribed in this circle with one vertex at the point of contact. The ratio of the areas of the circumscribed and the inscribed squares is (a) 1 (b) 2 : 1 (c) 3 (d) 4
View Solution
