(a) Draw the ray diagram showing refraction of ray of light through a glass prism. Derive the expression for the refractive index n of the material of prism in terms of the angle A ray angle of minimum deviation $δ_{m}$
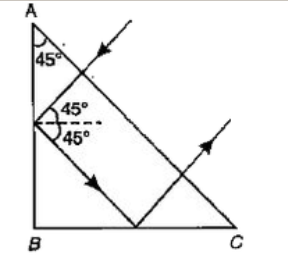
(b) A ray of light PQ enters an isosceles right angles prism ABC of refractive index 1.5 as shown in figure .
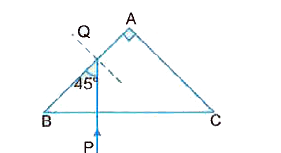
$(a) Draw the ray diagram showing refraction of ray of light through a glass prism. Derive the expression for the refractive index n of the material of prism in terms of the angle A ray angle of minimum deviation deltam (b) A ray of light PQ enters an isosceles right angles prism ABC of refractive index 1.5 as shown in figure . (i) Trace the path of the ray through the prism . (ii) What will be the effect on the path of the ray if refractive index of the prism is 1.4 ?$
(i) Trace the path of the ray through the prism .
(ii) What will be the effect on the path of the ray if refractive index of the prism is 1.4 ?

Text Solution

Verified by Experts

(a) Refraction of light rays through a prism has been shown below .
Here $∠ i$ is the incidence angle at first surface of prism and $∠ e$ is the angle of emergence from the second surface $∠ r_{1} and ∠ r_{2}$ are the respective refraction angles at the two faces and $∠ δ$ is the angle of deviation.
Now in $Δ Q M R, ∠ r_{1} + ∠ r_{2} + ∠ M = 180^{\circ} ... (i)$
and in quadrilateral AQMR, $∠ A + 90^{\circ} + ∠ M + 90^{\circ} = 360^{\circ}$
or $∠ A + ∠ M = 180^{\circ} ... (i i)$

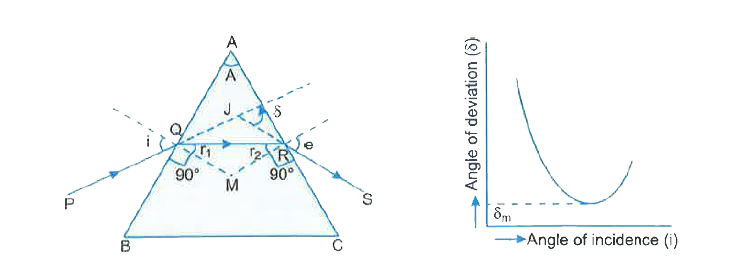
Comparing (i) and e(ii), we get
$∠ r_{1} + ∠ r_{2} = ∠ A ... (i i i)$
Now in $Δ J Q R ∠ δ = ∠ J Q R + ∠ J R Q$
$= (∠ i - ∠ r_{1}) (∠ e - ∠ r_{2}) = ∠ r + ∠ e - (∠ r_{1} + ∠ r_{2}) = ∠ i + ∠ e - ∠ A$
$\Rightarrow ∠ i + ∠ e = ∠ A + ∠ δ ... . (i v)$
A graph showing variation in angle of deviation $(δ)$ with variation in angle of incidence (i) is also shown below . As minimum deviation position corresponds to only one angle of incidence, it means that for minimum deviation position $∠ i = ∠ e$ and also $∠ r_{1} = ∠ r_{2} = r$ (say) .
So in minimum deviation position , we have
$∴ ∠ A = ∠ r_{1} + ∠ r_{2} = ∠ r + ∠ r = 2 ∠ r or ∠ r = (\frac{A}{2})$
and $∠ A + ∠ δ_{m} = ∠ i + ∠ e = ∠ i + ∠ i = 2 ∠ i or ∠ i = (\frac{A + δ_{m}}{2})$
$∴$ Refractive of prism $n = \frac{sin i}{sin r} = \frac{sin (\frac{A + δ_{m}}{2})}{sin (\frac{A}{2})}$
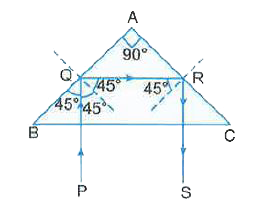
(b) (i) Complete path of the ray through the prism has been traced. Since critical angle of prism is $i_{c} = {sin}^{- 1} (\frac{1}{1.5}) = 42^{\circ}$ , the light ray undergoes total internal reflection two times as shown .

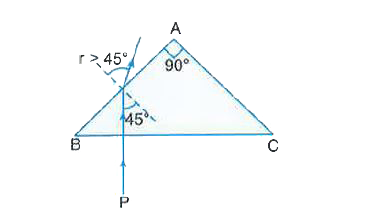
(ii) If refractive index of prism is n = 1.4 , then critical angle $i_{c} = {sin}^{- 1} (\frac{1}{1.4}) = {45.6}^{\circ}$ . In that case the light ray will be refracted into air at point Q and deviates away from the normal as shown below .

Updated on:21/07/2023

When a ray of light falls on a prism of angle $48^{\circ}$ , it suffers minimum deviation. Refractive index of the material of prism is $1.6$ . The angle of incidence is nearly
A $16^{\circ}$
B $48^{\circ}$
C $30^{\circ}$
D $41^{\circ}$
The angle of a prism is $60^{\circ}$ and its refractive index is $\sqrt{2}$ . The angle of minimum deviation suffered by a ray of light in passing through it is
AAbout $20^{\circ}$
B $30^{\circ}$
C $60^{\circ}$
D $45^{\circ}$
A ray of light is incident at an angle of $60^{\circ}$ on the face of a prism with an angle of $60^{\circ}$ . Then the refractive index of the material of the prism is (the prism is in minimum deviation position)
A1.414
B1.623
C1.524
D1.732