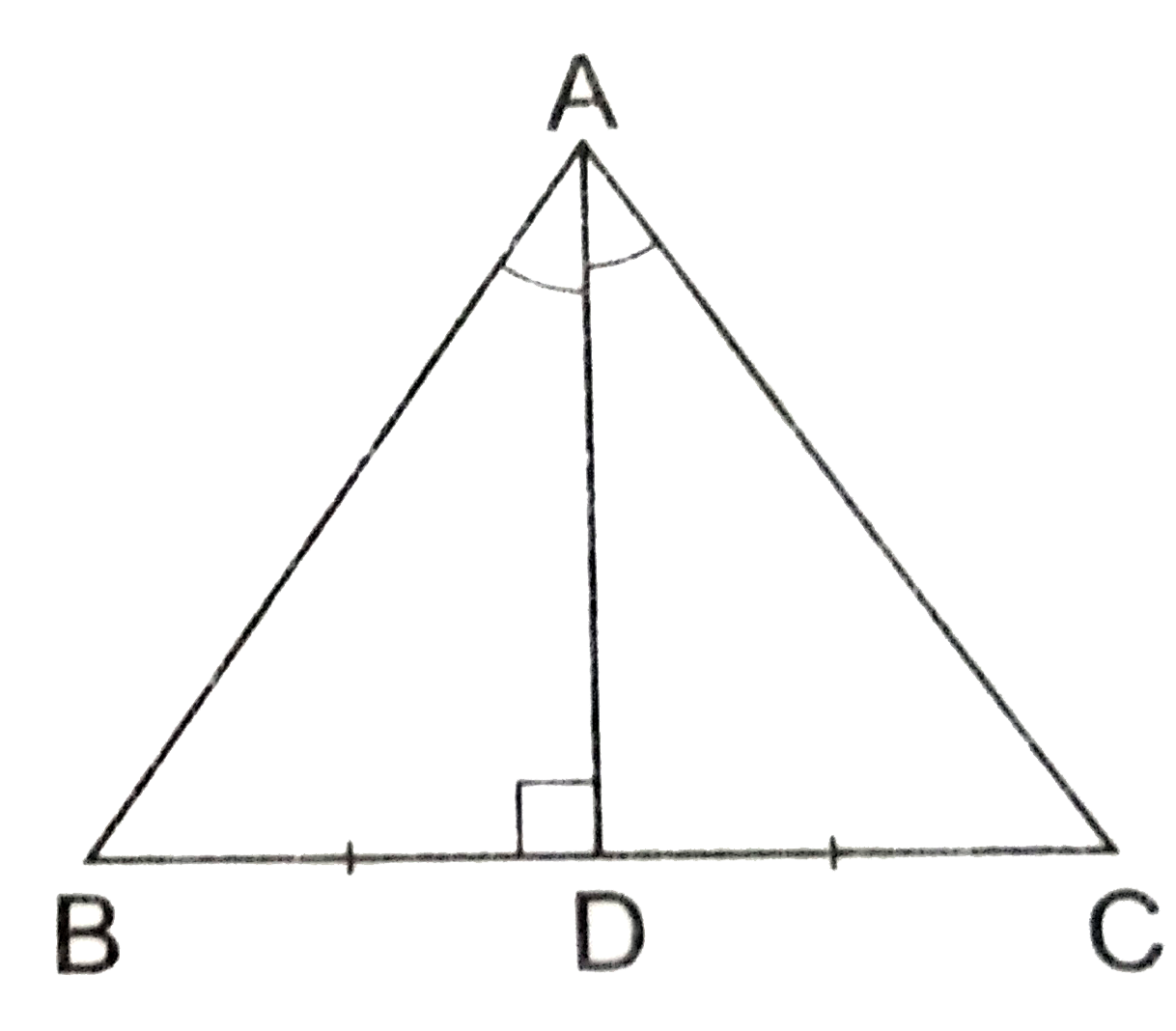
In an equilateral triangle ABC, if AD⊥BC then which of the following is true?
A
2AB2=3AD2
B
4AB2=3AD2
C
3AB2=4AD2
D
3AB2=2AD2
Video Solution
Text Solution
The correct Answer is:C
AB2=BD2+AD2
=(12AB)2+AD2=14AB2+AD2
⇒34AB2=AD2⇒3AB2=4AD2
|
Topper's Solved these Questions
- TRIANGLESBOOK - RS AGGARWALCHAPTER - TRIANGLESEXERCISE - MULTPILE-CHOICE QUESTIONS (MCQ) ( MATCHING OF COLUMNS)
1 Videos
- TRIANGLESBOOK - RS AGGARWALCHAPTER - TRIANGLESEXERCISE - Test Yourself
20 Videos
- TRIANGLESBOOK - RS AGGARWALCHAPTER - TRIANGLESEXERCISE - Exercise 7E
30 Videos
- T-RATIOS OF SOME PARTICULAR ANGLESBOOK - RS AGGARWALCHAPTER - T-RATIOS OF SOME PARTICULAR ANGLESEXERCISE - Exercise 11
27 Videos
- TRIGONOMETRIC IDENTITIESBOOK - RS AGGARWALCHAPTER - TRIGONOMETRIC IDENTITIESEXERCISE - Test Yourself
20 Videos
Similar Questions
Knowledge Check
In an equilateral ΔABCif AD⊥BC, then
A2AB2=3AD2B3AB2=4AD2C5AB2=6AD2D4AB2=5AD2In the given figure, AB = BC, AD = CD. Then, which of the following is true?
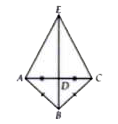 A∠ADE=90∘BAE=ECCBoth (a) and (b)DAE=BC
A∠ADE=90∘BAE=ECCBoth (a) and (b)DAE=BCIn a △ABC,AB = AC and AD⊥BC, then :
AAB<ADBAB>ADCAB=ADDAB≤AD
AD is an altitude of an equilateral triangle ABC. On AD as base, another equilateral triangle ADE is constructed. Prove that Area ( triangle ADE ): Area ( triangle ABC )=3:4.
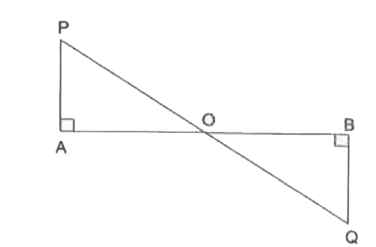
View SolutionIn the given figure, PA⊥AB, QB⊥AB and PA=QB.
Which of the following is true?
View SolutionΔABC is an equilateral triangle such that AD⊥ BC, then AD2=
View SolutionIn an equilateral triangle ABC, the side BC is trisected at D. Find the value of AD2 :
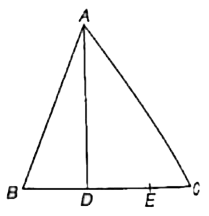
View SolutionIn an equilateral triangle ABC of side 10cm, the side BC is trisected at D. Then the length (in cm) of AD is
View Solution