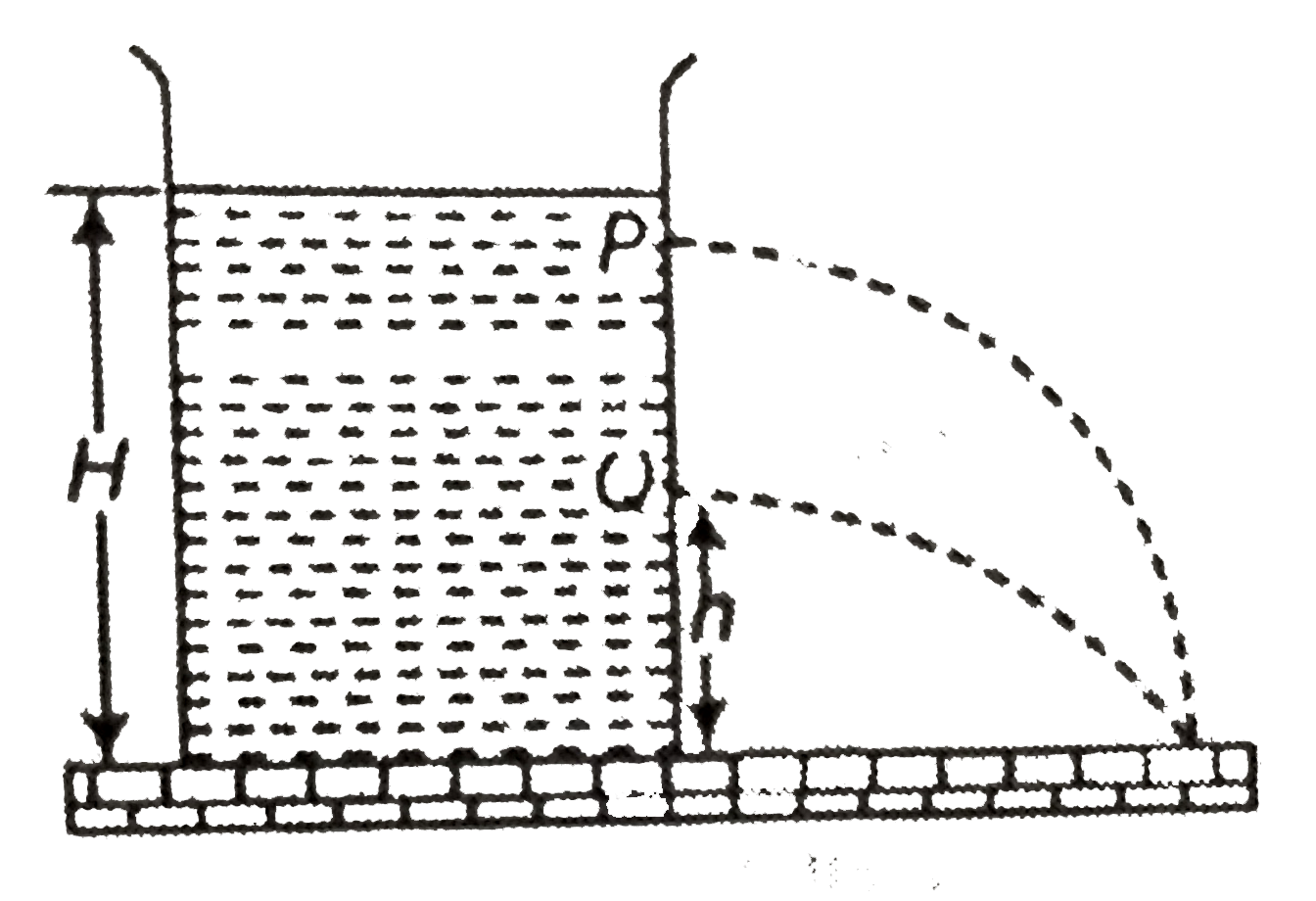
A cylindrical vessel filled with water to a hight H. A vessel has two small holes in the side, from which water iins rushing out horizontal and the two steams stike the ground at the same poin, if the lower hole Q Q is h height above the ground, then the height of hole P above the ground will be

Video Solution

Text Solution

Verified by Experts

Suppose there is a hole in the cylinder at depth y below water level

Velocity of efflux $v = \sqrt{2 g y}$
time taken by water to reach on the plane PQ will be
$t = \sqrt{\frac{2 (\frac{h}{2} + (h - y))}{g}} = \sqrt{\frac{3 h - 2 y}{g}}$
$∴$ horizontal distance x travelled by the liquid is
$x = v t = \sqrt{2 g y} \sqrt{\frac{3 h - 2 y}{g}}$
$x = \sqrt{2 y (3 h - 2 y)}$
for x to be maximum $\frac{d x}{d y} = 0$
$\frac{1}{2 \sqrt{2 y (3 h - 2 y)}} \times 2 (3 h - 4 y) = 0$
$3 h - 4 y = 0, ∴ y = \frac{3 h}{4}$
Hence , x will be maximum at $y = \frac{3 h}{4} = (h - \frac{h}{4})$
where hole number 2 is present.

Updated on:21/07/2023

A cylinderical vessel is filled with water up to height H. A hole is bored in the wall at a depth h from the free surface of water. For maximum range h is equal to
A $H / 4$
B $H / 2$
C $3 H / 4$
D $H$
figure shows two holes in a wide tank containing a liquid common. The water streams coming out of these holes strike the ground at the same point. The heigth of liquid column in the tank is

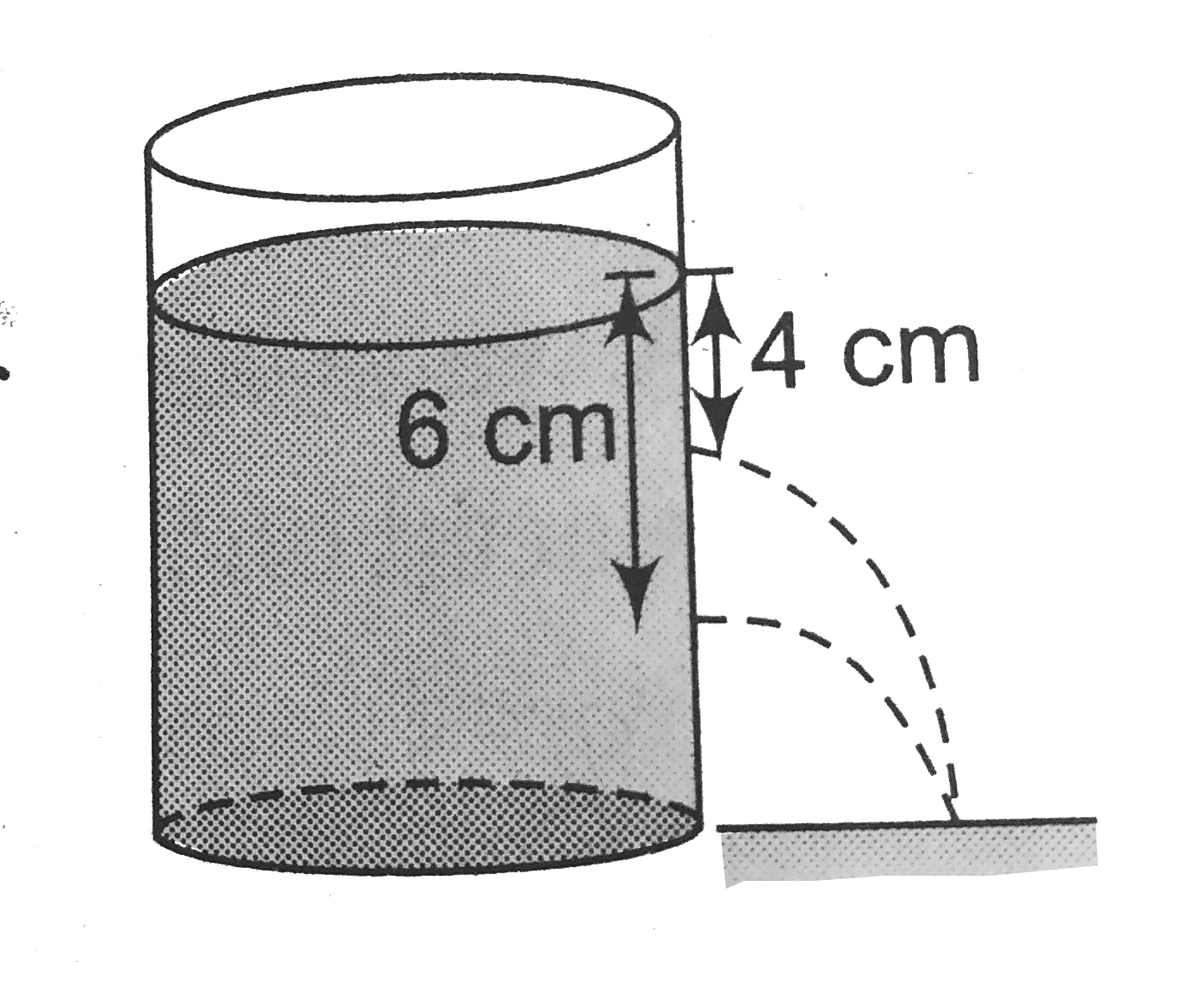A10 cm
B8 cm
C9. 8 cm
D980 cm
A cylindrical vessel is filled with a liquid up to a height H. A small hole is made in the vessel at a distance y below the liquid surface as shown in figure. The liquid emerging from the hole strike the ground at distance x

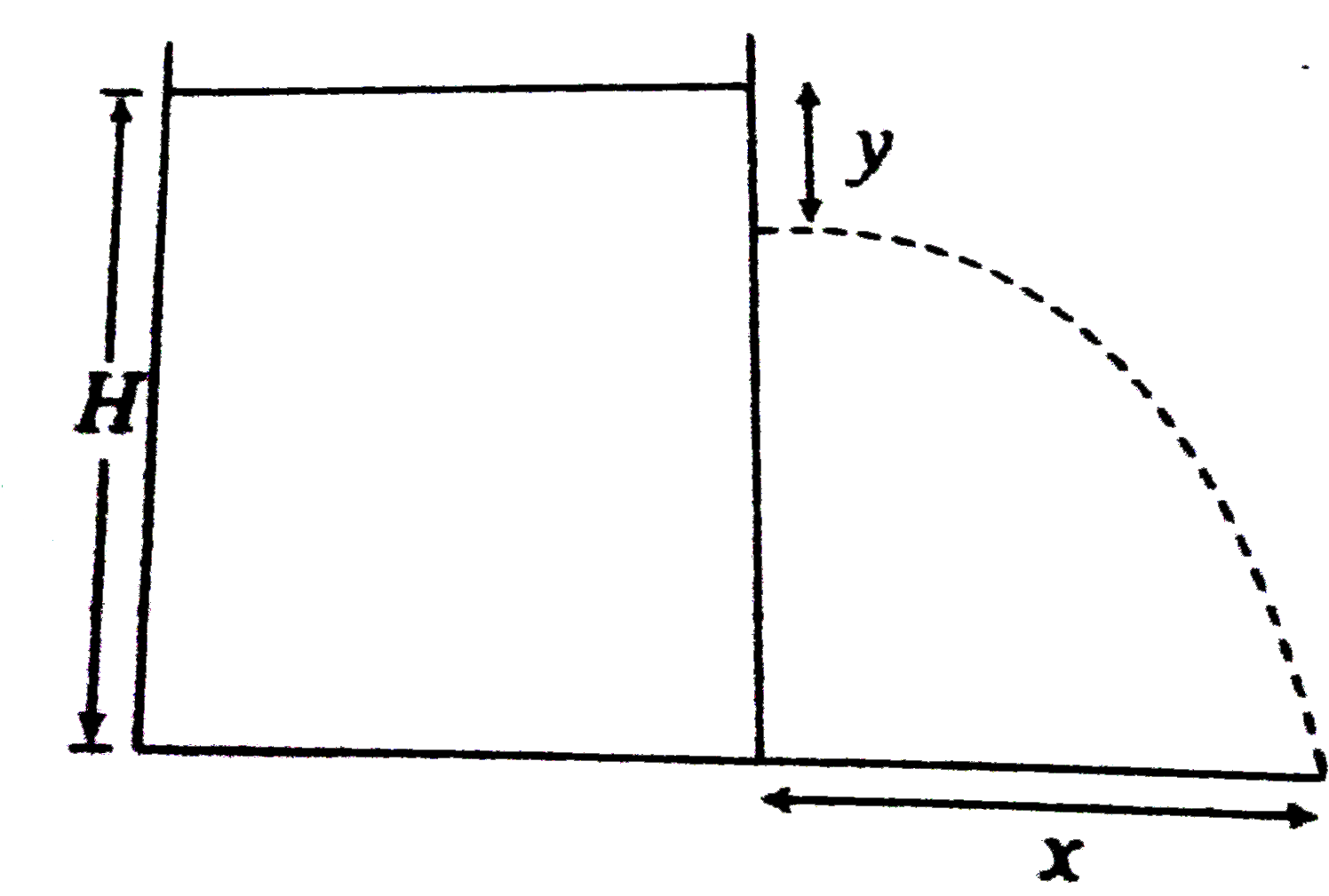Ax is equal if hole is at depth y or H-y
Bx is maximum for y $= H / 2$
CBoth (a ) and (b) are correct
DBoth (d) and (d) are wrong