In the previous question, calculate the time required for the potential difference across capacitor to become equal to that across resistor.
A
13.86s
B
4.86s
C
9.2s
D
21.63s
Text Solution
The correct Answer is:A
Charge as a function of time can be written as: q=q0(1−e−t/τ)⇒q=CV0(1−e−t/RC) On differentiating charge we can write current as a function of time:
i=dqdt=CV0(0+1RCe−t/RC)=V0Re−t/RC
Potential difference across capacitor = Potential difference across resistor
⇒q/C=iR
⇒V0(1−e−t/RC)=V0e−t/RC
⇒e−t/RC=12⇒et/RC=2
⇒tRC=ln2⇒t=RC(ln2)
=(5×106)(4×10−6)(0.693)=13.86s Hence, option (a) is correct
|
Topper's Solved these Questions
- CURRENT ELECTRICITYBOOK - MODERN PUBLICATIONCHAPTER - CURRENT ELECTRICITYEXERCISE - Objective Type Questions (MULTIPLE CHOICE QUESTIONS) (B. MULTIPLE CHOICE QUESTION FOR COMPETITIVE EXAMINATIONS)
20 Videos
- CURRENT ELECTRICITYBOOK - MODERN PUBLICATIONCHAPTER - CURRENT ELECTRICITYEXERCISE - Objective Type Questions (MULTIPLE CHOICE QUESTIONS) (B. MULTIPLE CHOICE QUESTION JEE (MAIN) & OTHER STATE BOARDS FOR ENGINEERING ENTRANCE)
45 Videos
- CURRENT ELECTRICITYBOOK - MODERN PUBLICATIONCHAPTER - CURRENT ELECTRICITYEXERCISE - REVISION EXERCISES (NUMERICAL PROBLEMS)
24 Videos
- ATOMS BOOK - MODERN PUBLICATIONCHAPTER - ATOMS EXERCISE - Chapter Practice Test
16 Videos
- DUAL NATURE OF RADIATION AND MATTER BOOK - MODERN PUBLICATIONCHAPTER - DUAL NATURE OF RADIATION AND MATTER EXERCISE - CHAPTER PRACTICE TEST
15 Videos
Similar Questions
Knowledge Check
In the previous question, the potential difference across A is.
A2εnBε(1−1n)C2ε(1−1n)D(εn−2n)In the given circuit, with steady current, the potential difference across the capacitor is
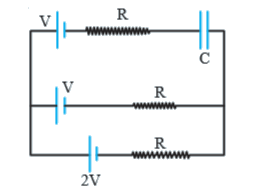 AVBV/2C2V/3DNone of these
AVBV/2C2V/3DNone of theseIn the adjoning figure, the potential difference across the 4.5μF capacitor is
 A4 VB6 VC8 VD4.5 V
A4 VB6 VC8 VD4.5 V
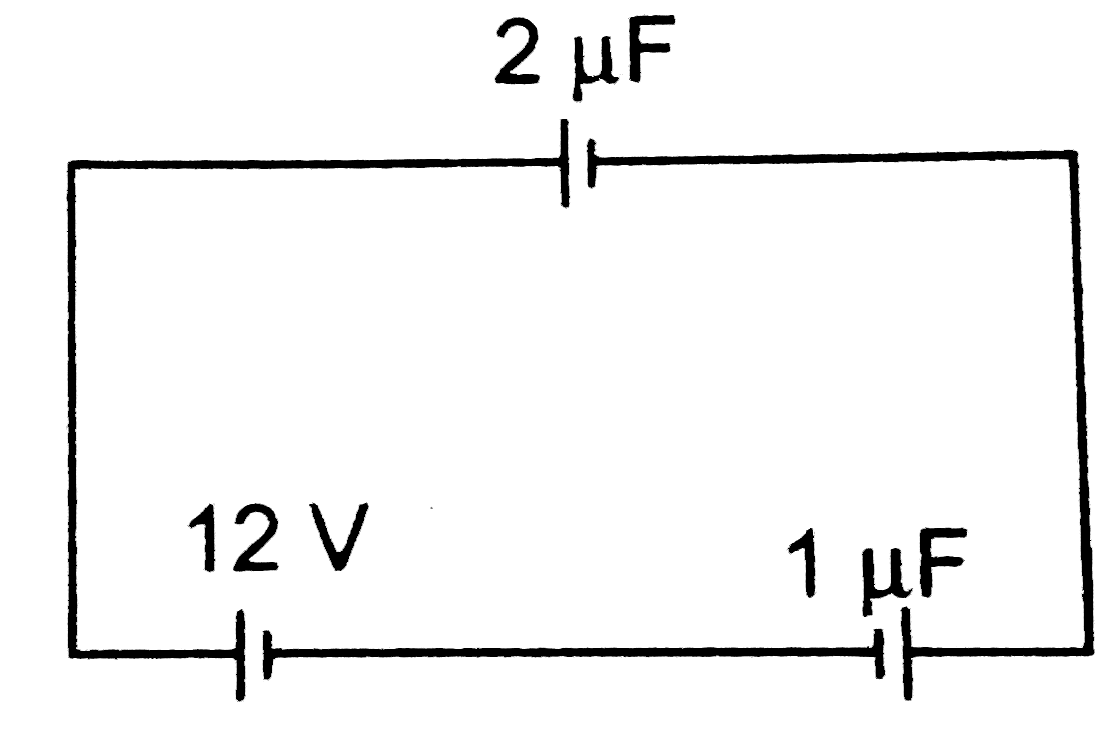
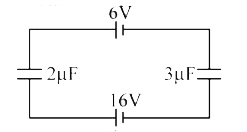
What is the potential difference across 2μF capacitor in the circuit shown?
View SolutionIn the circuit diagram find the potential difference across the plates of capacitor C
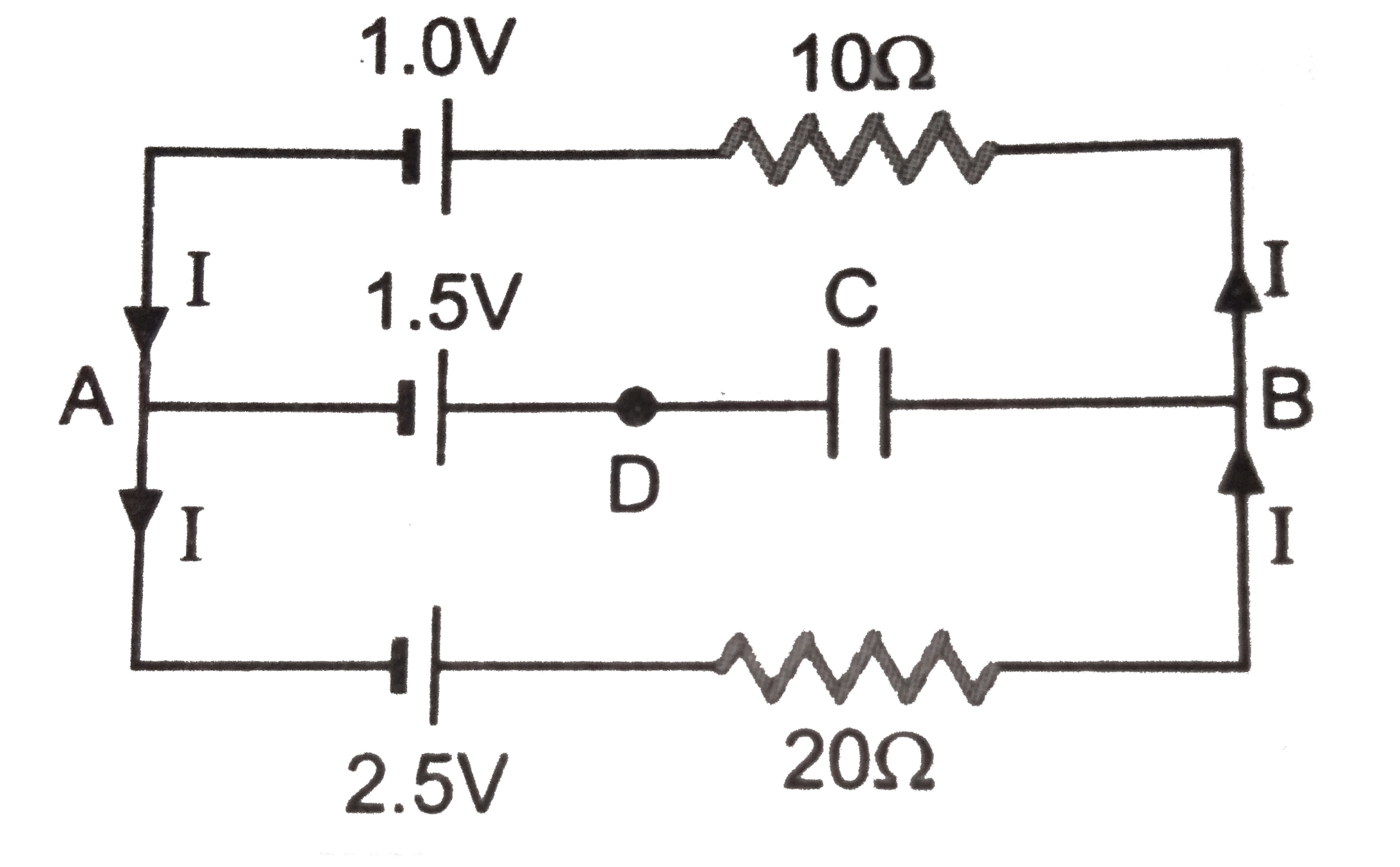
View SolutionIn the adjoning figure, the potential difference across the 4.5μF capacitor is

View SolutionFind charge on each capacitor and potential difference across each capacitor .

View SolutionWhat is the potential difference across resistor in the given circuit ?
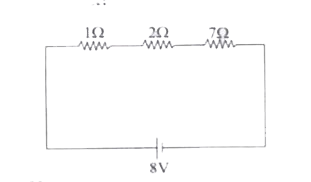
View Solution