For the following frequency distribution, draw a cumulative frequency curve of more than type and hence obtain the median value:

Text Solution
The correct Answer is:Median = 35
|
Topper's Solved these Questions
- POST-MID TEAM TEST PAPERBOOK - VK GLOBAL PUBLICATIONCHAPTER - POST-MID TEAM TEST PAPEREXERCISE - SECTION-C
10 Videos
- POLYNOMIALS BOOK - VK GLOBAL PUBLICATIONCHAPTER - POLYNOMIALS EXERCISE - SELF-ASSESSMENT TEST
11 Videos
- PRE-MID TERM TEST PAPERBOOK - VK GLOBAL PUBLICATIONCHAPTER - PRE-MID TERM TEST PAPEREXERCISE - SECTION-D
3 Videos
Similar Questions
Knowledge Check
In the following frequency distribution, find the median class.

What is the value of median height ?A145.67B157.67C155.83D159.67
Convert the following cumulative frequency distribution into a simple frequency distribution
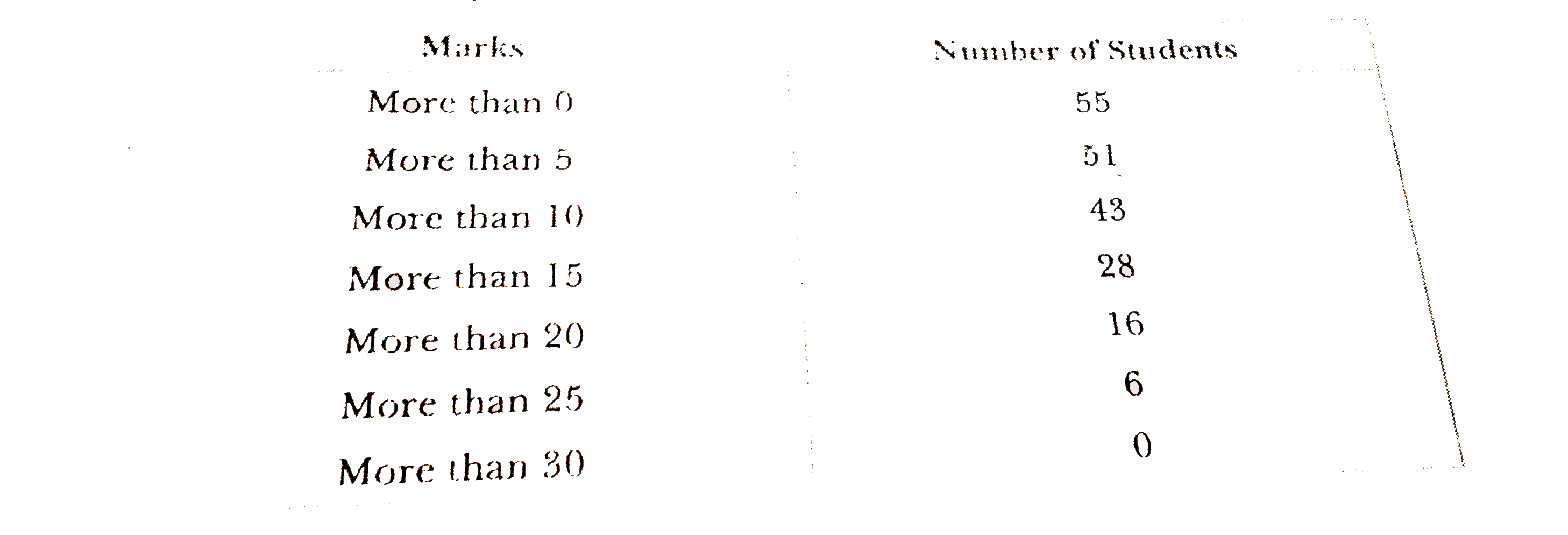
View SolutionConvert the following cumulative frequency distribution into a simple frequency distribution
View SolutionConstruction of Cumulative frequency distribution
View SolutionCumulative Frequency Distribution
View SolutionCumulative Frequency Distribution
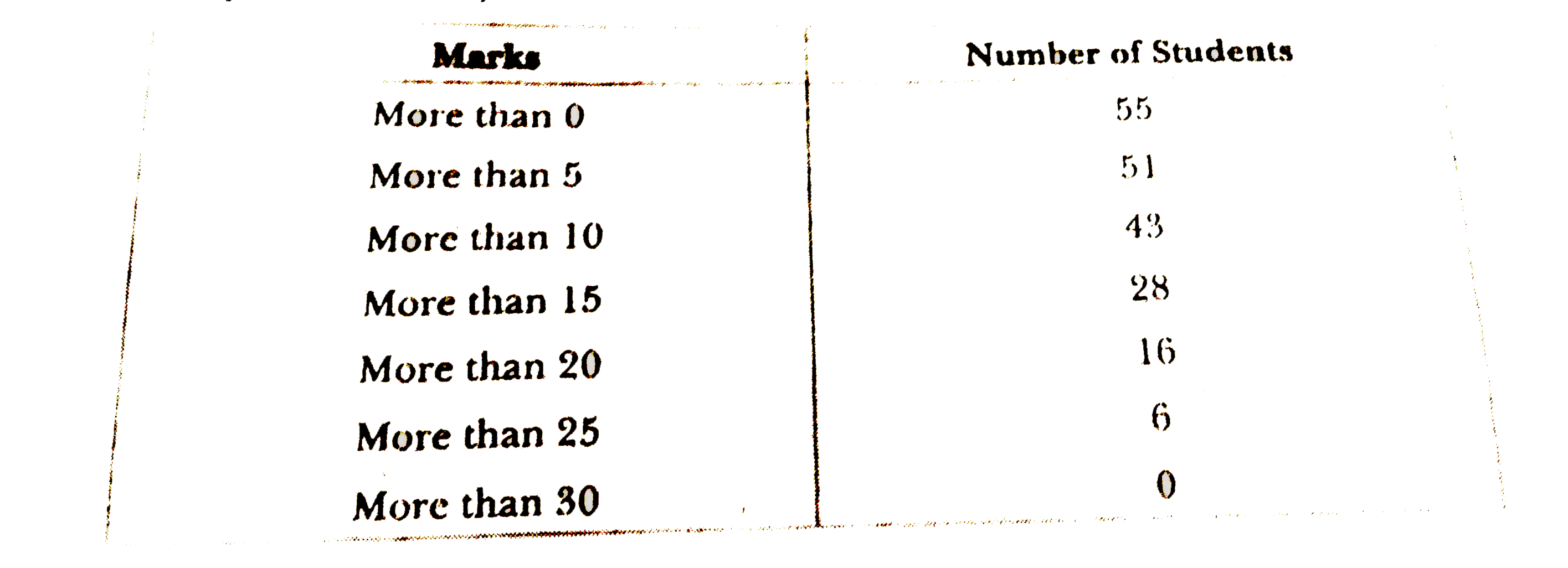
View SolutionFollowing distribution gives cumulative frequencies of 'more than type':
Change the above data to a continuous grouped frequency distribution.
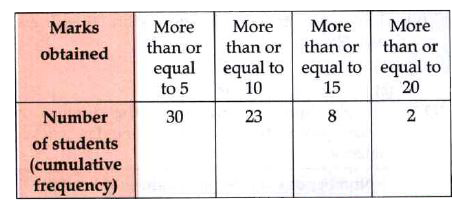
View SolutionMedian OF grouped data || Cumulative frequency distribution table || Different types OF cumulative frequency table less than type and more than or equal to type || Median=L+(n/2-COF/F)h
View Solution
