Let $S = S_{1} \cap S_{2} \cap S_{3}$ , where $s_{1} = {z \in C | z ∣ < 4}, S_{2} = {z \in C : ln [\frac{z - 1 + \sqrt{3} i}{1 - \sqrt{31}}] > 0} and S_{3} = {z \in C : R e z > 0}$

A

0

B

1

C

2

D

$\infty$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:B

Let z=x+iy
Set A corresponds to the region $y \leq 1$
Set B consists of points lying on the circle center at (2,1) and readius 3
i.e $x^{2} + y^{2} y - 4$
Set C consistes of points lying on the $x + y = \sqrt{2}$
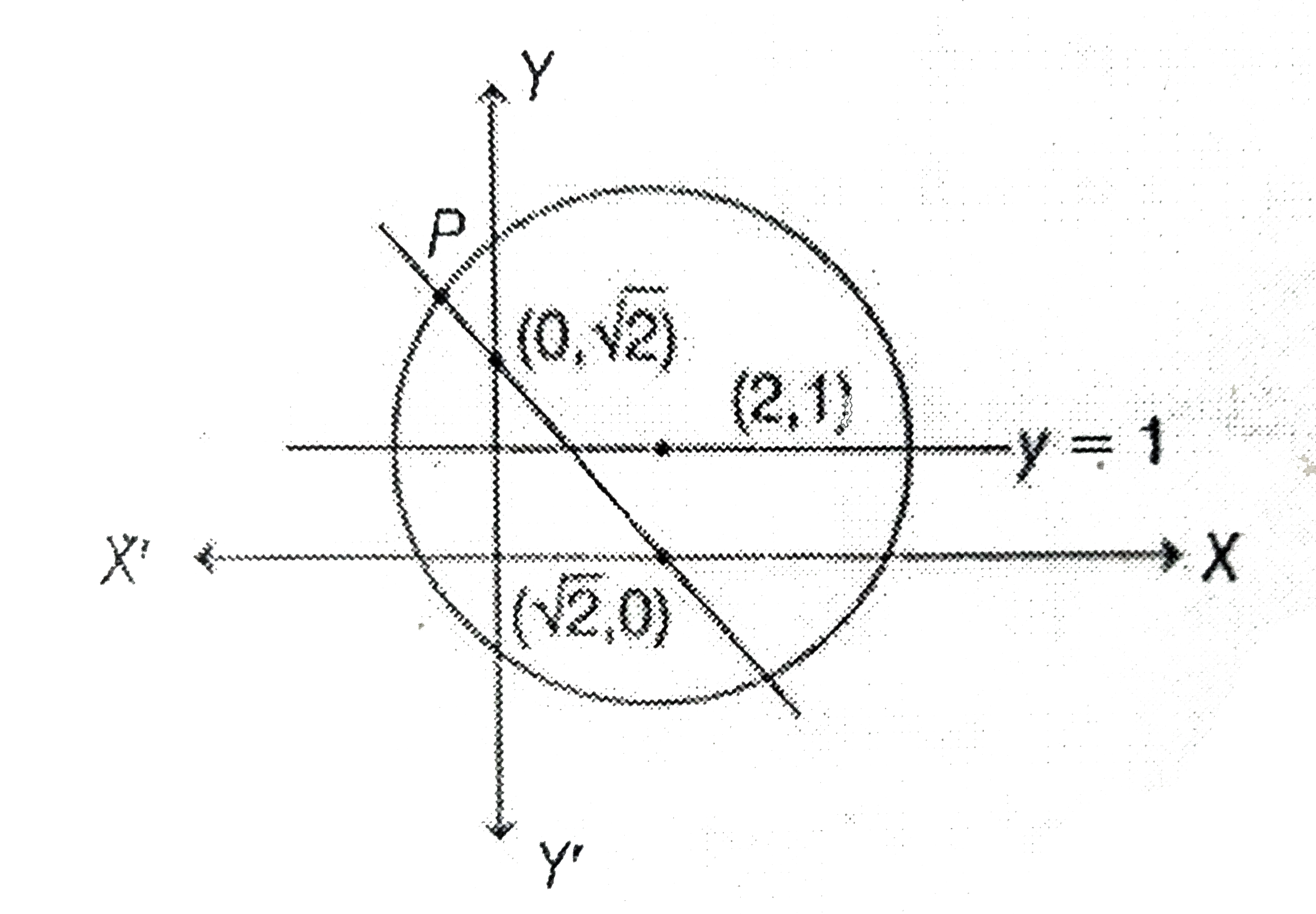Clearly there is only one point of the line $x + y = \sqrt{2}$ and circle $x^{2} + y^{2} - 4 x 2 y = 4$

Updated on:21/07/2023

|

Class 12 MATHS COMPLEX NUMBERS

Topper's Solved these Questions

Similar Questions

Knowledge Check

'Let $S = S_{1} \cap S_{2} \cap S_{3}$ where $S_{1} {z \in C : | z | < 4}, S_{2} = {z \in C : I m [\frac{z - 1 + \sqrt{3} i}{1 - \sqrt{3} i}] > 0}$ and $S_{3} = {z \in C : R e (z) > 0}$ ''
$\frac{min}{z \in C} | 1 - 3 i - z | =$
A $\frac{2 - \sqrt{3}}{2}$
B $\frac{2 + \sqrt{3}}{2}$
C $\frac{3 - \sqrt{3}}{2}$
D $\frac{3 + \sqrt{3}}{2}$
'Let $S = S_{1} \cap S_{2} \cap S_{3}$ where $S_{1} {z \in C : | z | < 4}, S_{2} = {z \in C : I m [\frac{z - 1 + \sqrt{3} i}{1 - \sqrt{3} i}] > 0}$ and $S_{3} = {z \in C : R e (z) > 0}$ ''
Area of S=
A $\frac{10 π}{3}$
B $\frac{20 π}{3}$
C $\frac{16 π}{3}$
D $\frac{32 π}{3}$
Let $S = S_{1} \cap S_{2} \cap S_{3}$ where
$S_{1} = {z \in C | z ∣ < 4}, S_{2} = {z \in C : l m [\frac{z - 1 + \sqrt{3} i}{1 - \sqrt{3} i}] > 0}$ and $S_{3} : {z \in : R e z < 0}$
Let z be any point in $A \cap B \cap C$
The ${| z + 1 - i |}^{2} + {| z - 5 - i |}^{2}$ lies between
A25 and 29
B30 and 34
C35 and 39
D40 and 44

IIT JEE PREVIOUS YEAR-COMPLEX NUMBERS-TOPIC 2 CONJUGATE AND MODULUS OF A COMPLEX NUMBER (PASSAGE BASED PROBLEMS )(PASSAGE II)

Home
Ask Doubt
Profile