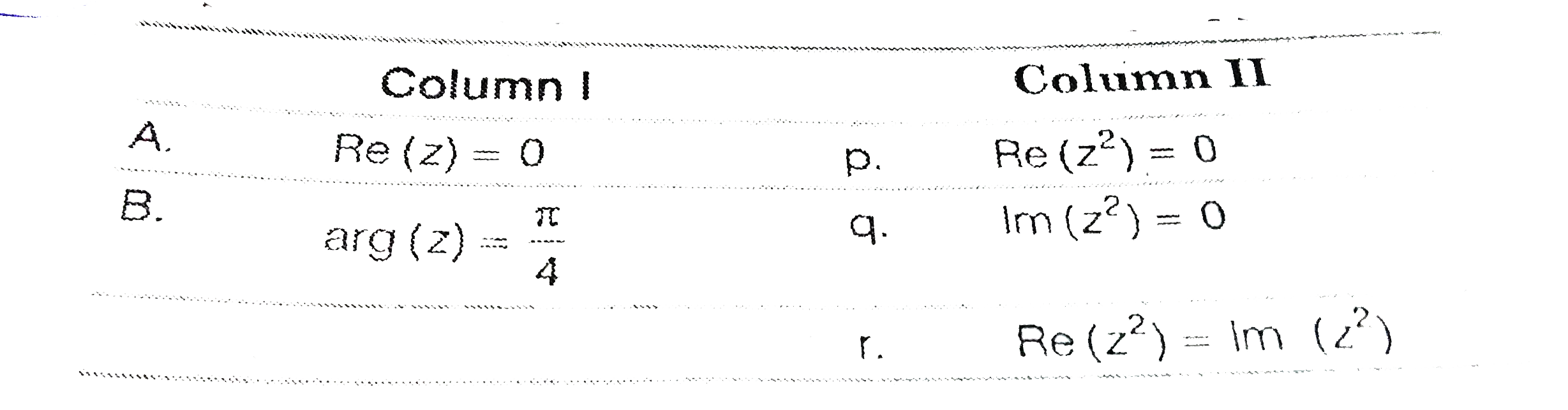
Match the statements of Column I with those of Column II
Here ,z takes values in the complex plane and Im (z) and Re(z) denote respectively , the imaginary part and the real part of z

Text Solution

Verified by Experts

The correct Answer is: $A \to q, r; B \to p; C \to p, s, t; D \to q, r, s t$

A.Let Z=x+iy
$\Rightarrow w e \geq t y \sqrt{x^{+} y^{2}} = 0$
$\Rightarrow y = 0$
$\Rightarrow I_{m} (z) = 0$
B. We have
$\Rightarrow 10_{e} = 8$
$\Rightarrow e = \frac{4}{5}$
$\Rightarrow b^{2} = 25 (1 - \frac{16}{25}) = 9$
$∴ \frac{x^{2}}{25} + \frac{y^{2}}{9} = 1$
C. Let $w = 2 (cos θ + I sin θ)$
$∴ z = 2 (cos θ + i sin θ) - \frac{1}{2 {cos θ}_{i} sin θ}$
$= 2 (cos θ + i sin θ) - \frac{1}{2} (cos θ - sin θ)$
$= \frac{3}{2} cos θ + \frac{5}{2} i sin θ$
Let z=x+iy
$\Rightarrow x = \frac{3}{2} cos θ and y = \frac{5}{2} sin θ$
$\Rightarrow {(\frac{2 x}{3})}^{2} + {(\frac{2 y}{5})}^{2} = 1$
$\Rightarrow \frac{x^{2}}{9 / 4} + \frac{y^{4}}{25 / 4} = 1$
$∴ e = \sqrt{1 - 9 / \frac{4}{25} / 4 = \frac{4}{5}}$
D Let $W = cos θ + i sin θ$
Then $z = x + i y = cos θ + i sin θ + \frac{1}{cos θ + i sin θ}$
$= 2 cos θ$
$\Rightarrow x = 2 cos θ y = 0$

|

Updated on:21/07/2023

Class 12 MATHS COMPLEX NUMBERS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
If z is a complex number such that Re(z)=Im(z), then
A $R e ($ z^2)=0
B $l m ($ z^2)=0
CRe $(z^{2})$ = lm $(z^{2})$
DRe $(z^{2})$ = -lm $(z^{2})$

IIT JEE PREVIOUS YEAR-COMPLEX NUMBERS-TOPIC 2 CONJUGATE AND MODULUS OF A COMPLEX NUMBER (MATCH THE COLUMNS)

Match the statements of Column I with those of Column II Here ,z ta...
Text Solution

Home
Ask Doubt
Profile