If the complex number z1,z2andz3 represent the vertices of an equilateral triangle inscribed in te circel |z|=2andz1=1+i√3 then (A) z2=1,z3=1−i√3 (B) z2=1−i√3,z3=−−i√3 (C) z2=1−i√3,z3=−1+i√3 (D) z2=,z3=1−i√3
The correct Answer is:z2=−2,z3=1−i√3
z1=1+i√3=r(cosθ+isinθ) [let]
⇒rcosθ=1,rsinθ=√3
⇒ r=2andθ=√3
Since |z2|=|z3|=2 [given]
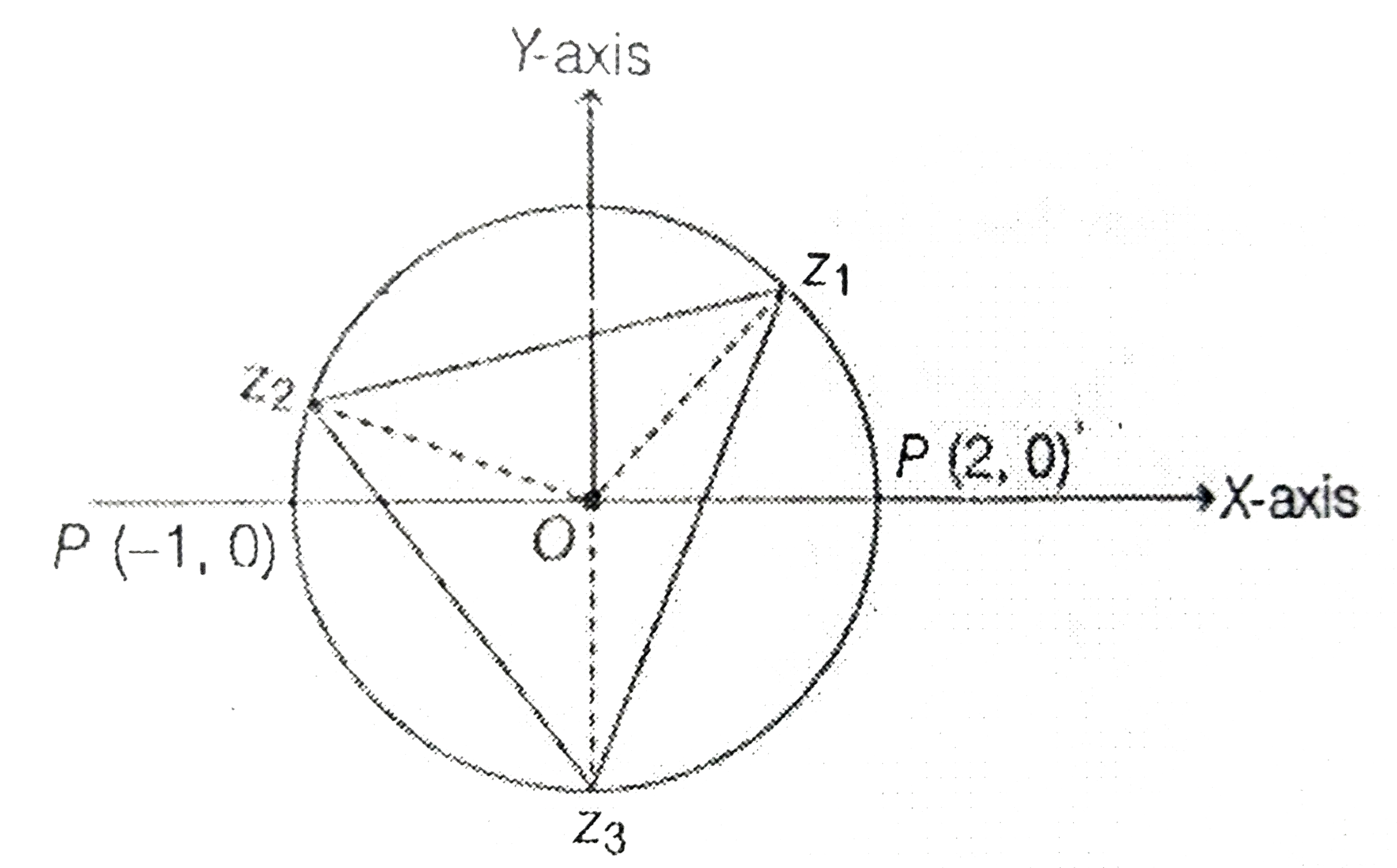
Now the triangle z1,z2andz3 being an equilateral and the sides z1z2and z1z3 make an angle 2π/3 at the centre.
Therefore ∠POz2=π3+2π3=π
and ∠POz3=π3+2π3+2π3=5π3
Therefore , z2=2(cosπ+isinπ)=2(−1+0)=−2
andz3=2(cos5π3+isin5π3)=2(12−i√32)=1−i√3
Alternate Solution
Wherenever vertices of an equilateral triangle having centroid is given its vertices are of the form z.zω,zω2
∴ If one of the vertex is z1=1+i√3 then other two vertices are (z1ω),(z1ω2)
⇒(1+i√3)(−1+i√3)2,(1+i√3)(−1−i√3)2
⇒−(1+3)2,−(1+i2(√3)2+2i√3)2
⇒−2,−(−2+2i√3)2=1−i√3
∴z2=−2 and z3=1−i√3
Topper's Solved these Questions
- BOOK - IIT JEE PREVIOUS YEARCHAPTER - COMPLEX NUMBERSEXERCISE - TOPIC 4 ROTATION OF A COMPLEX NUMBER (ANALYTICAL & DESCRIPTIVE QUESTIONS)7videos
COMPLEX NUMBERS
- BOOK - IIT JEE PREVIOUS YEARCHAPTER - COMPLEX NUMBERSEXERCISE - TOPIC 4 ROTATION OF A COMPLEX NUMBER (INTERGER ANSWER TYPE QUESTION )1videos
COMPLEX NUMBERS
- BOOK - IIT JEE PREVIOUS YEARCHAPTER - COMPLEX NUMBERSEXERCISE - TOPIC 4 ROTATION OF A COMPLEX NUMBER (OBJECTIVE QUESTION II)(ONE OR MORE THAN ONE CORRECT OPTION)2videos
COMPLEX NUMBERS
- BOOK - IIT JEE PREVIOUS YEARCHAPTER - CIRCLEEXERCISE - Topic 5 Integer Answer type Question1videos
CIRCLE
- BOOK - IIT JEE PREVIOUS YEARCHAPTER - DEFINITE INTEGRATIONEXERCISE - LIMITS AS THE SUM6videos
DEFINITE INTEGRATION
Similar Questions
If the complex number z1,z2andz3 represent the vertices of an equilateral triangle inscribed in the circle |z|=2andz1=1+i√3 then (A) z2=1,z3=1−i√3 (B) z2=1−i√3,z3=−i√3 (C) z2=1−i√3,z3=−1+i√3 (D) z2=,z3=1−i√3
View Solutionlf z1,z2,z3 are vertices of an equilateral triangle inscribed in the circle |z|=2 and if z1=1+ι√3 , then
View Solution
Knowledge Check
- Question 1 - Select One
If z1,z2andz3 represent the vertices of an equilateral triangle such that
Az1+z2=z3Bz1+z2+z3=0Cz1z2=1z3Dz1−z2=z3−z2 - Question 2 - Select One
If z1,z2andz3 represent the vertices of an equilateral triangle such that |z1|=|z2|=|z3|, then
Az1+z2=z3Bz1+z2+z3=0Cz1z2=1z3Dz1−z2=z3−z2 - Question 3 - Select One
If z1,z2,z3 are the vertices of an equilateral triangle inscribed in the circle |z|=1 then the area of the triangle having z1,z2,z3 as its vertices is
A√32B√34C3√34DNone of these
If the complex numbers z1,z2 and z3 represent the vertices of an equilateral triangle such that |z1|=|z2|=|z3|, then z1+z2+z3=0
View Solutionif the complex no z1,z2andz3 represents the vertices of an equilateral triangle such that |z1|=|z2|=|z3| then relation among z1,z2andz3
View Solutionlf z1,z2,z3 are vertices of an equilateral triangle inscribed in the circle |z|=2 and if z1=1+ι√3 , then
View Solutionif the complex no z1,z2andz3 represents the vertices of an equilateral triangle such that |z1|=|z2|=|z3| then relation among z1,z2andz3
View SolutionIf the points z1,z2 and z3 are the vertices of an equilateral triangle in the complex plane, then the value of z21+z22+z23 is equal to
View Solution

