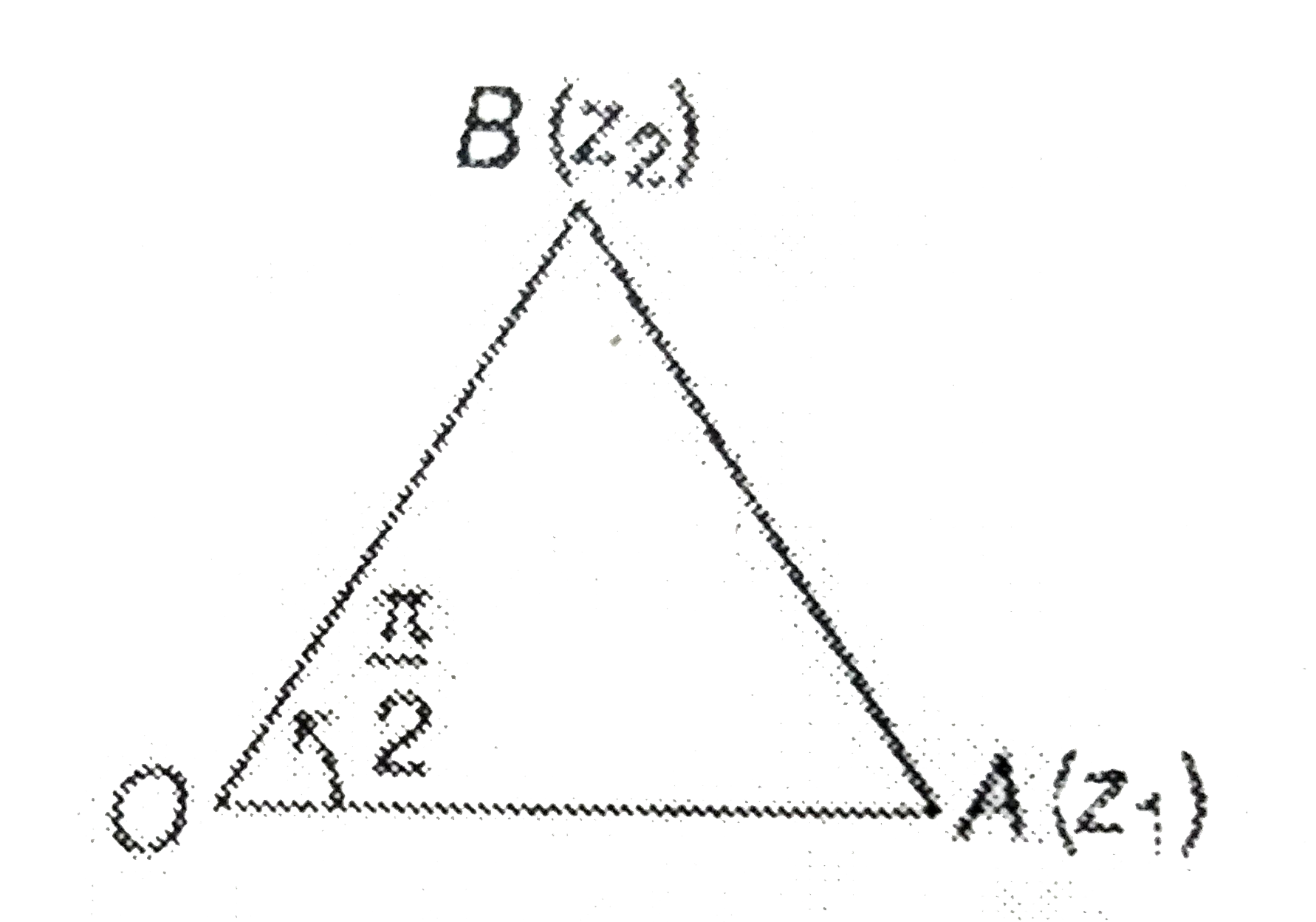
Q. Let $z_{1}$ and $z_{2}$ be nth roots of unity which subtend a right angle at the origin, then n must be the form $4 k$ .

A

$4 k + 1$

B

$4 k + 2$

C

$4 k + 3$

D

4k

Video Solution

Text Solution

Verified by Experts

The correct Answer is:D

Since arg $\frac{z_{1}}{z_{2}} = \frac{π}{2}$
$\Rightarrow \frac{z_{1}}{z_{2}} = cos l \frac{π}{2} + I sin \frac{π}{2} = i$
$∴ \frac{z_{1}}{z_{2}} = cos \frac{π}{2} + I sin \frac{π}{2} = i$
$∴ z_{1}^{n} = {(i)}^{n} \Rightarrow i^{n} = 1 [∵ | z_{2} | = | z_{1} | = 1]$
$\Rightarrow n = 4 k$
Alternate Solution
Since arg $\frac{z_{2}}{z_{1}} = \frac{π}{2}$
$∴ \frac{z_{2}}{z_{1}} = ∣ ∣ ∣ \frac{z_{2}}{z_{1}} ∣ ∣ ∣ e^{i \frac{π}{2}}$
$\Rightarrow \frac{z_{2}}{z_{1}} = i [∵ | z_{1} | = | z_{2} | = 1]$
$\Rightarrow {(\frac{z_{2}}{z_{1}})}^{n} = i^{n}$
$∴ z_{1} and z_{2}$ are nth roots of unity
$\Rightarrow {(\frac{z_{2}}{z_{1}})}^{z_{1}^{n} = z_{2}^{n} = 1}$
$\Rightarrow i^{n} = 1$
$\Rightarrow n = 4 k$ where k is an integer.

|

Updated on:21/07/2023

Class 12 MATHS COMPLEX NUMBERS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
Let $z_{1} and z_{2}$ be nth roots of unity which subtend a right angle at the origin, then n must be of the form
A $4 k + 1$
B $4 k + 2$
C $4 k + 3$
D $4 k$
Question 2 - Select One
Q. Let $z_{1}$ and $z_{2}$ be nth roots of unity which subtend a right angle at the origin, then n must be the form $4 k$ .
A4 k - 1
B4 k + 2
C4 k + 3
D4 k
Question 3 - Select One
If $z_{1}$ and $z_{2}$ are two $n^{t h}$ roots of unity, then arg $(\frac{z_{1}}{z_{2}})$ is a multiple of
A $n π$
B $\frac{3 π}{n}$
C $\frac{2 π}{n}$
Dnone of these

IIT JEE PREVIOUS YEAR-COMPLEX NUMBERS-TOPIC 5 DE-MOIVRES THEOREM,CUBE ROOTS AND nth ROOTS OF UNITY ( OBJECTIVE QUESTIONS I)(ONLY ONE CORRECT OPTION)

Home
Ask Doubt
Profile