ABCD is a square with side AB = 2. A point P moves such that its distance from A equals its distance from the line BD. The locus of P meets the line AC at $T_{1}$ and the line through A parallel to BD at $T_{2}$ and $T_{3}$ . The area of the triangle $T_{1} T_{2} T_{3}$ is :

A

$\frac{1}{2}$ sq unit

B

$\frac{2}{3}$ sq unit

C

1 sq unit

D

2sq units

Video Solution

Text Solution

Verified by Experts

The correct Answer is:C

Since, $A G = \sqrt{2}$
$A T_{1} = T_{1} G = \frac{1}{\sqrt{2}}$
As, A is the focus, $T_{1}$ is the vertex and BD I s the directrix of parabola.

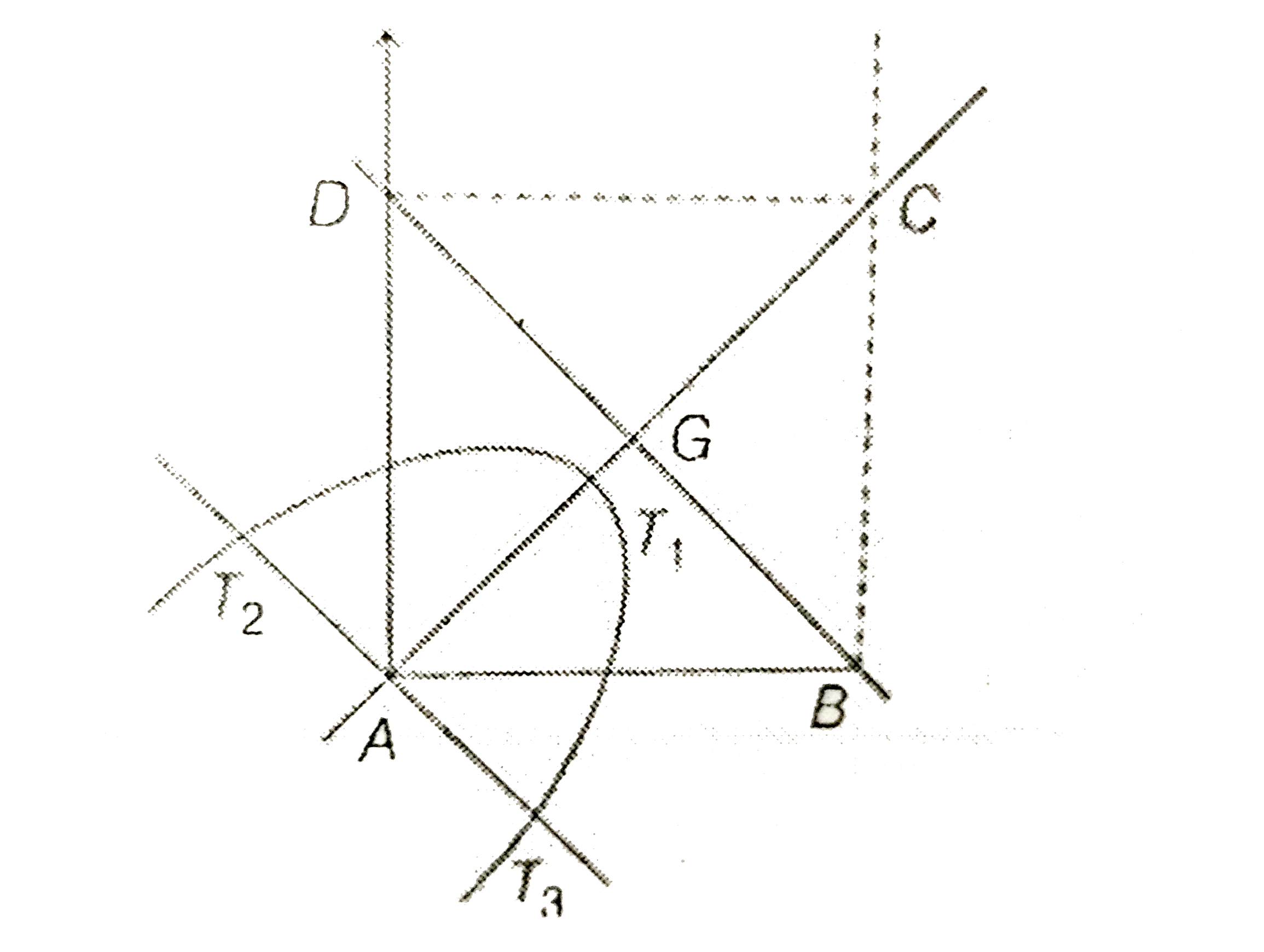
Also, $T_{2} T_{3}$ is latusrectum.
$∴ T_{2} T_{3} = 4 . \frac{1}{\sqrt{2}}$
$∴ Area of Δ T_{1} T_{2} T_{3} - \frac{1}{2} \times \frac{1}{\sqrt{2}} \times \frac{4}{\sqrt{2}} = 1$ sq unit

|

Updated on:21/07/2023

Class 12 MATHS CIRCLE

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
A point P moves such that its distances from (1 , 2) and (-2 , 3) are equal . Then the locus of P is
AStraight line
BParabola
CEllipse
DHyperbola
Question 2 - Select One
A point P moves such that its distances from (1 , 2) and (-2 , 3) are equal . Then the locus of P is
AStraight line
BParabola
CEllipse
DHyperbola
Question 3 - Select One
ABCD is a square of side length 2. $C_{1}$ is a circle inscribed in the square and $C_{2}$ is a circle. circumscribing the square. P and Q are any two points on $C_{1} and C_{2}$ respectively. Also R is fixed point and L is a fixed line in the same plane. A circle C touches line L and circle $C_{1}$ externally. S is a point which is equidistant from given point Rand fixed line L. Point R coincides with B.
Line Lpasses through A and Cand a line parallel to AC passes through B. If the locus of S cuts this line at 2 points $T_{1}$ and $T_{2}$ and the diagonal BD at $T_{3}$ , the area of triangle $T_{1} T_{2} T_{3}$ is :
A1 sq. unit
B $\frac{1}{2}$ sq. unit
C2 sq. units
D3 sq. units

IIT JEE PREVIOUS YEAR-CIRCLE-Topic 2 Passage Based Problems

Home
Ask Doubt
Profile