AD is a median of the ΔABC . If AE and AF are medians of the triangles ABD and ADC repectively , and AD=m1,AE=m2,AF=m3, then m22+m23−2m21=
a2
a22
a24
a28
The correct Answer is:d
Topper's Solved these Questions
- SOLUTION OF TRIANGLESBOOK - MTG-WBJEECHAPTER - SOLUTION OF TRIANGLESEXERCISE - WB JEE WORKOUT (CATEGORY 3 : One or More than One Option Correct Type
10 Videos
- SOLUTION OF TRIANGLESBOOK - MTG-WBJEECHAPTER - SOLUTION OF TRIANGLESEXERCISE - WB JEE (Previous Years Questions) CATEGORY 1 : Single Option Correct Type
5 Videos
- SOLUTION OF TRIANGLESBOOK - MTG-WBJEECHAPTER - SOLUTION OF TRIANGLESEXERCISE - WB JEE (Previous Years Questions) CATEGORY 2: Single Option Correct Type
1 Video
- SETS , RELATIONS AND FUNCTIONSBOOK - MTG-WBJEECHAPTER - SETS , RELATIONS AND FUNCTIONSEXERCISE - WB JEE PREVIOUS YEARS QUESTIONS (SINGLE OPTION CORRECT TYPE)
44 Videos
- STATISTICS AND PROBABILITYBOOK - MTG-WBJEECHAPTER - STATISTICS AND PROBABILITYEXERCISE - WB JEE Previous Years Questions ( CATEGORY 3 : One or More than One Option Correct Type ( 2 Marks) )
3 Videos
Similar Questions
Let AD be a median of the ΔABC. If AE and AF are medians of the triangle ABD and ADC, respectively, and BD=a/2 AD = m1,AE=m2,AF=m3, then a2/8 is equal to
View SolutionAD is a median of the ΔABC. If AE are medians of the ΔABDandΔADC respectively, and AD=m1,AE=m2,AF=m3, then find the value of a28.
View Solution
Knowledge Check
Let AD be a median of the ΔABC. If AE and AF are medians of the triangle ABD and ADC, respectively, and AD = m1,AE=m2,AF=m3, then a2/8 is equal to
Am22+m23−2m21Bm21+m22−2m23Cm21+m23−2m22Dnone of theseIn triangle ABC m1,m2,m3 are the lenghts of the medians through A,B and C respectively . If C=π2, then m21+m22m23=
A2B3C4D5
Let AD be a median of the ABC. If AEandAF are medians of the triangle ABDandADC , respectively, and AD=m1,AE=m2,AF=m3, thena28 is equal to (a)m22+m23−2m21 (b) m21+m22−2m23 (c)m21+m23−2m22 (d) none of these
View SolutionLet AD be a median of the ABC. If AEandAF are medians of the triangle ABDandADC , respectively, and AD=m1,AE=m2,AF=m3, thena28 is equal to (a)m22+m23−2m21 (b) m21+m22−2m23 (c)m21+m23−2m22 (d) none of these
View SolutionIn ΔABC, E is the midpoint of the median AD. BE produced meets AC at F. Prove that AF=(13)AC
View SolutionIn a triangle ABC, having AD is median and area of triangle ABD= 120m2. Find area of triangle ABC.
View SolutionThe slope of graph as shown in figure at points 1,2 and 3 is m1,m2 and m3 respectively then
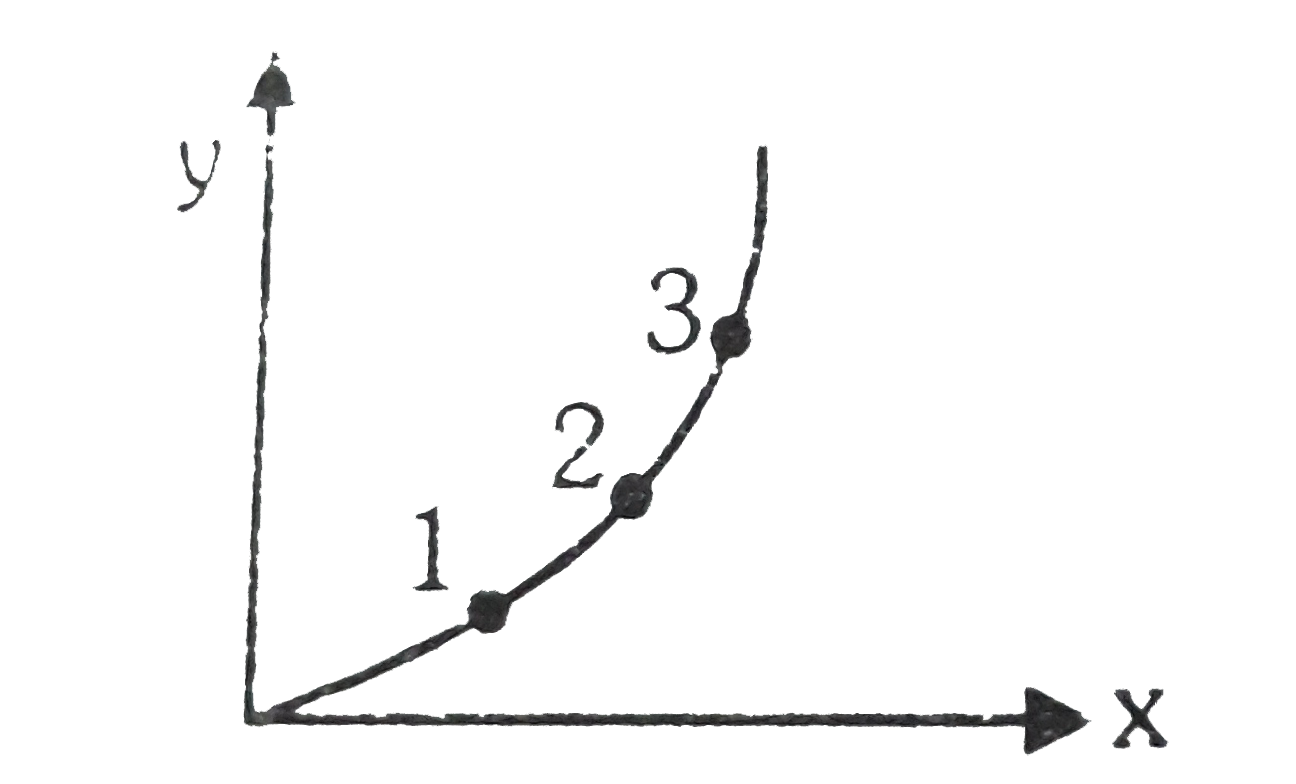
View SolutionThe slope of graph as shown in figure at points 1,2 and 3 is m1,m2 and m3 respectively then

View Solution
