I is the incentre of ΔABCandP1,P2andP3 respectively are the radii of the circumcircles of the ΔIBC,ΔICAandΔIAB . Then P1P2P3=
Rr2
2R2r
2Rr
R2r
The correct Answer is:b
Topper's Solved these Questions
- SOLUTION OF TRIANGLESBOOK - MTG-WBJEECHAPTER - SOLUTION OF TRIANGLESEXERCISE - WB JEE WORKOUT (CATEGORY 3 : One or More than One Option Correct Type
10 Videos
- SOLUTION OF TRIANGLESBOOK - MTG-WBJEECHAPTER - SOLUTION OF TRIANGLESEXERCISE - WB JEE (Previous Years Questions) CATEGORY 1 : Single Option Correct Type
5 Videos
- SOLUTION OF TRIANGLESBOOK - MTG-WBJEECHAPTER - SOLUTION OF TRIANGLESEXERCISE - WB JEE (Previous Years Questions) CATEGORY 2: Single Option Correct Type
1 Videos
- SETS , RELATIONS AND FUNCTIONSBOOK - MTG-WBJEECHAPTER - SETS , RELATIONS AND FUNCTIONSEXERCISE - WB JEE PREVIOUS YEARS QUESTIONS (SINGLE OPTION CORRECT TYPE)
44 Videos
- STATISTICS AND PROBABILITYBOOK - MTG-WBJEECHAPTER - STATISTICS AND PROBABILITYEXERCISE - WB JEE Previous Years Questions ( CATEGORY 3 : One or More than One Option Correct Type ( 2 Marks) )
3 Videos
Similar Questions
If I is the incenter of ΔABCandR1,R2,andR3 are, respectively, the radii of the circumcircle of the triangle IBC, ICA, and IAB, then prove that R1R2R3=2rR2
View SolutionIn ΔABC, I is the incentre, Area of ΔIBC,ΔIACandΔIAB are, respectively, Δ1,Δ2andΔ3. If the values of Δ1,Δ2andΔ3 are in A.P., then the altitudes of the ΔABC are in
View Solution
Knowledge Check
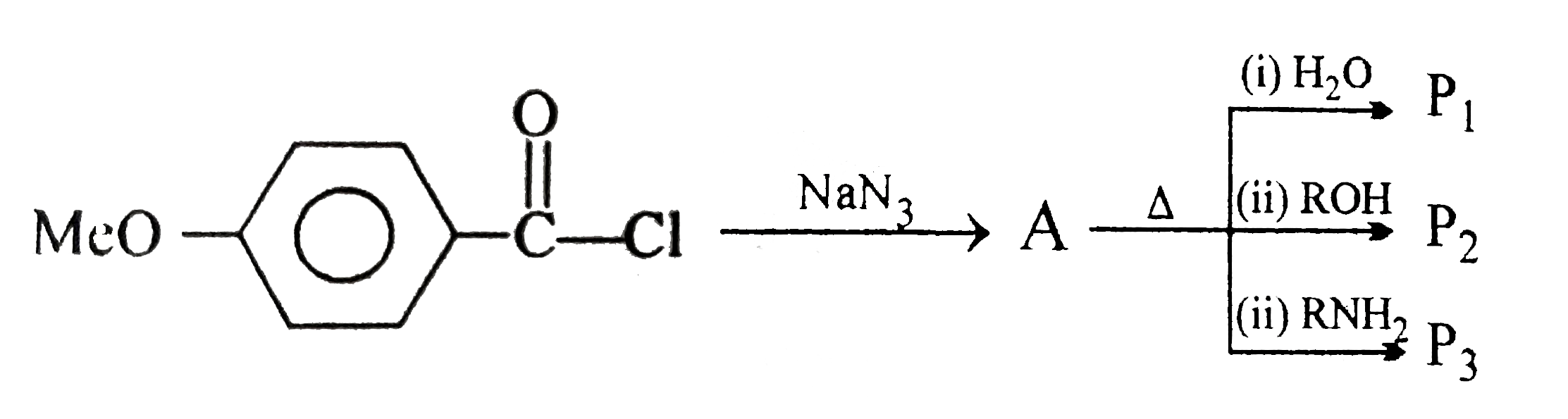
P1,P2,P3,are:A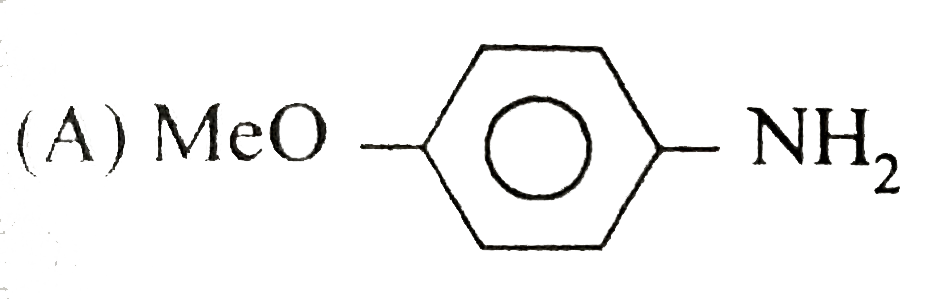 B
B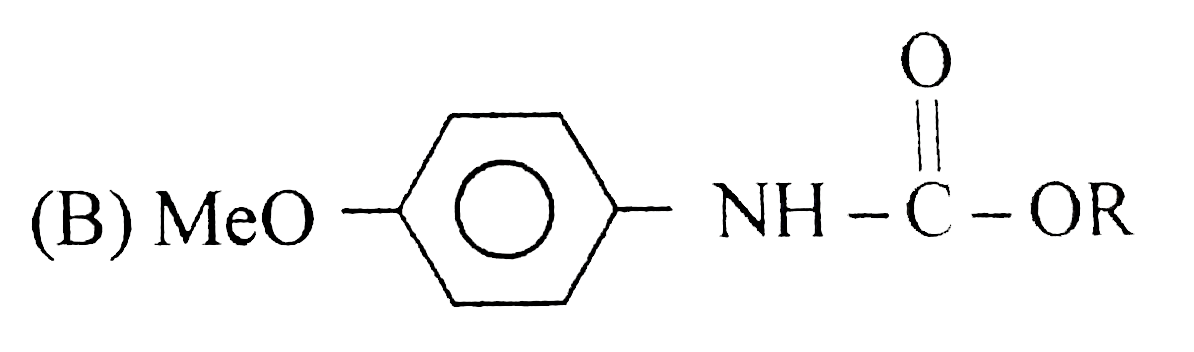 C
C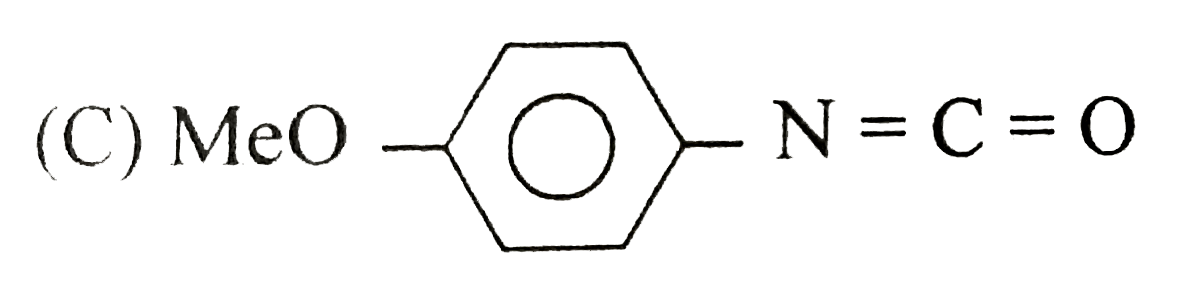 D
D
In ΔABC, I is the incentre, Area of ΔIBC,ΔIACandΔIAB are, respectively, Δ1,Δ2andΔ3. If the values of Δ1,Δ2andΔ3 are in A.P., then the altitudes of the ΔABC are in
AA.P.BG.P.CH.P.Dnone of theseConsider three planes P1:x−y+z=1
P2:x+y−z=−1
and P3:x−3y+3z=2
Let L1,L2,L3 be the lines of intersection of the planes P2 and P3,P3 and P1,P1 and P2 respectively.
Statement I Atleast two of the lines L1,L2 and L3 are non-parallel.
Statement II The three planes do not have a common point.AStatement I is true, Statement II is also true,
Statement II is the correct explanation of
Statement IBStatement I is true, Statement II is also true,
Statement II is not the correct explanation of
Statement ICStatement I is true, Statement II is falseDStatement I is false, Statement II is true
Consider three planes P1:x−y+z=1, P2:x+y−z=−1 and
P3:x−3y+3z=2. Let L1,L2,L3 be the lines of intersection of the planes P2 and P3,P3 and P1,P1 and P2 respectively.
Statement I Atleast two of the lines L1,L2 and L3 are non-parallel.
Statement II The three planes do not have a common point.
View SolutionIn a triangle ABC,r,r1,r2,r3, are the radii of in circle and ex circles and P1,P2,P3, are altitudes of triangle ABC.Then 1P1+1P2+1P3 is equal to
View SolutionIf p1,p2,p3 are respectively the perpendicular from the vertices of a triangle to the opposite sides, then find the value of p1p2p3.
View SolutionIf p1,p2,p3 are respectively the perpendicular from the vertices of a triangle to the opposite sides, then find the value of p1p2p3.
View SolutionIf P1,P2 and P3 are the altitudes of a triangle from vertices A,B and C respectively and Δ is the area of the triangle, then the value of 1P1+1P2−1P3=
View Solution
