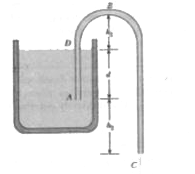
Figure shows a siphon , which is a device for removing liquid from a container . Tube ABC must initially be filled , but once this has been done , liquid will flow through the tube until the liquid surface in the container is level with the tube opening at A . The liquid has density 1000kg/m3 and negligible viscosity . The distances shown are h1=25 cm d = 12 cm and h2=40 cm
If the atmospheric pressure is 1.0×105Pa, what is the pressure in the liquid at the topmost point B ?
Since vB=vC by equation of continuity , and pC=pair , Bernouli.s equation becomes
pB=pC+ρg(hC−hB)=pair−ρg(h1+h2+d)
=10×105Pa−(1.0×103kg/m3)(9.8m/s2)×(0.25m+0.40m+0.12m)
=9.2×104 Pa
Topper's Solved these Questions
- FLUIDSBOOK - RESNICK AND HALLIDAYCHAPTER - FLUIDSEXERCISE - SAMPLE PROBLEM 14.23
1 Videos
- FLUIDSBOOK - RESNICK AND HALLIDAYCHAPTER - FLUIDSEXERCISE - SAMPLE PROBLEM 14.24
1 Videos
- FLUIDSBOOK - RESNICK AND HALLIDAYCHAPTER - FLUIDSEXERCISE - SAMPLE PROBLEM 14.21
1 Videos
- ELECTROMAGNETIC WAVES BOOK - RESNICK AND HALLIDAYCHAPTER - ELECTROMAGNETIC WAVES EXERCISE - PRACTICE QUESTIONS (Integer Type)
3 Videos
- FORCE AND MOTION - I BOOK - RESNICK AND HALLIDAYCHAPTER - FORCE AND MOTION - I EXERCISE - PRACTICE QUESTIONS (Integer Type)
3 Videos
Similar Questions
Figure shows a siphon , which is a device for removing liquid from a container . Tube ABC must initially be filled , but once this has been done , liquid will flow through the tube until the liquid surface in the container is level with the tube opening at A . The liquid has density 1000kg/m3 and negligible viscosity . The distances shown are h1=25 cm d = 12 cm and h2=40 cm
(a) With what speed does the liquid emerge from the tube at C ?
View SolutionFigure shows a siphon , which is a device for removing liquid from a container . Tube ABC must initially be filled , but once this has been done , liquid will flow through the tube until the liquid surface in the container is level with the tube opening at A . The liquid has density 1000kg/m3 and negligible viscosity . The distances shown are h1=25 cm d = 12 cm and h2=40 cm
Theoretically , what is the greatest possible height h1 that a siphon can lift water ?
View Solution
Knowledge Check
The velocity of liquid flowing through a tube at certain distance from the axis of tube
Aincreases with distance.Bremains constant.Cdecreases with distance.Ddepends upon length of the tube.A container filled with a liquid A is floating on the surface of another liquid 'B' as shown in the figure, then volume of the liquid displaced by the container
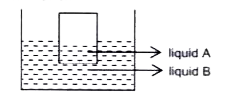 Ais equal to the volume of the liquid A.Bis greater than the volume of the liquid A.Cis less than the volume of the liquid A.DCannot be determined
Ais equal to the volume of the liquid A.Bis greater than the volume of the liquid A.Cis less than the volume of the liquid A.DCannot be determinedA container filled with a liquid A is floating on the surface of another liquid 'B' as shown in the figure, then volume of the liquid displaced by the container
 Ais equal to the weight of the liquid A.Bis greater than the weight of the liquid A.Cis less than the weight of the liquid ADCannot be determined
Ais equal to the weight of the liquid A.Bis greater than the weight of the liquid A.Cis less than the weight of the liquid ADCannot be determined
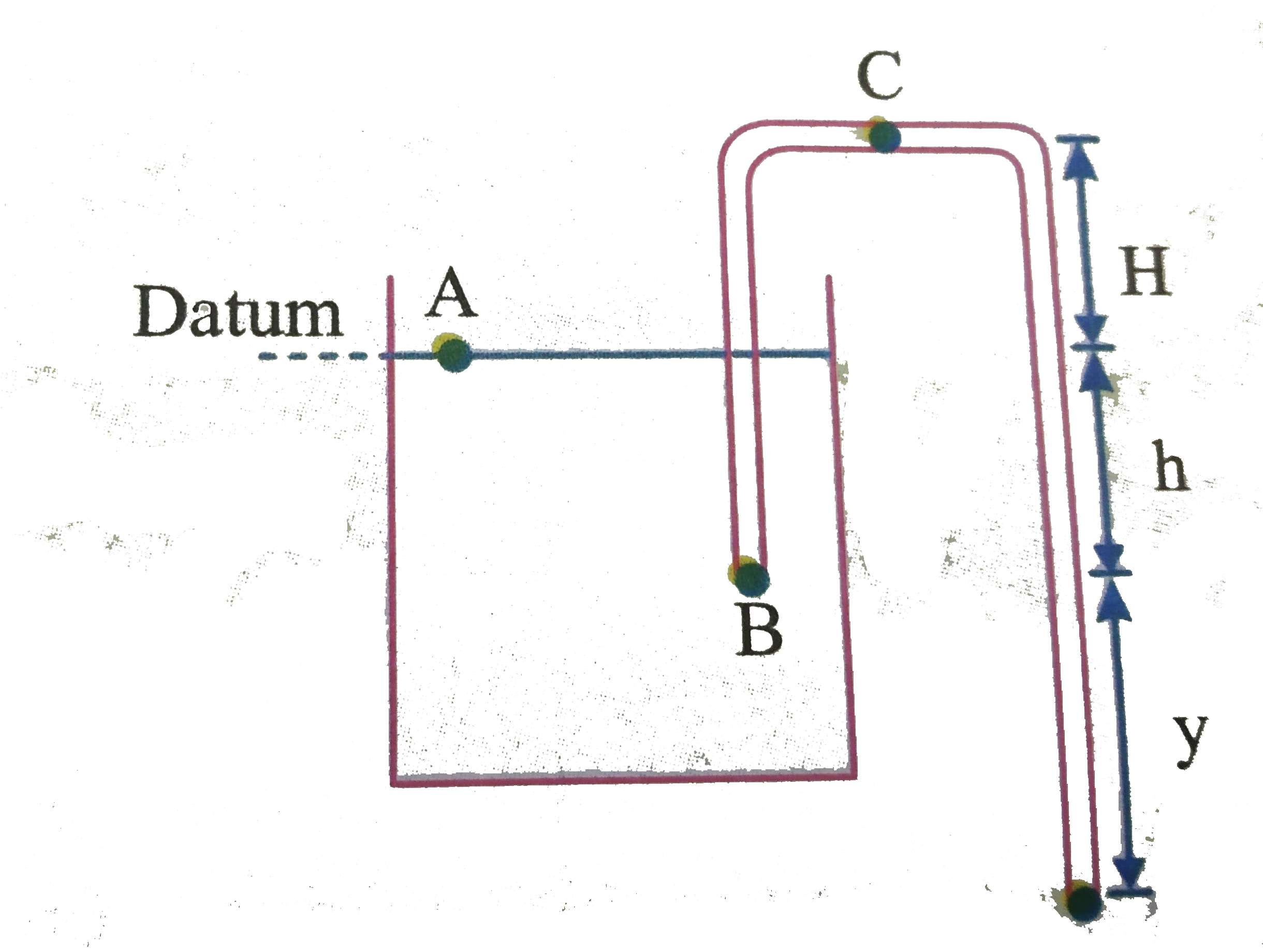
A siphon tube is used to remove liquid from a container as shown in fig. In order to operate the siphon tube, it must initially be filled with the liquid.
(i). Determine the pseed of the liquid through the siphon.
(ii). Determine the pressure at the point C.
View SolutionThe velocity of liquid flowing through a tube at certain distance from the axis of tube
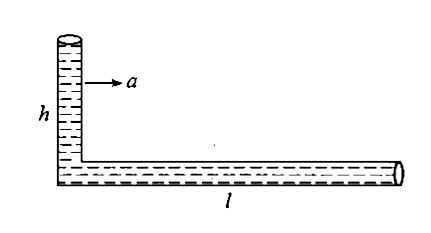
View SolutionFigure shows as a L-shaped tube in which a liquid of density ρ is filled. Find with what acceleration the tube is accelerated towards right so that no liquid will fall out of the tube.
View SolutionWater rises in a capillary tube through a height l. If the tube is inclined to the liquid surface at 30∘ the liquid will rise in the tube upto it's length equal to
View Solution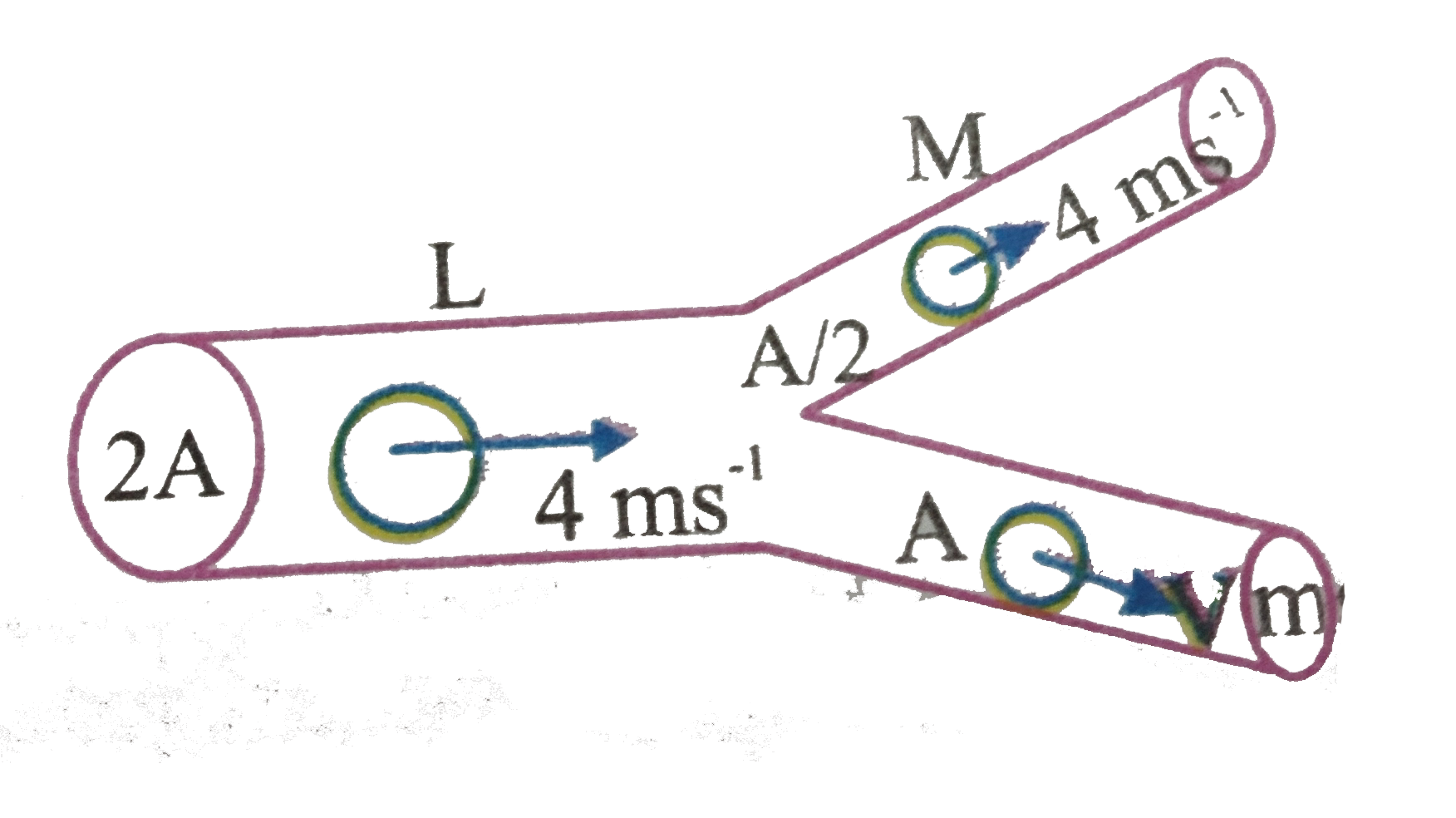
An incompressible liquid flows through a horizontal tube LMN as shown in the figure. Then the velocity V of the liquid throught he tube N is:
View Solution
