A 100 kg cubical box lies on a floor. A child pushes horizontally at a top edge. What force magnitude puts the box on the verge of tipping over if there is sufficient friction between it and the floor to prevent sliding?
To solve the problem of determining the force magnitude that puts a 100 kg cubical box on the verge of tipping over when pushed horizontally at a top edge, we can follow these steps:
Step 1: Understand the System
We have a cubical box with a mass of 100 kg resting on the floor. The box can tip over when a horizontal force is applied at its top edge. The weight of the box acts downwards at its center of gravity.
Step 2: Identify Forces Acting on the Box
- The weight of the box (W) = mass (m) × gravitational acceleration (g).
- The gravitational acceleration (g) is approximately 9.81 m/s².
- The force applied by the child (F) is horizontal.
Step 3: Calculate the Weight of the Box
The weight of the box can be calculated as follows:
W=m⋅g=100kg⋅9.81m/s2=981N
Step 4: Determine the Point of Tipping
The box will begin to tip over about the edge of the base that is opposite to where the force is applied. The distance from the pivot point (the edge of the box) to the center of gravity is half the length of the side of the box (A/2).
Step 5: Set Up the Torque Equation
For the box to be on the verge of tipping, the torque due to the applied force (F) must equal the torque due to the weight of the box (W).
- Torque due to the applied force (F) = F × A
- Torque due to the weight (W) = W × (A/2)
Setting the torques equal gives us:
F⋅A=W⋅A2
Step 6: Simplify the Equation
We can cancel A from both sides (assuming A ≠ 0):
F=W2
Step 7: Substitute the Weight of the Box
Now, substitute W with 981 N:
F=981N2=490.5N
Step 8: Conclusion
The magnitude of the force that the child must apply to put the box on the verge of tipping over is:
490.5N
---
We have a cubical box with a mass of 100 kg resting on the floor. The box can tip over when a horizontal force is applied at its top edge. The weight of the box acts downwards at its center of gravity.
- The weight of the box (W) = mass (m) × gravitational acceleration (g).
- The gravitational acceleration (g) is approximately 9.81 m/s².
- The force applied by the child (F) is horizontal.
The weight of the box can be calculated as follows:
W=m⋅g=100kg⋅9.81m/s2=981N
The box will begin to tip over about the edge of the base that is opposite to where the force is applied. The distance from the pivot point (the edge of the box) to the center of gravity is half the length of the side of the box (A/2).
For the box to be on the verge of tipping, the torque due to the applied force (F) must equal the torque due to the weight of the box (W).
- Torque due to the weight (W) = W × (A/2)
F⋅A=W⋅A2
We can cancel A from both sides (assuming A ≠ 0):
F=W2
Now, substitute W with 981 N:
F=981N2=490.5N
The magnitude of the force that the child must apply to put the box on the verge of tipping over is:
490.5N
Topper's Solved these Questions
- RIGID BODY DYNAMICS - IBOOK - RESNICK AND HALLIDAYCHAPTER - RIGID BODY DYNAMICS - IEXERCISE - PRACTICE QUESTIONS (SINGLE CORRECT CHOICE TYPE)
43 Videos
- RIGID BODY DYNAMICS - IBOOK - RESNICK AND HALLIDAYCHAPTER - RIGID BODY DYNAMICS - IEXERCISE - PRACTICE QUESTIONS (MORE THAN ONE CORRECT CHOICE TYPE)
7 Videos
- RIGID BODY DYNAMICS - IBOOK - RESNICK AND HALLIDAYCHAPTER - RIGID BODY DYNAMICS - IEXERCISE - CHECKPOINT
18 Videos
- RELATIVITYBOOK - RESNICK AND HALLIDAYCHAPTER - RELATIVITYEXERCISE - PRACTICE QUESTIONS (Integer Type)
5 Videos
- RIGID BODY DYNAMICS-IIBOOK - RESNICK AND HALLIDAYCHAPTER - RIGID BODY DYNAMICS-IIEXERCISE - PRACTICE QUESTIONS (Integer Type)
2 Videos
Similar Questions
25% of a uniform chain hangs from the edge of a horizontal table top. If the chain is on the verge of sliding,the coefficient of static friction between the chain and horizontal table is
View SolutionA sphere of mass m and radius R is kept on a rough horizontal floor. Constant force Facts at the top point of sphere along tangent. If there is sufficient friction on the floor to support pure rolling then calculate acceleration of sphere.
View Solution
Knowledge Check
A man of mass 80kg pushes box of mass 20kg horizontaly. The man moves the box with a constant acceleration of 2m/s2 but his foot does not slip on the ground. There is no friction between the box and the ground. There is no friction between the box and the ground. whereas there is sufficient friction between the man's foot and the ground to prevent him from slipping.
Assertion:- The force applied by the man on the box is equal and opposite to the force applied by the force applid by the box on the man.
Reason:- Friction force applied by the ground on the man is 200N.AIf both Assertion & Reason are True & the Reason is a correct explanation of the Assertion.BIf both Assertion & Reason are True but Reason is not a correct explanation of the Assertion.CIf Assertion is True but the Reason is False.DIf both Assertion & Reason are False.A man of mass 80kg pushes a box of mass 20kg horizontally. The man moves the box with a constant acceleration of 2m/s2 but his foot does not slip on the ground. There is no friciton between the box and the ground, whereas there is sufficient friction between the man's foot and the ground to prevent him from slipping.
Assertion:− The force applied by the man on the box is equal and opposite to the force applied by the force applied by the box on the man.
Reason :− Friction force applied by the ground on the man is 200N.AIf both Assertion & Reason are True & the Reason is a correct explanation of the Assertion.BIf both Assertion & Reason are True but Reason is not a correct explanation of the Assertiion.CIf Assertion is True but the Reason is False.DIf both Assertion & Reason are FalseA man of mass 75kg is pushing a heavy box on a flat floor. The coefficient of kinetic and static friction between the floor and the box is 0.20, and the coefficient of static friction between the man's shoes and the floor is 0.80. If the man pushes horizontally, what is the maximum values (in kg) of the box he can move?
 A300kgB60kgC900kgDnone of these
A300kgB60kgC900kgDnone of these
In Fig. 6-34 a block of weight W experiences two applied forces, each of magnitude W/2. What coefficient of static friction between the block and the floor puts the block on the verge of sliding?

View SolutionIf a box placed on a floor is pushed, do you think frictional force experienced by the box acts on the entire box or only on the surface of the box touching the floor? Give a reason for your answer.
View SolutionA 3.5 kg block is pushed along a horizontal floor by a force →F of magnitude 15 N at an angle θ=40∘ with the horizontal (Fig. 6-33). The coefficient of kinetic friction between the block and the floor is 0.25. Calculate the magnitudes of (a) the frictional force on the block from the floor and (b) the block's acceleration.

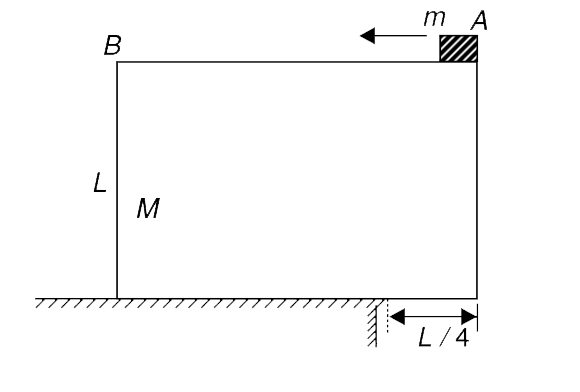
View SolutionA uniform cubical block of mass M and side length L is lying on the edge of a rough table with 14th of its edge overhanging. When a small block of mass m is placed on its top surface at the right edge (see fig.), the cube is on verge of toppling. The block of mass m is given a sharp horizontal impulse so that it acquires a velocity towards B. The small block moves on the top surface and falls on the other side. What is maximum coefficient of friction between the small block and the cube so that the cube does not rotate as the block moves over it. Assume that the friction between the cube and the table is large enough to prevent sliding of the cube on the table.
View SolutionA cubical box is filled with sand and weighs 520 N. We wish to "roll" the box by pushing horizontally on one of the upper edges. (a) What minimum force is required? (b) What minimum coefficient of static friction between box and floor is required? (c) If there is a more efficient way to roll the box, find the smallest possible force that would have to be applied directly to the box to roll it. (Hint: At the onset of tipping, where is the normal force located?)
View Solution
