A circle of radius 'r' passes through the origin $O$ and cuts the axes at A and B,Locus of the centroid of triangle OAB is

A

$x^{2} + y^{2} = k^{2}$

B

$x^{2} + y^{2} = 2 k^{2}$

C

$x^{2} + y^{2} = 3 k^{2}$

D

none of these

Video Solution

Text Solution

Verified by Experts

The correct Answer is:D

Let the coordinates of A and B be (a, 0) and (0, b) respectively . Clearly, $Δ O A B$ is a right-angled triangle, the hypotenuse AB is a diameter of the circle.

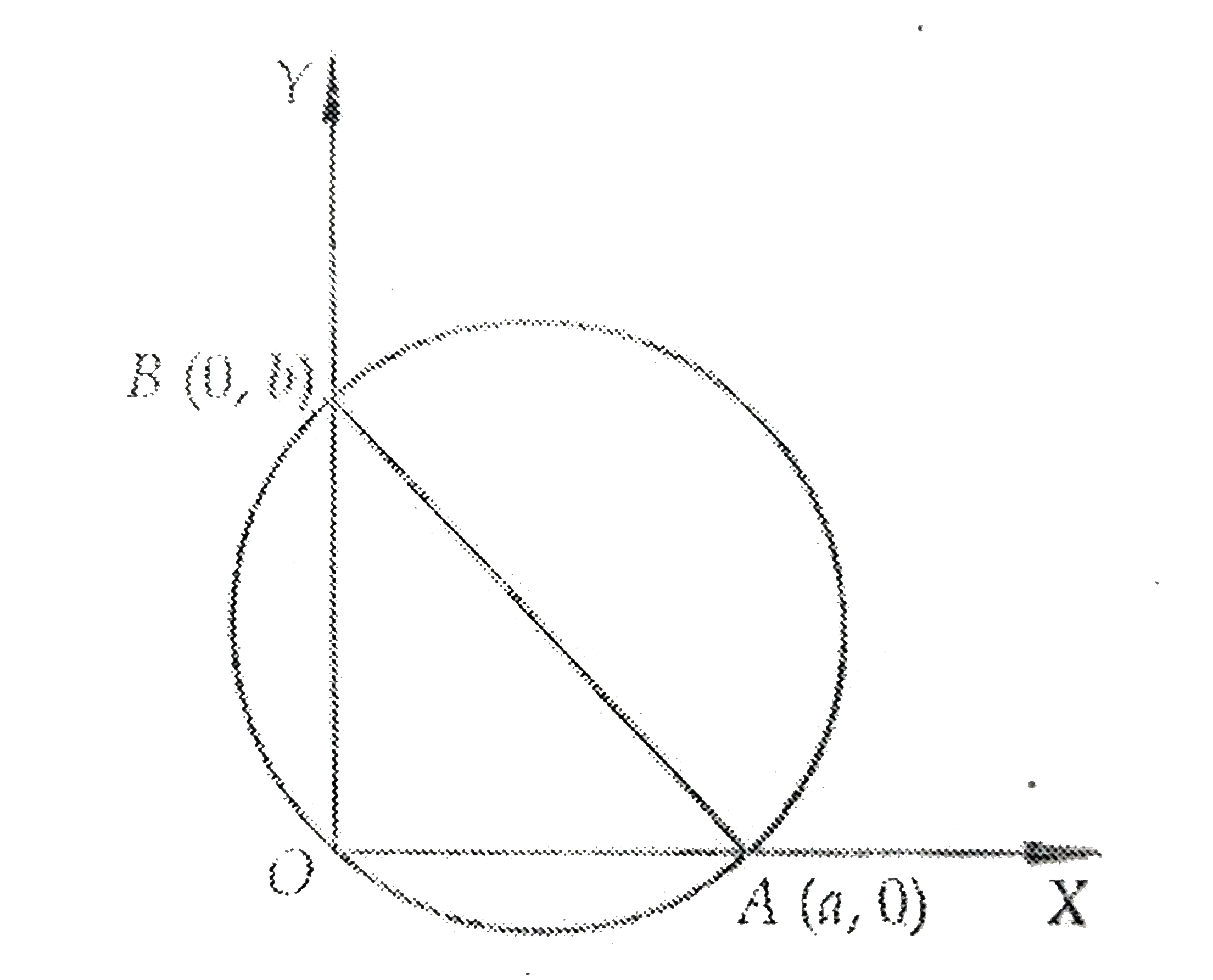
$∴ A B = 2 (3 k) = 6 k$ .
Now, $O A^{2} + O B^{2} = A B^{2} \Rightarrow a^{2} + b^{2} = 36 k^{2} ... (i)$
Let $(α, β)$ be the coordinates of the centroid of $Δ O A B$ . Then,
$α = a / 3, β = b / 3 \Rightarrow a = 3 α, b = 3 β$ .
Substituting the value of a and b in (i), we get
$9 α^{2} + 9 β^{3} = 36 k^{2} \Rightarrow α^{2} + β^{2} = 4 k^{2}$
Hence, the locus of $(α, β)$ is $x^{2} + y^{2} = 4 k^{2}$

|

Updated on:21/07/2023

Class 12 MATHS CIRCLES

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
A circle of constant radius 2r passes through the origin and meets the axes in 'P' and 'Q' Locus of the centroid of the $△ P O Q$ is :
A $x^{2} + y^{2} = r^{2}$
B $9 (x^{2} + y^{2}) = 16 r^{2}$
C $2 (x^{2} + y^{2}) = r^{2}$
D $3 (x^{2} + y^{2}) = 8 r^{2}$
Question 2 - Select One
A variable circle having fixed radius 'a' passes through origin and meets the co-ordinate axes in point A and B. Locus of centroid of triangle OAB where 'O' being the origin, is -
A $9 (x^{2} + y^{2}) = 4 a^{2}$
B $9 (x^{2} + y^{2}) = a^{2}$
C $9 (x^{2} + y^{2}) = 2 a^{2}$
D $9 (x^{2} + y^{2}) = 8 a^{2}$
Question 3 - Select One
A circle of constant radius r passes through the origin O, and cuts the axes at A and B. The locus of the foots the perpendicular from O to AB is $(x^{2} + y^{2}) = 4 r^{2} x^{2} y^{2}$ , Then the value of k is
A2
B1
C3
D4

OBJECTIVE RD SHARMA-CIRCLES-Section I - Solved Mcqs

Home
Ask Doubt
Profile