The number of points with integral coordinates that are interior to the circle x2+y2=16, is

A

43

B

49

C

45

D

51

Video Solution
Doubtnut Promotions Banner
Text Solution
Verified by Experts

The correct Answer is:C

The circle cuts the cordinates axes at (-4, 0), (4,0), (0, 4) and (0, -4). So, the number of point is equal to the number of integral solutions , (x, y) such that x2+y2<16, where −4<x<4 and −4<y<4
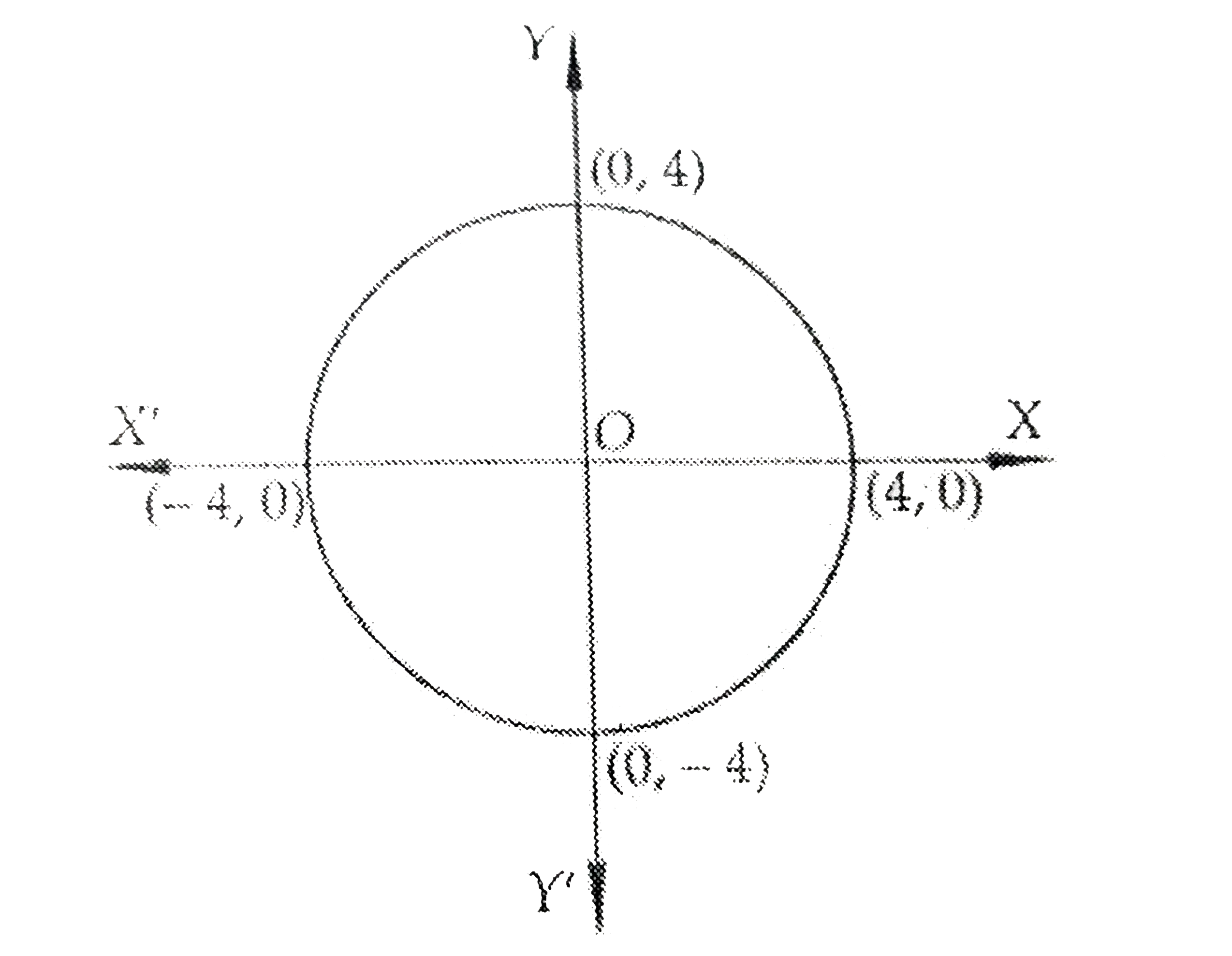
ocr_image
i.e., x∈[−3,3] and y∈[−3,3]
Clearly, x and y each can take 7 integral values, namely, -3, -2, -1, 0, 1, 2, 3. So, the number of ordered pairs (x, y) is 7 xx 7 = 49.<br>But,outofthese49orderedpairs(3,3),(−3,3),(−3,−3)and(3,−3)do¬satiyx^(2) + y^(2) lt 16.
Hence, required number of ordered pairs = 45.

Updated on:21/07/2023
|
Class 12MATHSCIRCLES

Topper's Solved these Questions

Similar Questions

Knowledge Check

  • The number of points with integral coordinates that lie in the interior of the region common to the circle x2+y2=16 and the parabola y2=4x, is

    A8
    B10
    C16
    Dnone of these

  • The range of values of θ∈[0,2π] for which (1+cosθ,sinθ) is on interior point of the circle x2+y2=1, is

    A(π/6,5π/6)
    B(2pu/3,5π/3)
    C(π/6,7π/6)
    D(2π/3,4π/3)

  • The coordinates of the foci for the hyperbola 9x2−16y2=144 are

    A(5.0 ) and(-5,0)
    B(2,0)and(-2,0)
    C(4,0) and (-4, 0)
    D(1,0) and (-1,0)