The number of real solution (x,y) where |y|=sinx,y=cos−1(cosx),−2π≤x≤2π is
A
2
B
1
C
3
D
4
Video Solution
Text Solution
The correct Answer is:C
Graph of |y| =sin x and y = cos−1 are shown in fig 19 it is evident form the graph that the curves inersect at (0,0)(2,π,0) and (−2π,0) hence there are 3 solution
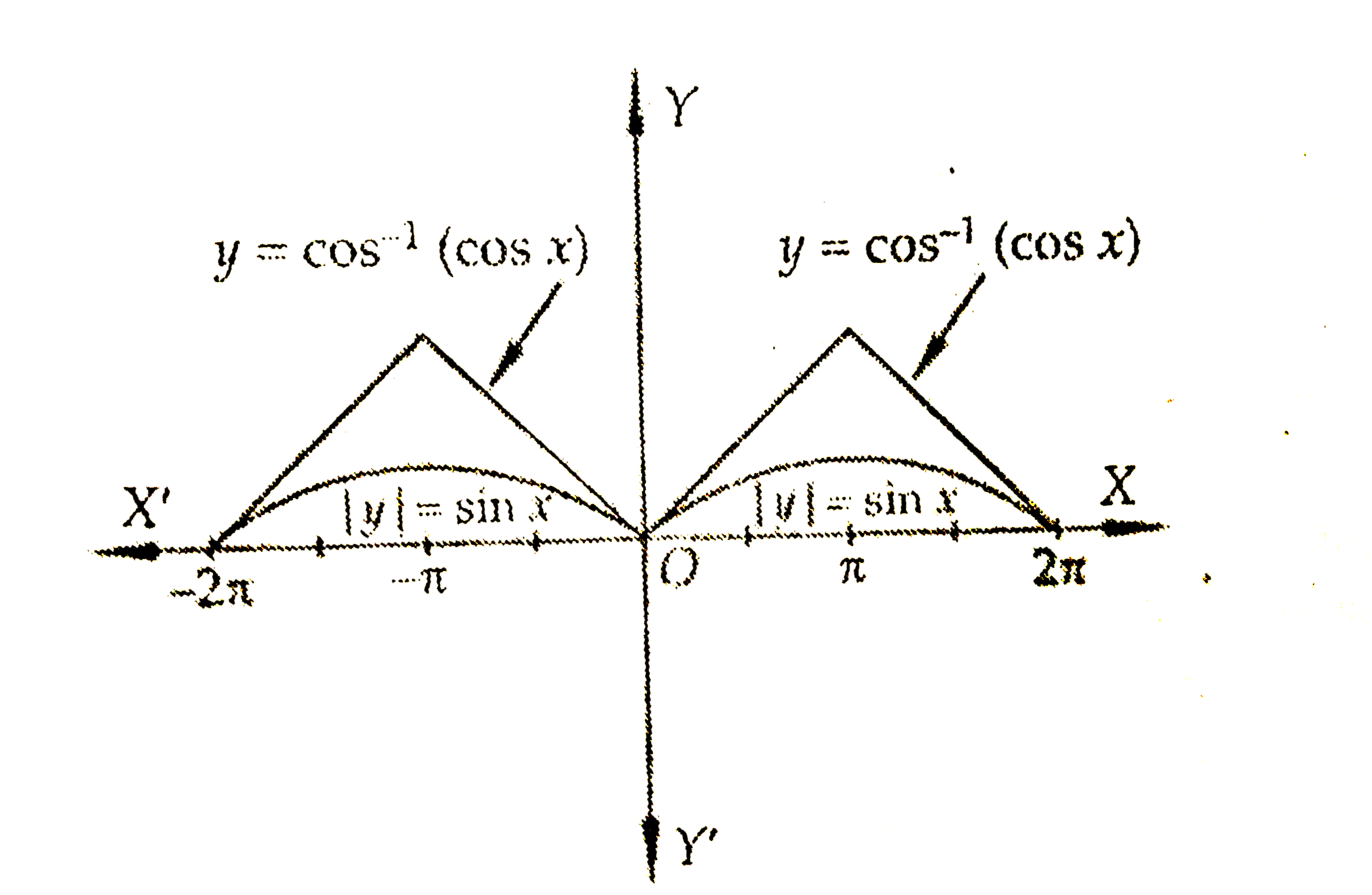
|
Topper's Solved these Questions
- INVERSE TRIGONOMETRIC FUNCTIONS BOOK - OBJECTIVE RD SHARMACHAPTER - INVERSE TRIGONOMETRIC FUNCTIONS EXERCISE - Section II - Assertion Reason Type
11 Videos
- INVERSE TRIGONOMETRIC FUNCTIONS BOOK - OBJECTIVE RD SHARMACHAPTER - INVERSE TRIGONOMETRIC FUNCTIONS EXERCISE - Exercise
72 Videos
- INVERSE TRIGONOMETRIC FUNCTIONS BOOK - OBJECTIVE RD SHARMACHAPTER - INVERSE TRIGONOMETRIC FUNCTIONS EXERCISE - Chapter Test
30 Videos
- INTEGRALSBOOK - OBJECTIVE RD SHARMACHAPTER - INTEGRALSEXERCISE - Illustration
1 Video
- LIMITSBOOK - OBJECTIVE RD SHARMACHAPTER - LIMITSEXERCISE - Chapter Test
60 Videos
Similar Questions
Knowledge Check
The number of solutions of (x,y), which satisfy |y|=sin x and y=cos−1(cosx), where −2π≤x≤2π is
A3B2C4DNone of theseThe number of real solutions of y+y2=sinxandy+y3=cos−1(cosx) is/are
A0B1C3DInfiniteThe number of real solutions of the equation
√1+cos2x=√2cos−1(cosx) in [π2,π] isA0B1C2D∞
Find the number of solutions of (x, y) which satisfy |y|=cosx and y=sin−1(sinx), where |x|≤3π.
View SolutionThe number of solutions of the equation |y|=cosxandy=cot−1(cotx) in (−3π2,5π2) is
View SolutionThe number of real solutions of equation √1+cos2x=√2sin−1(sinx),−10π≤x≤10π is/are
View SolutionIn the interval [−π4,π4] the number of real solutions of the equations ∣∣ ∣∣sinxcosxcosxcosxsinxcosxcosxcosxsinx∣∣ ∣∣=0
View SolutionIn the interval [−π4,π4], the number of real solutions of the equations ∣∣ ∣∣sinxcosxcosxcosxsinxcosxcosxcosxsinx∣∣ ∣∣
View Solution
