A uniform magnetic field $\to B$ is set up along the positive x-axis. A particle of charge 'q' and mass 'm' moving with a velocity $\to v$ entresthe field at the origin in X-Y plane such that it has velocity companents both along and perpendicular to the magnetic field $\to B$ . Trace, giving reason. the fraectory followed by the particle . Find out the expression for the distance moved by the particle along the magnetic field in one rotation.

Text Solution

Verified by Experts

field at an axial point of a circular coil:
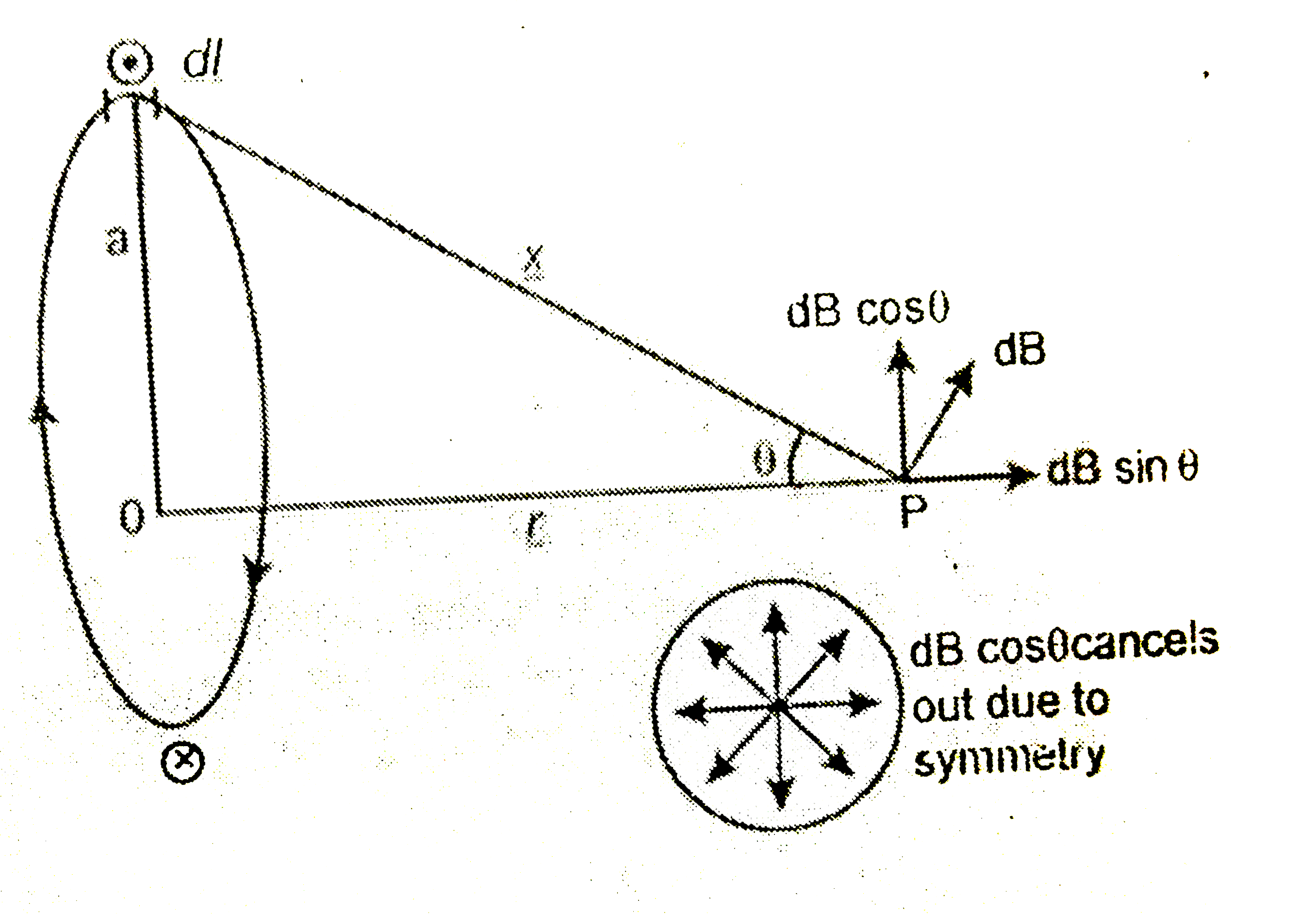
$B = \oint d B sin θ$
where, $d B = \frac{μ_{0}}{4 π} \frac{id l}{x^{2}}$
Where N is the number of turns of the circular coil. If M -iA is the magnetic moment of the coil, then
$sin θ = \frac{a}{x} \Rightarrow B = \frac{μ_{0}}{4 π} \frac{i a}{x^{3}} \oint d l = \frac{μ_{0}}{4 π} \frac{i a (2 π a)}{{(r^{2} + a^{2})}^{3 / 2}}$
$B = \frac{μ_{0} i a^{2} N}{2 {(r^{2} + a^{2})}^{3 / 2}}$
Where, N is the number of turns of the circular, coil. If M =iA is the magnetic moment of the coil, then
$M = i π a^{2}$ for single turn
$M = π a^{2}$ for a coil of N turns
$B = \frac{μ_{0}}{4 π} . \frac{2 M}{{(r^{2} + a^{2})}^{3 / 2}}$
for small loop,
$B = \frac{μ_{0}}{4 π} . \frac{2 M}{r^{3}} W b / m^{2}$
This is a similar result as obtained end on position of electric dipole where,
$E = \frac{1}{4 π e_{0}} . \frac{2 p}{r^{3}}$ ( where is electric moment.

Updated on:21/07/2023

A charged particle is moving with velocity Vin magnetic field
AIts momentum changes but total energy remains same
Bboth momentum and total energy remains same
Cboth momentum and total energy change
Dtotal energy changes but momentum remains the same
If a velocity has both perpendicular and parallel components while moving through a magnetic field, what is the path followed by a charged particle?
ALinear
BCircular
CHelical
DElliptical
A charge particle is moving in a plane perpendicular to uniform magnetic field, then
AIts momentum changes but kinetic energy remains the same
BBoth momentum and kinetic energy remain the same
CBoth will change
DKinetic energy change but momentum remains the same