A uniform magnetic field →B is set up along the positive x-axis. A particle of charge q and mass m moving with a velocity →v enters the field at the origin in X - Y plane such that it has velocity components both along and perpendicular to the magnetic field →B. Trace, giving reasons, the trajectory followed by the particle. Find out the expression for the distance moved by the particle along the magnetic field in one rotation.
Consider a charged particle of mass m and charge q entering a uniform magnetic field →B with a velocity →v along an angle θ with the direction of magnetic field in the plane of peper .
Velocity →v may be resolved into two components(i) vcosθ along the magnetic field, and (ii) vsinθ perpendicular to the magnetic field as shown in fig. Obviously due to normal component vsinθ the charged particle will ecperience a force F=q(vsinθ)B along a direction perpendicular to →B as well as (vsinθ) i.e, force F is along the z-axis. Under its effect, the charged particle describe a circular path of radius r, such that
Bqvsinθ=m(vsinθ)2r
⇒r=mvsinθBq ......(i)
The time period of revolution will be given by
T=2πr(vsinθ)=2πmBq .......(ii)
or Frequency of revolution v=1T=Bq2πm ........(iii)
Due to component vcosθ the charged particle does not experience force due to magnetic field and tends to move linearly with a constant speed. Thus, under the combined effect of both the velocity componetns we can say that the charged particle will describe a helical path having its axis parallel to magnetic field.
In one complete revolution of its circular path the charged particle covers a linear distance along →B, which is called as "pitch" of helix and is denoted by p. Obviously
p=(vcosθ)T=2πmvcosθBq

Topper's Solved these Questions
- MOVING CHARGES AND MAGNETISM BOOK - U-LIKE SERIESCHAPTER - MOVING CHARGES AND MAGNETISM EXERCISE - LONG ANSWER QUESTIONS-II
11 Videos
- MOVING CHARGES AND MAGNETISM BOOK - U-LIKE SERIESCHAPTER - MOVING CHARGES AND MAGNETISM EXERCISE - SELF ASSESSMENT TEST (SECTION A) (MULTIPLE CHOICE QUESTIONS)
5 Videos
- MOVING CHARGES AND MAGNETISM BOOK - U-LIKE SERIESCHAPTER - MOVING CHARGES AND MAGNETISM EXERCISE - SHORT ANSWER QUESTIONS
41 Videos
- MODEL TEST PAPER 3 (UNSOLVED)BOOK - U-LIKE SERIESCHAPTER - MODEL TEST PAPER 3 (UNSOLVED)EXERCISE - SECTION A
3 Videos
- NUCLEIBOOK - U-LIKE SERIESCHAPTER - NUCLEIEXERCISE - Self Assessment Test
10 Videos
Similar Questions
A uniform magnetic field →B is set up along the positive x-axis. A particle of charge 'q' and mass 'm' moving with a velocity →v entresthe field at the origin in X-Y plane such that it has velocity companents both along and perpendicular to the magnetic field →B. Trace, giving reason. the fraectory followed by the particle . Find out the expression for the distance moved by the particle along the magnetic field in one rotation.
View SolutionWhat is the direction of the force acting on a charged. Particle q moving with a velocity →v in a uniform magnetic field →B ?
View Solution
Knowledge Check
If a velocity has both perpendicular and parallel components while moving through a magnetic field, what is the path followed by a charged particle?
ALinearBCircularCHelicalDEllipticalA charged particle moves with velocity →v in a uniform magnetic field →B. The magnetic force experienced by the particle is
AAlways zeroBNever zeroCZero, if →B and →v are perpendicularDZero, if →B and →vare parallelA particle of charge q and mass m moving with a velocity v along the x-axis enters the region x>0 with uniform magnetic field B along the ˆk direction. The particle will penetrate in this region in the x-direction upto a distance d equal to
AZeroBmvqBC2mvqBDinfinity
A particle of charge q and mass m moving with a velocity v along the x-axis enters the region x>0 with uniform magnetic field B along the ˆk direction. The particle will penetrate in this region in the x-direction upto a distance d equal to
View SolutionA positive charge particle of charge q, mass m enters into a uniform magnetic field with velocity v as shown in the figure. There is no magnetic field to the left of PQ. Find
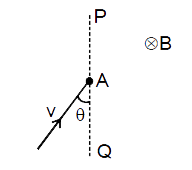
distance travelled in the magnetic field
View SolutionA charged particle is moved along a magnetic field line. The magnetic force on the particle is
View SolutionA charged particle moves with a velocity →v in a uniform magnetic field →B. The magnetic force experienced by the particle is
View SolutionA charged particle is moved along a magnetic field line. The magnetic force on the particle is
View Solution
