Two particles A and B of masses m and 2m have charges q and 2q respectively. Both these particle moving with velocities $v_{1} and v_{2}$ respectively in the same direction enter the same magnetic field B acting normally to their direction of motion. If the two force $F_{A} and F_{B}$ acting on them are in the ratio 1:2 , find the ratio of their velocities.

Text Solution

Verified by Experts

As force $F = q v B$ , hence $\frac{F_{A}}{F_{B}} = \frac{1}{2} = \frac{q_{A} v_{A} B}{q_{B} v_{B} B} = \frac{q v_{A}}{2 q v_{B}} = \frac{v_{A}}{2 v_{B}} \Rightarrow v_{A} = v_{B}$

|

Updated on:21/07/2023

Class 12 PHYSICS MOVING CHARGES AND MAGNETISM

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
A proton and an alpha-particle enter a magnetic field in a direction perpendicular to it. If the force acting on the proton is twice that acting on the alpha-particle , the ratio of their velocities is
A $4 : 1$
B $1 : 4$
C $1 : 2$
D $2 : 1$
Question 2 - Select One
Two particles A and B of masses 10 kg and 38 kg, respectively, are moving along the same straight line with velocities 15 m/s and 3 m/s, respectively, in the same direction. After elastic collision, the velocities of A and B are $v_{A}$ and $v_{B}$ , respectively, in the direction of initial motion. Then

A $v_{A} = 20 m / s, v_{B} = 8 \frac{m}{s}$
B $v_{A} = - 4 m / s, v_{B} = 8 m / s$
C $v_{A} = 16 \frac{m}{s}, v_{B} = 28 m / s$
D $v_{A} = - 5 m / s, v_{B} = 10 m / s$
Question 3 - Select One
Two particles $A and B$ of masses $m_{A} and m_{B}$ respectively and having the same charge are moving in a plane . The speeds of the particles are $v_{A} and v_{B}$ respectively and the trajectories are as shown in the figure. Then

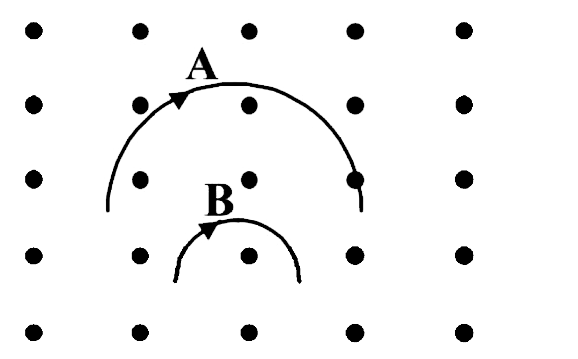A $m_{A} v_{A} < m_{B} v_{B}$
B $m_{A} v_{A} > m_{B} v_{B}$
C $m_{A} v_{A} < m_{B} and v_{A} < v_{B}$
D $m_{A} = m_{B} and v_{a} = v_{B}$

U-LIKE SERIES-MOVING CHARGES AND MAGNETISM -VERY SHORT ANSWER QUESTIONS

Home
Ask Doubt
Profile