Question

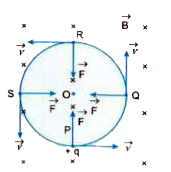
Consider the motion of a charged particle of mass m and charge q moving with velocity $\overrightarrow{v}$ in a magnitude field $\overrightarrow{B}$. If $\overrightarrow{v}$ is perpendicular to $\overrightarrow{B}$, show that it describe a circular path having angular frequency $\omega =\frac{qB}{m}$

Explanation

When a charged particle having charge q and moving with a velocity `vecv` enters a uniform magnetic field `vecB`, it experiences a Lorentz force `vecF = q(vecv xx vecB)` whose direction is given by Fleming.s left hand rule. The force is maximum when charged particle is moving perpendicular to the magnetic field. In that case
`F = q v B sin 90^@ = q v B`
As the force is acting perpendicular to both `vecv and vecB`, it does not change the magnitude of velocity but changes its direction from a straight from a straight line path to a circular path of radius r such that the magnetic force - centripetal force needed.
`:. B q v = (mv^2)/(r) implies r = (mv)/(Bq)`
The time period and frequency of the charged particle in its circular path will be
`T = (2 pi r)/(v) = (2pi ((mv)/(Bq)))/(v) = (2 pi m)/(Bq) , ` Frequency `v = 1/T = (Bq)/(2 pi m)`
and angular frequency `omega = 2 pi v = (qB)/(m)`.
From this relation it is clear that the time period (as well as frequency) is independent of the velocity (energy) of the charged particle as well as the radius of the circular path.