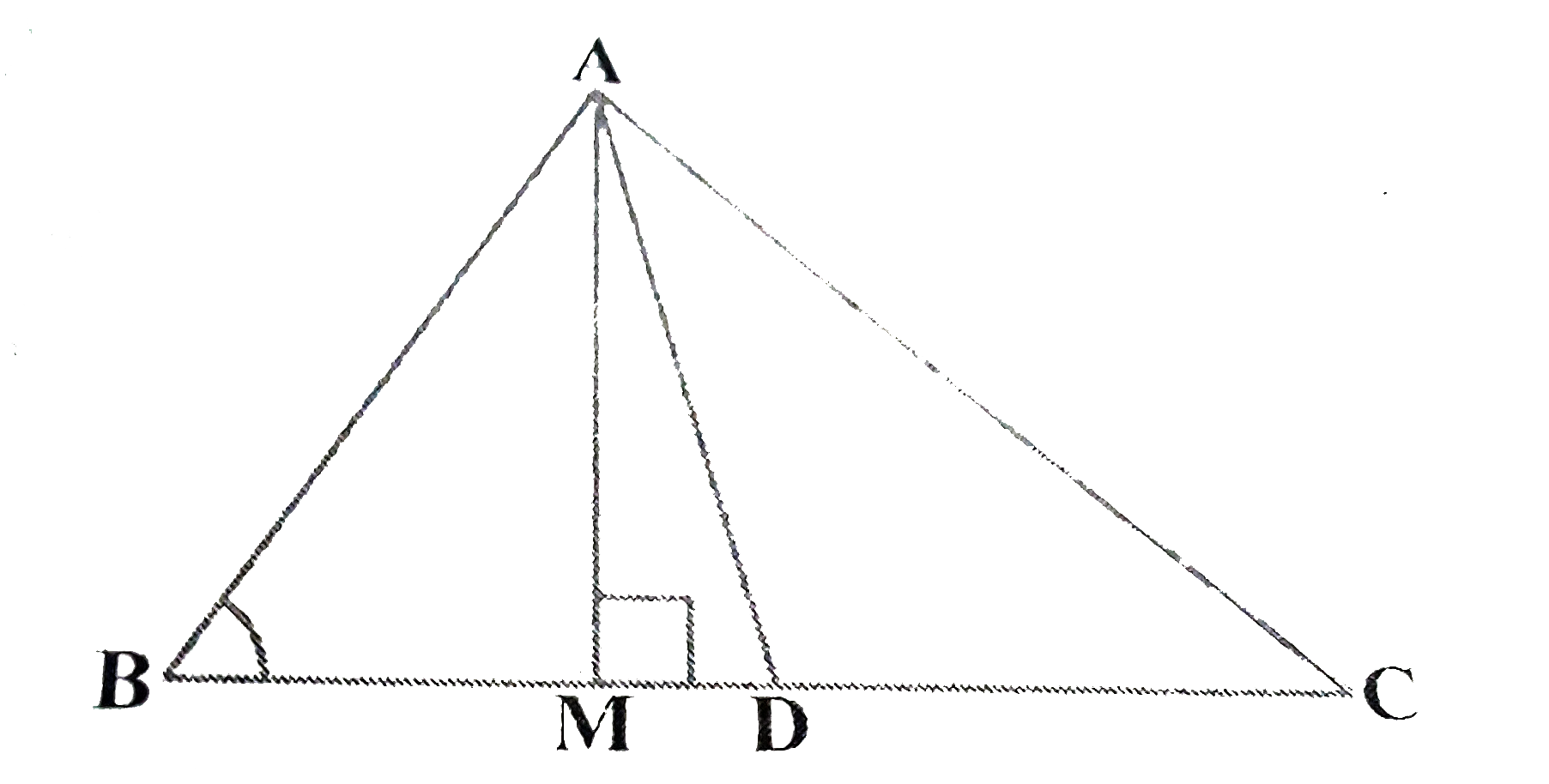
In figure, ABC is triangle in which ∠ABC>90∘ and AD⊥CBproduced. Prove that AC2=AB2+BC2+2BC.BD.

(##X06_E0603_s10##)
Using Pythagoras Theorem In ΔADC as ∠ADC=90°
AC2=AD2+DC2
AC2=AD2+[BC−BD]2
AC2=AD2+BD2+BC2−2BC.BD
AC2=AB2+BC2−2BC.BD [∵ In ΔADB, AB2=AD2+BD2]
Hence it is proved that AC2=AB2+BC2−2BC.BD.
Topper's Solved these Questions
Similar Questions
In figure, ABC is a triangle in which ∠ABC=90∘and AD⊥BC. Prove that AC2=AB2+BC2−2BC.BD.

View SolutionIn Figure, ABC is an obtuse triangle, obtuse angled at B . If AD⊥CB , prove that AC2=AB2+BC2+2 BC×BD .
View SolutionABC is a triangle in which AB=AC and D is any point in BC . Prove that AB2−AD2=BDCD .
View SolutionIn Figure, ∠B of ABC is an acute angle and AD⊥BC , prove that AC2=AB2+BC2−2 BC×BD
View SolutionIn figure, AD is a median of a triangle ABC and AM⊥BC. Prove that:
(i) AC2=AD2+BC.DM+(BC2)2
(ii) AB2=AD2−BC.DM+(BC2)2
(iii) AC2+AB2=2AD2+12BC2
View SolutionIn fig., if AD⊥BC, prove thatAB2+CD2=BD2+AC2.
View SolutionIn Figure, AD⊥BC and BD=13CD. Prove that 2CA2=2AB2+BC2
View SolutionIn figure ∠ACB=90∘and CD⊥AB. Prove that BC2AC2=BDAD.
View SolutionABCD is a rhombus. Prove that AB2+BC2+CD2+DA2=AC2+BD2
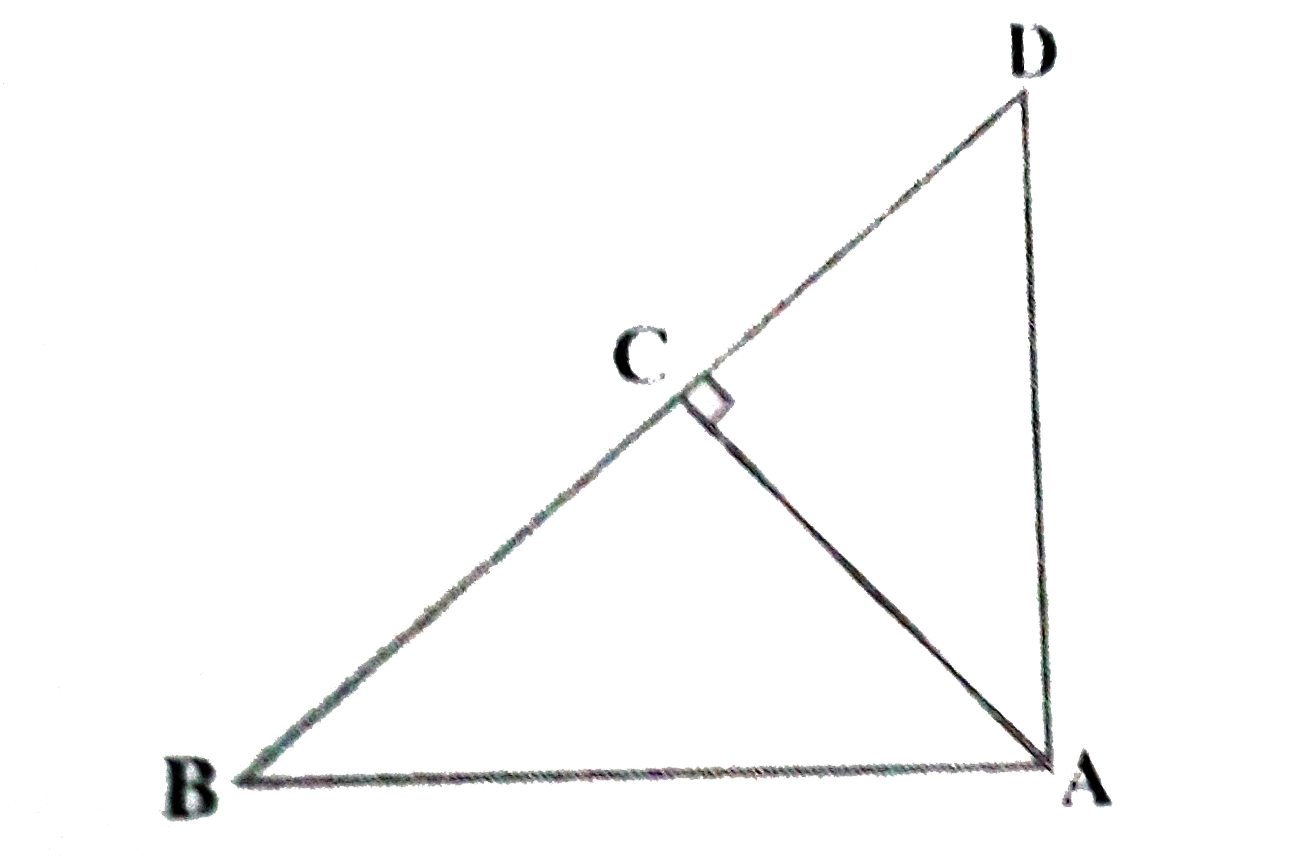
View SolutionIn Figure, ABD is a triangle right-angled at A and AC⊥BD. Show that
(i)AB2=BC.BD
(ii) AC2=BC.DC
(iii) AD2=BD.CD
View Solution
