In Figure, ABC is an obtuse triangle, obtuse angled at B . If AD⊥CB , prove that AC2=AB2+BC2+2 BC×BD .
To prove that AC2=AB2+BC2+2BC⋅BD in triangle ABC where AD⊥CB and B is the obtuse angle, we can follow these steps:
Step 1: Identify the triangles
We have triangle ABC with ∠B being obtuse. The point D is on line CB such that AD is perpendicular to CB.
Step 2: Apply the Pythagorean theorem in triangle ADB
In triangle ADB, we can apply the Pythagorean theorem:
AB2=AD2+BD2(1)
Step 3: Apply the Pythagorean theorem in triangle ADC
In triangle ADC, we also apply the Pythagorean theorem:
AC2=AD2+DC2(2)
Step 4: Express DC in terms of BD and BC
Since D lies on CB, we can express DC as:
DC=BD+BC
Step 5: Substitute DC into equation (2)
Now substitute DC in equation (2):
AC2=AD2+(BD+BC)2
Step 6: Expand the square
Expanding (BD+BC)2:
AC2=AD2+(BD2+2⋅BD⋅BC+BC2)
Step 7: Substitute AD2 from equation (1)
From equation (1), we have AD2=AB2−BD2. Substitute this into the equation:
AC2=(AB2−BD2)+(BD2+2⋅BD⋅BC+BC2)
Step 8: Simplify the equation
Now, simplify the equation:
AC2=AB2−BD2+BD2+2⋅BD⋅BC+BC2
The −BD2 and +BD2 cancel each other out:
AC2=AB2+BC2+2⋅BD⋅BC
Conclusion
Thus, we have proved that:
AC2=AB2+BC2+2BC⋅BD
Hence, the statement is proved.
We have triangle ABC with ∠B being obtuse. The point D is on line CB such that AD is perpendicular to CB.
In triangle ADB, we can apply the Pythagorean theorem:
AB2=AD2+BD2(1)
In triangle ADC, we also apply the Pythagorean theorem:
AC2=AD2+DC2(2)
Since D lies on CB, we can express DC as:
DC=BD+BC
Now substitute DC in equation (2):
AC2=AD2+(BD+BC)2
Expanding (BD+BC)2:
AC2=AD2+(BD2+2⋅BD⋅BC+BC2)
From equation (1), we have AD2=AB2−BD2. Substitute this into the equation:
AC2=(AB2−BD2)+(BD2+2⋅BD⋅BC+BC2)
Now, simplify the equation:
AC2=AB2−BD2+BD2+2⋅BD⋅BC+BC2
The −BD2 and +BD2 cancel each other out:
AC2=AB2+BC2+2⋅BD⋅BC
Thus, we have proved that:
AC2=AB2+BC2+2BC⋅BD
Hence, the statement is proved.
Topper's Solved these Questions
Similar Questions
In Figure, ∠B of ABC is an acute angle and AD⊥BC , prove that AC2=AB2+BC2−2 BC×BD
View SolutionIn fig., if AD⊥BC, prove thatAB2+CD2=BD2+AC2.
View SolutionIn an equilateral ABC , AD⊥BC , prove that AD2=3 BD2
View SolutionIn figure, ABC is triangle in which ∠ABC>90∘ and AD⊥CBproduced. Prove that AC2=AB2+BC2+2BC.BD.

View SolutionIn Figure, ABC is a right triangle right angled at B and points DandE trisect BC . Prove that 8AE2=3AC2+5AD2.
View SolutionIn figure, ABC is a triangle in which ∠ABC=90∘and AD⊥BC. Prove that AC2=AB2+BC2−2BC.BD.

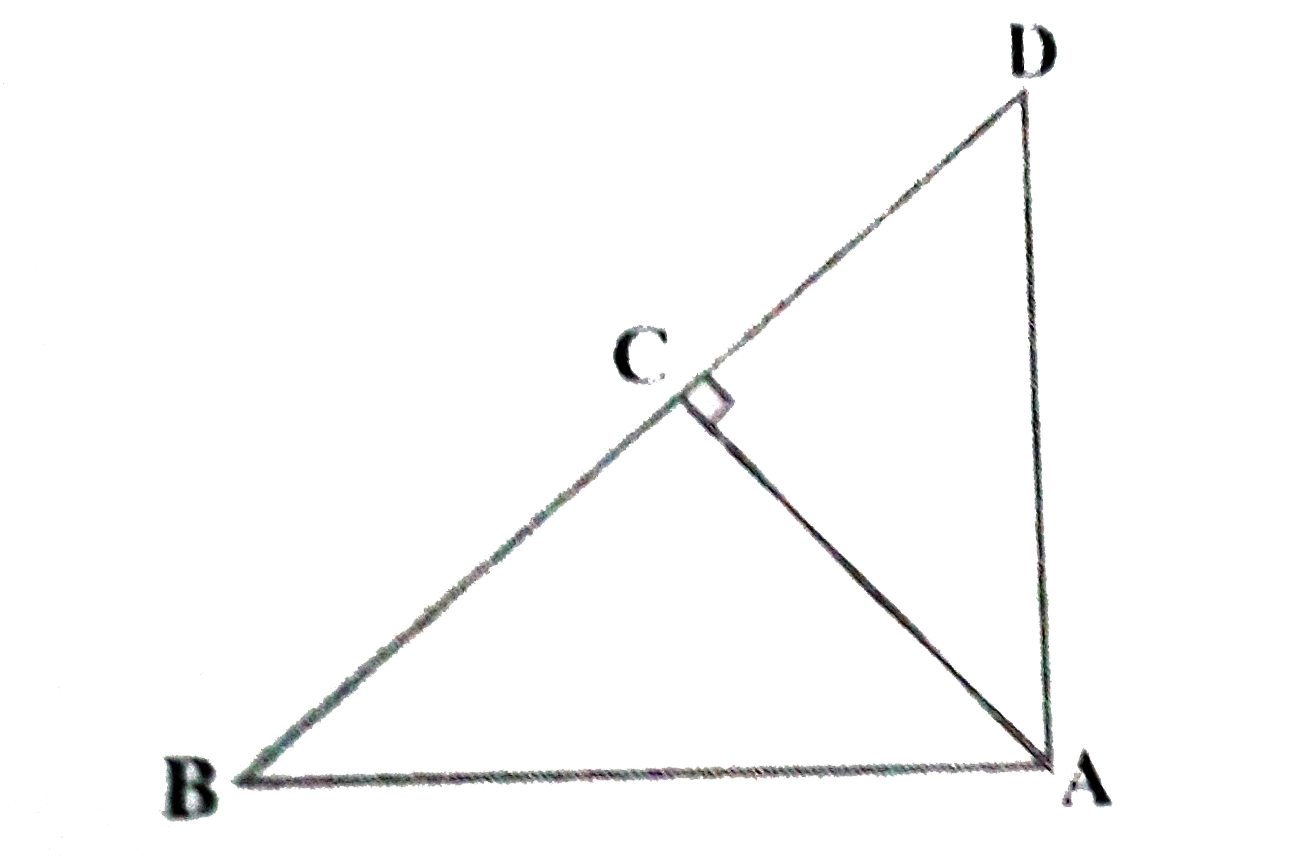
View SolutionIn Figure, ABD is a triangle right-angled at A and AC⊥BD. Show that
(i)AB2=BC.BD
(ii) AC2=BC.DC
(iii) AD2=BD.CD
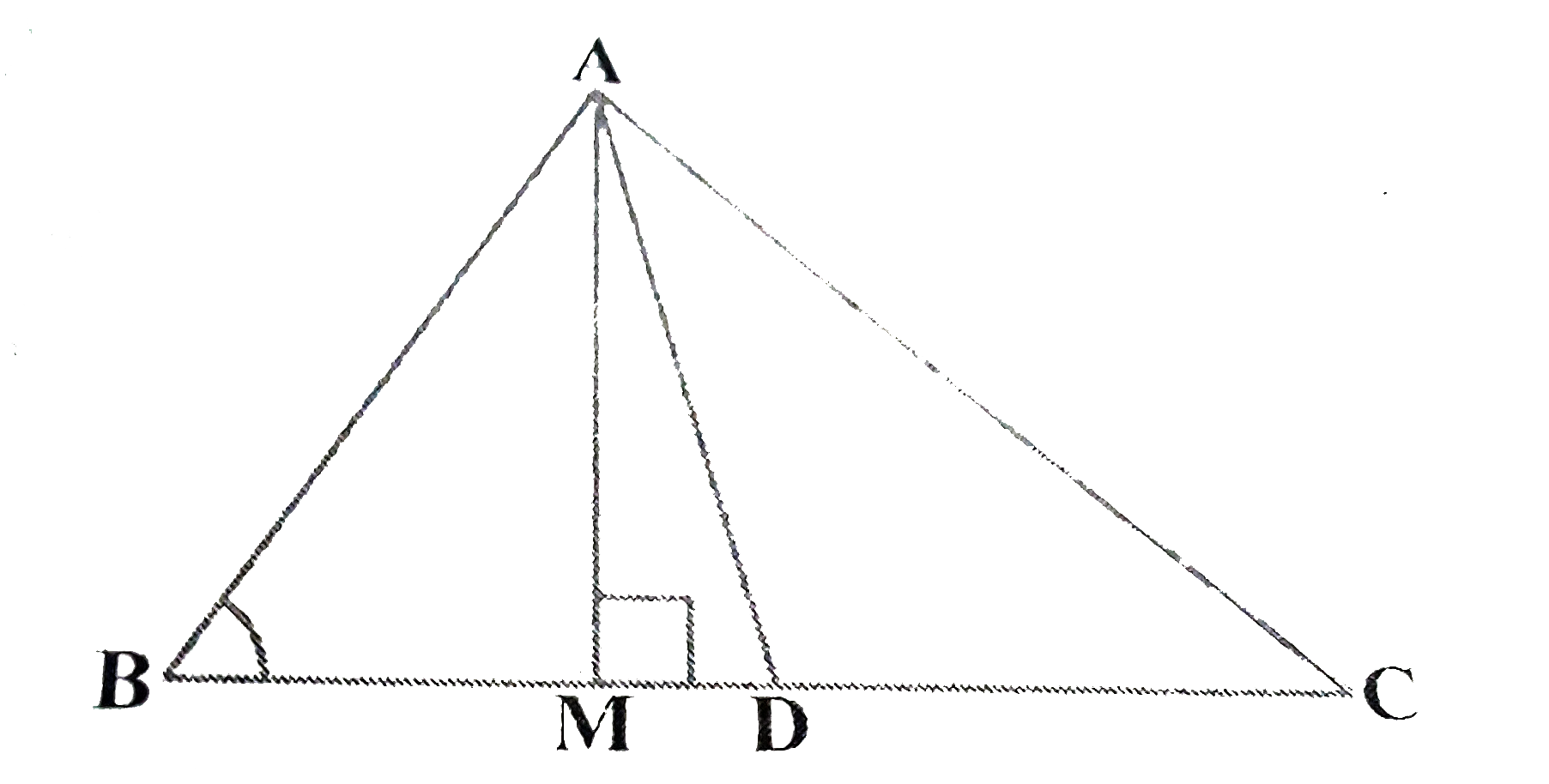
View SolutionIn figure, AD is a median of a triangle ABC and AM⊥BC. Prove that:
(i) AC2=AD2+BC.DM+(BC2)2
(ii) AB2=AD2−BC.DM+(BC2)2
(iii) AC2+AB2=2AD2+12BC2
View SolutionIn Figure, prove that CD+DA+AB+BC>2AC
View SolutionABC is an isosceles right triangle right-angled at C . Prove that AB2=2AC2 .
View Solution
