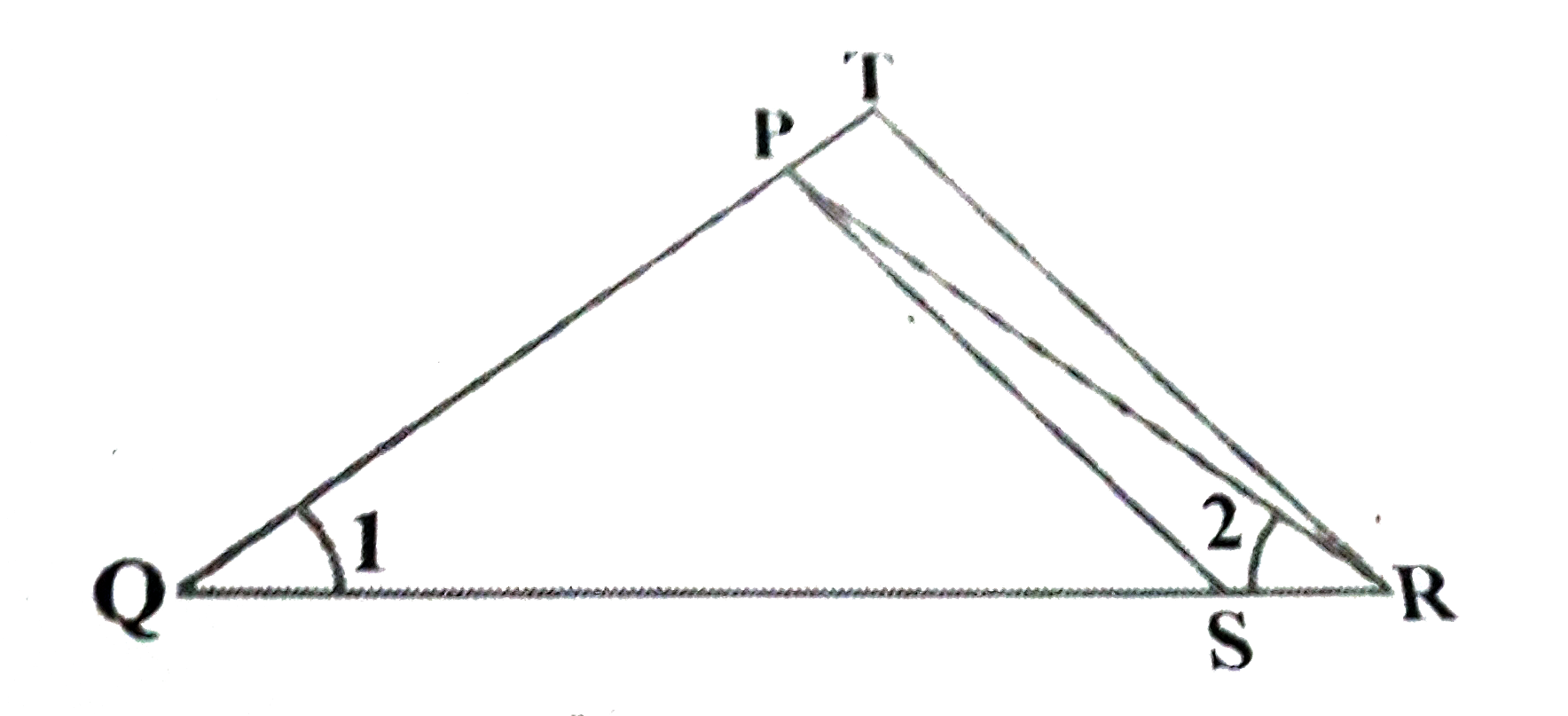
In Figure PSSQ=PTTRand ∠PST=∠PRQ. Prove that PQR is an isosceles triangle.
Video Solution
Text Solution
Here, we are given,
PSSQ=PTTR
As, ST is dividing two sides of triangle in equal ratio, it will be parallel to third side.
So, ST∣∣QR
That means,
∠PST=∠PQR
We are given,
∠PST=∠PRQ
So, ∠PRQ=∠PQR
As two angles of triangle ΔPQR are equal, it is an isosceles triangle.
|
Similar Questions
In Fig. 4.33, PSSQ=PTTR and ∠PST=∠PRQ . Prove that PQR is an isosceles triangle. (FIGURE)
View SolutionIn Figure, If PQ=PT and ∠TPS=∠QPR, prove that PRS is isosceles
View SolutionIn figure QRQS=QTPRand ∠1=∠2. Show that ΔPQS~ΔTQR.
View SolutionIn Fig. , if QTPR=QRQS and ∠1=∠2 . Prove that PQS~TQR . (FIGURE)
View SolutionIn Figure, PS=PR, ∠TPS= ∠QPR. Prove that PT=PQ
View SolutionIn Fig 7.51, PR > PQand PS bisects ∠QPR. Prove that ∠PSR >∠PSQ.

View SolutionIn Fig. 6.15, ∠PQR=∠PRQ, then prove that ∠PQS=∠PRT.

View SolutionIn Figure, PS=QR and ∠SPQ=∠RQP. Prove that PQS ≅ QPR, PR=QS and ∠QPR= ∠PQS
View SolutionIn Figure, ∠PQR= ∠PRQ, then prove that ∠PQS= ∠PRT.
View SolutionIn figure, PQ=PR . Show that PS>PQ.
View Solution
