In figure QRQS=QTPRand ∠1=∠2. Show that ΔPQS~ΔTQR.
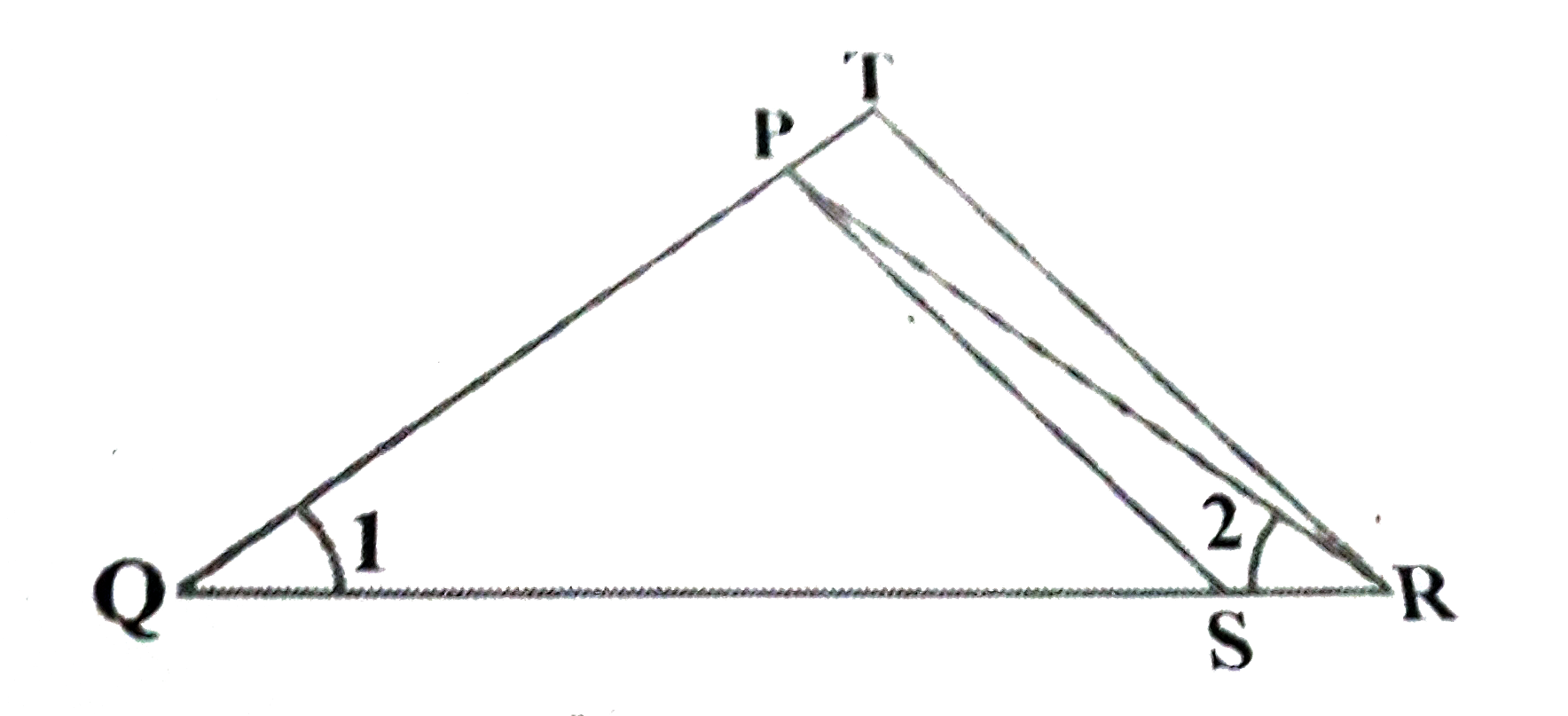
(##X06_E0304_s10##)
In △PQR,
Since, ∠1=∠2
∴ PR=PQ (Opposite sides of equal angles are equal) .....(1)
In △PQS and △TQR,
QRQS=QTPR
(Given)
i.e.,
QRQS=QTPR
....(From1)
Also, ∠Q is common
∴ By SAS criterion of similarity,
△PQS∼△TQR.
Topper's Solved these Questions
- TRIANGLESBOOK - NCERT ENGLISHCHAPTER - TRIANGLESEXERCISE - Exercise 6.2
10 Videos
- TRIANGLESBOOK - NCERT ENGLISHCHAPTER - TRIANGLESEXERCISE - SOLVED EXAMPLES
14 Videos
- TRIANGLESBOOK - NCERT ENGLISHCHAPTER - TRIANGLESEXERCISE - Exercise 6.5
17 Videos
- SURFACE AREAS AND VOLUMESBOOK - NCERT ENGLISHCHAPTER - SURFACE AREAS AND VOLUMESEXERCISE - Exercise 13.3
9 Videos
Similar Questions
In Fig. , if QTPR=QRQS and ∠1=∠2 . Prove that PQS~TQR . (FIGURE)
View SolutionS and T are points on sides PR and QR of ΔPQR such that ∠P=∠RTS. Show that ΔRPQ~ΔRTS.
View SolutionIn Figure PSSQ=PTTRand ∠PST=∠PRQ. Prove that PQR is an isosceles triangle.
View SolutionIn Fig. 4.33, PSSQ=PTTR and ∠PST=∠PRQ . Prove that PQR is an isosceles triangle. (FIGURE)
View SolutionIn Figure, PS=QR and ∠SPQ=∠RQP. Prove that PQS ≅ QPR, PR=QS and ∠QPR= ∠PQS
View SolutionIn Figure, If PQ=PT and ∠TPS=∠QPR, prove that PRS is isosceles
View SolutionIn figure, PQ=PR . Show that PS>PQ.
View SolutionIn Figure, PS=PR, ∠TPS= ∠QPR. Prove that PT=PQ
View SolutionIn figure Cm and RN are respectively the medians of ΔABCand ΔPQR. If ΔABC~ΔPQR, prove that: (i) ΔAMC~ΔPNR (ii) CMRN=ABPQ (ii) ΔCMB~ΔRNQ
View SolutionIn Figure, OP∣∣RS. Determine ∠PQR
View Solution
