Two charges ±10μC are placed 5⋅0mm apart. Determine the electric field at (a) point P on the axis of dipole 15cm away from its center on the side of the positive charge. As shown in Figure and at (b) a point Q. 15cm away form O on a line passing through O and a line passing through O and normal to the axis of the dipole as shown in Fig.
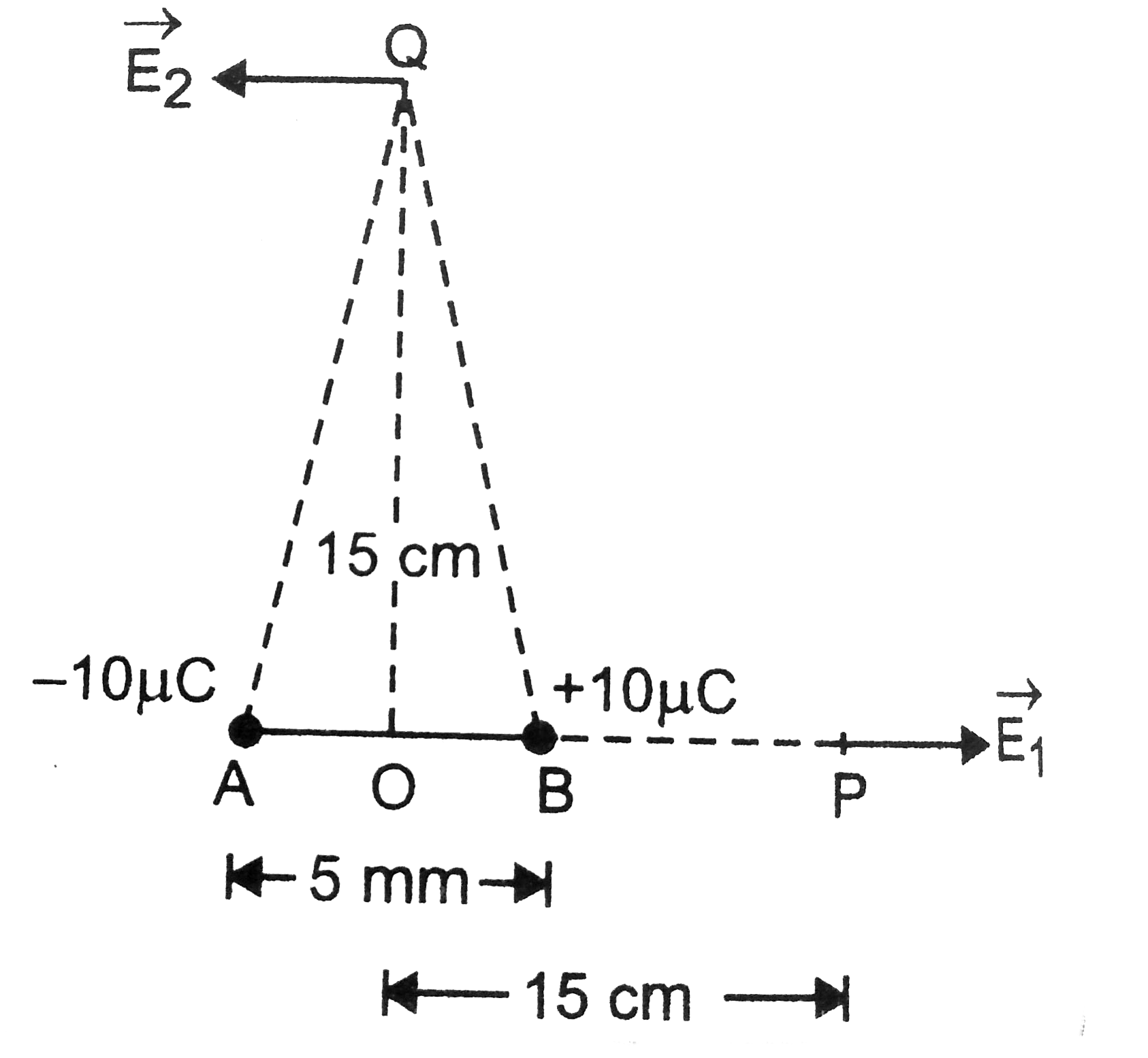
(a) Field at P due to charge +10μC
=108C4π(8.854×10−12C2N−1m−2)×1(15−0.25)3×10−4m3
=4.13×108NC−1along BP
Field at P due to charge −10μC
=108C4π(8.854×10−12C2N−1m−2)×1(15−0.25)3×10−4m3
The resultant electric field at P due to the two charges at A and B is
=2.7×105NC−1along BP.
In this example, the ratio OP/OB is quite large (= 60). Thus, we canexpect to get approximately the same result as above by directly usingthe formula for electric field at a far-away point on the axis of a dipole.For a dipole consisting of charges ± q, 2a distance apart, the electricfield at a distance r from the centre on the axis of the dipole has amagnitude
E=24πε0r3 (r/a>>1)
where p = 2a q is the magnitude of the dipole moment. The direction of electric field on the dipole axis is always along thedirection of the dipole moment vector (i.e., from –q to q).
Here,p=10−5C×5×10−3m=5×10−8Cm
Therefore
=108C4π(8.854×10−12C2N−1m−2)×1(15)3×10−4m3=2.6×105NC−1
along the dipole moment direction AB, which is close to the result obtained earlier.
(b) Field at Q due to charge +10μCatB
=105C4π(8.854×10−12C2N−1m−2)×1[152−(0.25)3]×10−4m2
=3.99×106NC−1along BQ ltBRgt Field at Q due to charge −10μCatA
=108C4π(8.854×10−12C2N−1m−2)×1[152−(0.25)3]×10−4m3
=3.99×106NC−1along QA.
Clearly, the components of these two forces with equal magnitudes cancel along the direction OQ but add up along the direction parallel to BA. Therefore, the resultant electric field at Q due to the two charges at A and B is =2×0.25√152+(0.25)2=3.99×106NC−1along BA
1.33×105NC−1along BAAs in (a), we can expect to get approximately the same result by directly using the formula for dipole field at a point on the normal to the axis of the dipole:
E=P4πε0r3 (r/a>>1)
=5×108Cm4π(8.854×10−12C2N−1m−2)×1(15)3×10−6m3
=1.33×105NC−1
The direction of electric field in this case is opposite to the direction of the dipole moment vector. Again, the result agrees with that obtained before.
Topper's Solved these Questions
- ELECTRIC CHARGES AND FIELDSBOOK - NCERT ENGLISHCHAPTER - ELECTRIC CHARGES AND FIELDSEXERCISE - Exercise
34 Videos
- DUAL NATURE OF RADIATION AND MATTERBOOK - NCERT ENGLISHCHAPTER - DUAL NATURE OF RADIATION AND MATTEREXERCISE - All Questions
32 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - NCERT ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Exercise
17 Videos
Similar Questions
Two charges 10μC are placed 5.0 mm apart. Determine the electric field at a point P on the axis of the dipole 15 cm away from its centre O on the side of the positive charge, as shown in Fig.

View SolutionTwo charges +20μCand−20μC are placed 10mm apart. The electric field at point P, on the axis of the dipole 10 cm away from its centre O on the side of the positive charge is
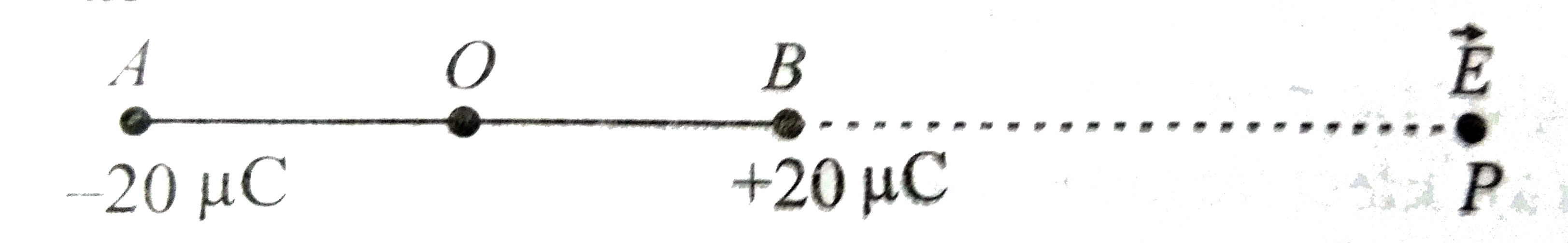
View SolutionTwo charges ±10μC are placed 5×10−3m apart. Determine the electric field at a point Q,0⋅15m away from O, on a line passing through O and normal to the axis of the dipole.
View SolutionTwo charges +5μC and +10μC are placed 20cm apart. The net electric field at the mid-point between the two charges is
View SolutionTwo charges +20μCand−20μC are held 1cm apart. Calculate the electric field at a point on the equatorial line at a distance of 50cm from the center of the dipole.
View SolutionTwo charges 4q and q are placed 30 cm. apart. At what point the value of electric field will be zero
View SolutionTwo charges +20μC and −20μC are 5 mm apart. Find the electric field on axial as well as on the equatorial line at a distance 20 cm from the mid -point of the dipole.
View SolutionTwo charges, each of 5μC but opposite in sign, are placed 4 cm apart. Calculate the electric field intensity of a point that is a distance 4 cm from the mid point on the axial line of the dipole.
View SolutionTwo point charges of +16μCand−9μC are placed 8 cm apart in air. Determine the position of the point at which the resultant electric field is zero.
View SolutionTwo point charges of 20μC and 80μC are 10 cm apart where will the electric field strength be zero on the line joining the charges from 20μC charge
View Solution
