A trolly of mass 200kg moves with a uniform speed of 36 km/h on a frictionless track. A child of mass 20kg runs on the trolly from one end to the other (10m away) with a speed of 4m/s relative to the trolly in a direction opposite to the trolly's motion and jumps out of the trolly. How much has teh trolly moved from the time the child begins to run ?
To solve the problem step by step, we will follow these calculations:
Step 1: Convert the speed of the trolley from km/h to m/s
The speed of the trolley is given as 36 km/h. We can convert this to meters per second using the conversion factor 518.
Speed of trolley=36km/h×518=10m/s
Step 2: Determine the initial momentum of the system
The initial momentum Pi of the system (trolley + child) can be calculated using the formula:
Pi=(mtrolley+mchild)×vtrolley
Where:
- mtrolley=200kg
- mchild=20kg
- vtrolley=10m/s
Calculating the initial momentum:
Pi=(200+20)×10=2200kg m/s
Step 3: Set up the final momentum equation
When the child runs on the trolley, the final momentum Pf can be expressed as:
Pf=mtrolley×vtrolley final+mchild×vchild final
Let vtrolley final=V and the child's speed relative to the ground when he jumps off is V−4m/s (since he runs opposite to the trolley's motion).
Thus, we can write:
Pf=200V+20(V−4)
Step 4: Apply the conservation of momentum
According to the conservation of momentum, Pi=Pf:
2200=200V+20(V−4)
Expanding and simplifying:
2200=200V+20V−80
2200+80=220V
2280=220V
V=2280220=10.36m/s
Step 5: Calculate the time taken by the child to run across the trolley
The child runs a distance of 10 meters at a speed of 4 m/s relative to the trolley:
Time=DistanceSpeed=10m4m/s=2.5s
Step 6: Calculate the distance moved by the trolley during this time
Now, we can find out how far the trolley moves in the time the child runs:
Distance moved by trolley=vtrolley final×Time
Distance=10.36m/s×2.5s=25.9m
Final Answer
The trolley has moved 25.9 meters from the time the child begins to run.
---
The speed of the trolley is given as 36 km/h. We can convert this to meters per second using the conversion factor 518.
The initial momentum Pi of the system (trolley + child) can be calculated using the formula:
- mtrolley=200kg
- mchild=20kg
- vtrolley=10m/s
When the child runs on the trolley, the final momentum Pf can be expressed as:
According to the conservation of momentum, Pi=Pf:
2200+80=220V
2280=220V
V=2280220=10.36m/s
The child runs a distance of 10 meters at a speed of 4 m/s relative to the trolley:
Now, we can find out how far the trolley moves in the time the child runs:
Distance=10.36m/s×2.5s=25.9m
The trolley has moved 25.9 meters from the time the child begins to run.
Topper's Solved these Questions
Similar Questions
A body of mass 10 kg moving at a height of 2 m, with uniform speed of 2 m/s. Its total energy is
View SolutionA trolley of mass 300 ks carrying a sand bag of 25 kg is moving uniformly with a speed of 27km/h on a frictionless track. After a while, sand starts leaking out of a hole on the trolley's floor at the rate of 0.05kgs−1. What is the speed of the trolley after the entire sand bag is empty ?
View SolutionA man of mass m moves with a constant speed on a plank of mass M and length l kept initially at rest on a frictionless horizontal surface, from one end to the other in time t. The speed of the plank relative to grounud while man is moving, is
View SolutionA man of mass m moves with a constant speed on a plank of mass M and length L kept initially at rest on a frictionless horizontal surface . From one end to the other he reaches in time t . The speed of the plank relative to ground while man is moving , is
View SolutionA girl of mass 25 kg running with a speed of 1⋅5ms jumps on a skateboard of mass 2 kg kept on a surface . What will be their combined velocity ?
View SolutionA girl of mass 25 kg running with a speed of 1.5 m/s jumps on a skateboard of mass 2 kg kept on a smooth surface. What will be their combined velocity?
View SolutionA cart A of mass 50 kg moving at a speed of 20 km/h hits a lighter cart B of mass 20 kg moving towards it at a speed of 10 km/h. The two carts cling to each other. Find the speed of the combined mass after the collision.
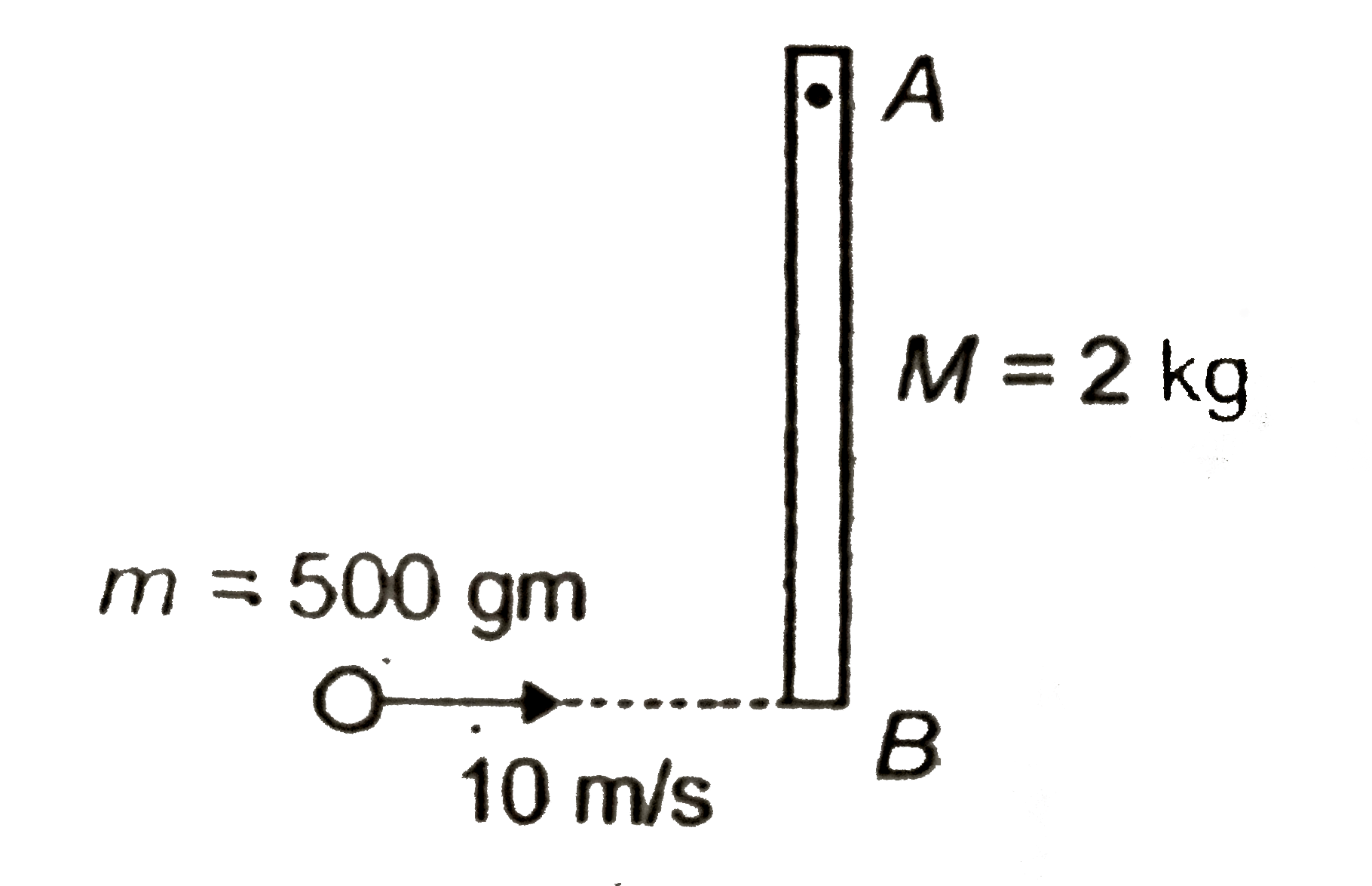
View SolutionA rod of mass 2kg and length 1m is pivoted at one end A and kept on a smooth surface. A particle of mass 500gmstrikestheotherendB of the rod and sticks to the rod. If particle was moving with the speed of 10m/s, what is the angular speed of the rod after collision ?
View SolutionA particle of mass 2 kg moving with a speed of 6 m/s collides elastically with another particle of mass 4 kg travelling in same direction with a speed of 2m/s. The maximum possible deflection of the 2 kg particle is
View SolutionA stone of mass m1 moving at uniform speed v suddenly explodes into two fragments. If the fragment of mass m2 is at rest, the speed of the other fragment is
View Solution