A Cassegrainian telescope uses two mirrors as shown in Fig. Such a telescope is built with the mirrors 20mm apart. If the radius of curvature of large mirror is 220mm and the small mirror is 40mm, where will the final image of an object at infinity be ?
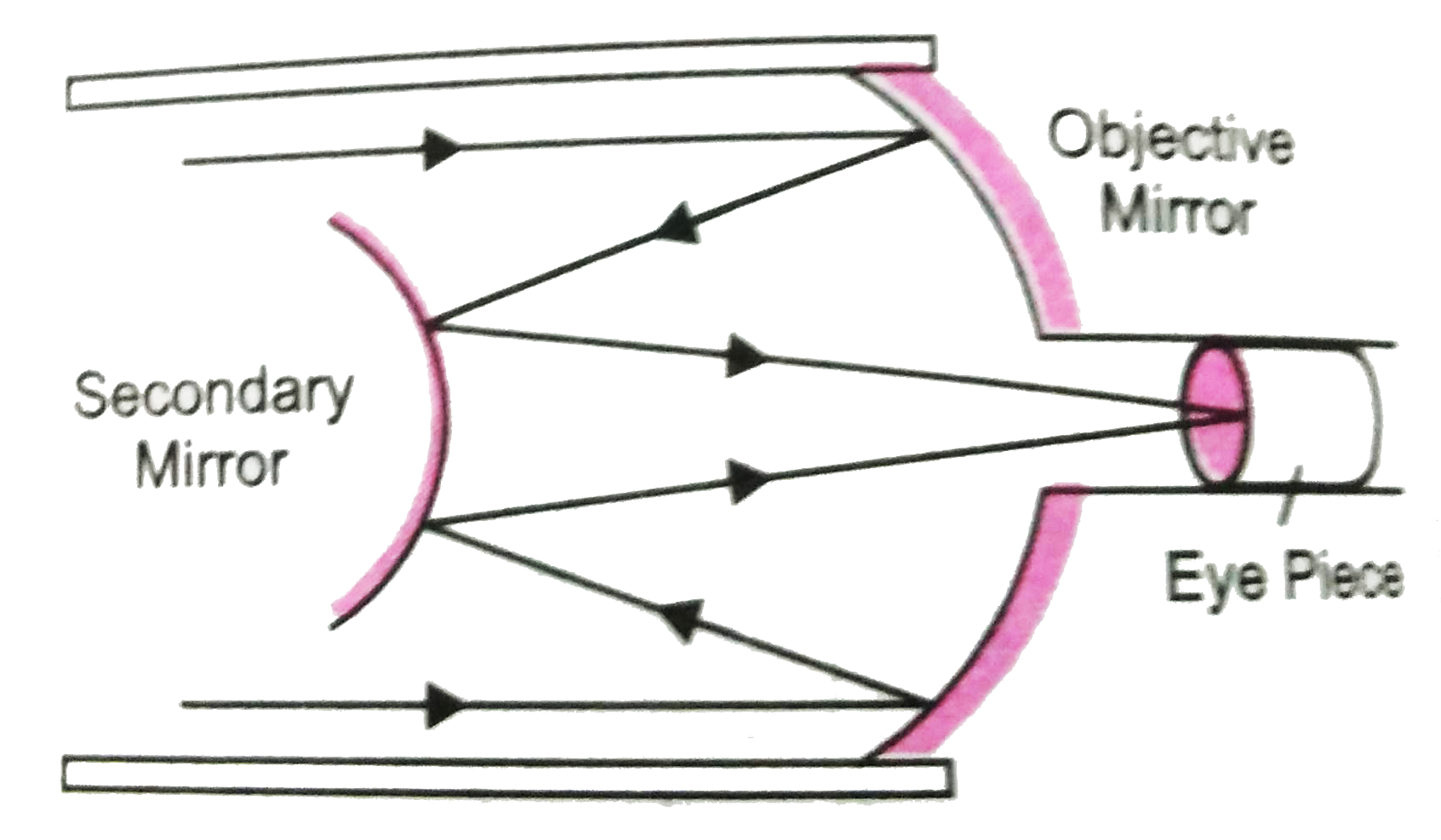
The following figure shows a Cassegrain telescope consisting of a concave mirror and a convex mirror.
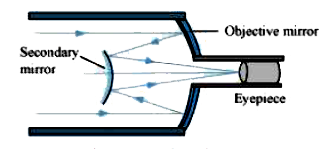 Distance between the objective mirror and the secondary mirror, d=20mm Radius of curvature of the secondary mirror, R1=140mm
Distance between the objective mirror and the secondary mirror, d=20mm Radius of curvature of the secondary mirror, R1=140mm
Hence, focal length of the secondary mirror, f2=R22=1402=70mm
The image of an object placed at infity, formed by the objective mirror, will act as a virtual object for the secondary mirror. Hence, the virtual object distance for the secondary mirror, μ=f1−d
110−20=90mm
Applying the mirror formula for the secondary mirror, we can calculate image distance (v) as
1v+1u=1f2
1v=1f2−1u
−170−190=9−7630=2630
∴v=6302=315mm
Hence, the final image will be formed 315 mm away from the secondary mirror.
Topper's Solved these Questions
- RAY OPTICS AND OPTICAL INSTRUMENTSBOOK - NCERT ENGLISHCHAPTER - RAY OPTICS AND OPTICAL INSTRUMENTSEXERCISE - Exercise
32 Videos
- NUCLEIBOOK - NCERT ENGLISHCHAPTER - NUCLEIEXERCISE - Exercise
31 Videos
- SEMICONDUCTOR ELECTRONICS MATERIALS DEVICES AND SIMPLE CIRCUITSBOOK - NCERT ENGLISHCHAPTER - SEMICONDUCTOR ELECTRONICS MATERIALS DEVICES AND SIMPLE CIRCUITSEXERCISE - Exercise
15 Videos
Similar Questions
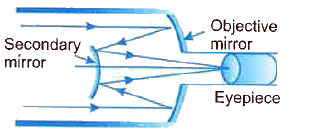
A Cassegrain telescope uses two mirrors as shown in Fig. 9.10. Such a telescope is built with the mirrors 20 mm apart. If the radius of curvature of the large mirror is 220 mm and the small mirror is 140 mm, where will the final image of an object at infinity be ?
View SolutionThe radius of curvature of a concave mirror is 50 cm. Where should an object be held from the mirror so as to form its image at infinity?
View Solution
Knowledge Check
A convex mirror forms a real image 5 times the size of the object placed at a distance of 20cm from it. The radius of curvature of the mirror is
A1003cmB503cmC100cmD50cmAn object is 100 mm in front of a concave mirror which produces an upright image (erect image). The radius of curvature of the mirror is :
Aless than 100 mmBbetween 100 mm and 200 mmCexactly 200 mmDmore than 200 mmThe radius of curvature of a convex mirror is 'x'. The distance of an object from focus of this mirror is 'y'. Then what is the distance of image from the focus ?
Ay24xBx2yCx24yD4y2x
The radius of curvature of a concave mirror is 50 cm. Where should an object be placed from the mirror so as to form its image at infinity ?
View SolutionIn a Cassegrainian telescope, ……………………. is a large concave mirror.
View Solution
An object kept on the principle axis is moving in the sme directions as that of mirror as shown in the figure. Speed of object and mirror is 10ms and 4013ms. Radius of the curvature of the mirror is 20 cm. If the distance of object from the mirror at this instant is 5x cm, velocity of image at this instant is found to be zero. Find x.
View Solution
An object kept on the principle axis is moving in the sme directions as that of mirror as shown in the figure. Speed of object and mirror is 10ms and 4013ms. Radius of the curvature of the mirror is 20 cm. If the distance of object from the mirror at this instant is 5x cm, velocity of image at this instant is found to be zero. Find x.
View SolutionAn object is placed at a large distance in front of a convex mirror of radius of curvature 40 cm. How far is the image behind the mirror?
View Solution
