Figure8-5a shows two industrial spies sliding an initially stationary 225 kg floor safe a displacement →d of magnitude 8.50 m. The push →F1 of spy 001 is 12.0 N at an angle of 30.0∘ downward from the horizontal, the pull →F2 of spy 002 is 10.0 N at 40.0∘ above the horizontal. The magnitudes and directions of these forces do not change as the safe moves, and the floor and safe make frictionless contact.
(a) What is the net work done on the safe by forces →F1 and →F2 during the displacement →d ?
KEY IDEAS
the net work W done on the safe by the two forces is the sum of the works they do individually. (2) Because we can treat the safe as a particle and the forces are constant in both magnitude and direction, we can use either Eq. 8−7(W=Fdcosϕ)or Eq.8−8(W=→F⋅→d) to calculate those works. Let.s choose Eq. 8-7.
Calculations: From Eq. 8-7 and the free-body diagram for the safe in Fig. 8-5b, the work done by →F1 is
W1=F1dcosϕ1=(12.0N)(8.50m)(cos30.0∘)
=88.33J,
and the work done by →F2 is
W2=F2dcosϕ2=(10.0N)(8.50m)(cos40.0∘)
=65.11J.
Thus, the net work W is
W=W1+W2=88.33J+65.11J
=153.4J≈153J.
During the 8.50 m displacement, therefore, the spies transfer 153 J of energy to the kinetic energy of the safe.
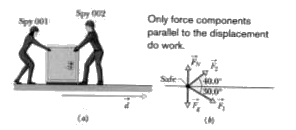
Figure 8-5 (a) Two spies move a floor safe through a displacement →d (b) A free-body diagram for the safe.
Topper's Solved these Questions
- WORK, POWER, AND ENERGYBOOK - RESNICK AND HALLIDAYCHAPTER - WORK, POWER, AND ENERGYEXERCISE - CHECKPOINT
6 Videos
- WORK, POWER, AND ENERGYBOOK - RESNICK AND HALLIDAYCHAPTER - WORK, POWER, AND ENERGYEXERCISE - PROBLEMS
58 Videos
- WAVES-IBOOK - RESNICK AND HALLIDAYCHAPTER - WAVES-IEXERCISE - Practice Questions (Integer Type)
4 Videos
Similar Questions
Figure8-5a shows two industrial spies sliding an initially stationary 225 kg floor safe a displacement →d of magnitude 8.50 m. The push →F1 of spy 001 is 12.0 N at an angle of 30.0∘ downward from the horizontal, the pull →F2 of spy 002 is 10.0 N at 40.0∘ above the horizontal. The magnitudes and directions of these forces do not change as the safe moves, and the floor and safe make frictionless contact.
(b) During the displacement, what is the work Wg done on the safe by the gravitational force →Fg and what is the work WN done on the safe by the normal force →FN from the floor ?
View SolutionFigure8-5a shows two industrial spies sliding an initially stationary 225 kg floor safe a displacement →d of magnitude 8.50 m. The push →F1 of spy 001 is 12.0 N at an angle of 30.0∘ downward from the horizontal, the pull →F2 of spy 002 is 10.0 N at 40.0∘ above the horizontal. The magnitudes and directions of these forces do not change as the safe moves, and the floor and safe make frictionless contact.
The safe is initially stationary. What is its speed vf at the end of the 8.50 m displacement?
View Solution
Knowledge Check
A force →F1 of magnitude 9 N makes an angle of 30∘ with the X-axis. Another force →F2 of magnitude 12 N, makes an angle of 120∘ with the X-axis. What is the magnitude of the resultant force ?
A7.5 NB10 NC12.5 ND15 NA boy pushes a toy box 2.0 m along the floor by means of a force of 10 N directed downward at an angle of 60∘ to the horizontal.The work done by the boy is
A6JB8JC10JD12JA 1.00×102 kg crate is being pushed across a horizontal floor by a force →P that makes an angle of 30.0∘ below the horizontal. The coefficient of kinetic friction is 0.200. What should be the magnitude of →P, so that the net work done by it and the kinetic frictional force is zero ?
A256NB354NC203ND287N
The vector →F is a force of 3.0 newton making an angle of 60∘ with the displacement . →R of magnitude 4.0 m , find
the magnitude and direction of the cross product →R×→F

View SolutionA person pushes horizontally with a force of 220 N on a 55 kg crate to move it across a level floor. The coefficient of kinetic friction between the crate and the floor is 0.35. What is the magnitude of (a) the frictional force?
View SolutionFind magnitude and direction of →F2−→F1 if →F1=500N due east and →F2=250N due north:
View SolutionA 3.5 kg block is pushed along a horizontal floor by a force →F of magnitude 15 N at an angle θ=40∘ with the horizontal (Fig. 6-33). The coefficient of kinetic friction between the block and the floor is 0.25. Calculate the magnitudes of (a) the frictional force on the block from the floor and (b) the block's acceleration.

View SolutionA horizontal force F pulls a 20 kg box at a constant speed along a rough horizotal floor. The coefficient of friction between the box and the floor is 0.25. The work done by force F on the block in displacing it by 2 m is
View Solution
