A 1500-kg elevator moves upward with constant speed through a vertical distance of 25 m. How much work was done by the tension in the elevator cable ?
990J
8100J
140,000J
370,000J
The correct Answer is:D
To solve the problem of how much work was done by the tension in the elevator cable, we can follow these steps:
Step 1: Identify the given data
- Mass of the elevator (m) = 1500 kg
- Distance moved upward (d) = 25 m
- Acceleration due to gravity (g) = 10 m/s² (or 9.8 m/s² if more precision is needed)
Step 2: Calculate the weight of the elevator
The weight (W) of the elevator can be calculated using the formula:
W=m⋅g
Substituting the values:
W=1500kg⋅10m/s2=15000N
Step 3: Determine the tension in the cable
Since the elevator is moving upward at a constant speed, the tension (T) in the cable must equal the weight of the elevator:
T=W=15000N
Step 4: Calculate the work done by the tension
Work (W) done by the tension can be calculated using the formula:
W=F⋅d⋅cos(θ)
Where:
- F is the force (tension in this case)
- d is the distance moved
- θ is the angle between the force and the direction of motion. Since both the tension and the displacement are in the same direction (upward), θ=0° and cos(0°)=1.
Thus, the work done by the tension is:
W=T⋅d⋅cos(0°)
Substituting the values:
W=15000N⋅25m⋅1
W=375000J
Final Answer
The work done by the tension in the elevator cable is 375000 Joules.
---
- Mass of the elevator (m) = 1500 kg
- Distance moved upward (d) = 25 m
- Acceleration due to gravity (g) = 10 m/s² (or 9.8 m/s² if more precision is needed)
The weight (W) of the elevator can be calculated using the formula:
W=m⋅g
Substituting the values:
W=1500kg⋅10m/s2=15000N
Since the elevator is moving upward at a constant speed, the tension (T) in the cable must equal the weight of the elevator:
T=W=15000N
Work (W) done by the tension can be calculated using the formula:
W=F⋅d⋅cos(θ)
Where:
- F is the force (tension in this case)
- d is the distance moved
- θ is the angle between the force and the direction of motion. Since both the tension and the displacement are in the same direction (upward), θ=0° and cos(0°)=1.
W=T⋅d⋅cos(0°)
Substituting the values:
W=15000N⋅25m⋅1
W=375000J
The work done by the tension in the elevator cable is 375000 Joules.
Topper's Solved these Questions
- BOOK - RESNICK AND HALLIDAYCHAPTER - WORK, POWER, AND ENERGYEXERCISE - PRACTICE QUESTIONS (More than one choice correct type)10videos
WORK, POWER, AND ENERGY
- BOOK - RESNICK AND HALLIDAYCHAPTER - WORK, POWER, AND ENERGYEXERCISE - PRACTICE QUESTIONS (Linked Comprehension)9videos
WORK, POWER, AND ENERGY
- BOOK - RESNICK AND HALLIDAYCHAPTER - WORK, POWER, AND ENERGYEXERCISE - PROBLEMS58videos
WORK, POWER, AND ENERGY
- BOOK - RESNICK AND HALLIDAYCHAPTER - WAVES-IEXERCISE - Practice Questions (Integer Type)4videos
WAVES-I
Similar Questions
The total mass of an elevator with a 80 kg man in it is 1000 kg. This elevator moving upward with a speed of 8 m/sec, is brought to rest over a distance of 16m. The tension T in the cables supporting the elevator and the force exerted on the man by the elevator floor will respectively be-
View SolutionAn elevator has mass of 600 kg, not including passengers The elevator is designed to ascend at constant speed a vertical distance of 20 m in 15 sec. It is driven by a motor that can provide up to 30 hp to the elevator, what is the maximum number of passengers that can ride in the elevator ? Assume that an average passenger has a mass of 65 kg
View Solution
Knowledge Check
- Question 1 - Select One
A force of magnitude 25 N directed at an angle of 37∘ above the horizontal moves a 10-kg crate along a horiaontal surface at constant velocity . How much work is done by this force in moving the crate a distance of 15 m ?
A0JB40JC300JD98J - Question 2 - Select One
In Q 63 above , if the elevator moves upward with a constant acceleration , the apparent weight of the person is
Aless than his true weightBequal to his true weightCmore or less than his true weightDmore or less than his true weight
A ski lift elevates a skier of total mass 70 kg a vertical displacement of 300 m.on ski slope.
What work is done to elevate the skier ?
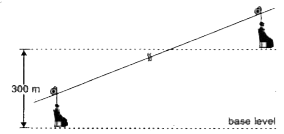 View Solution
View SolutionConsider an elevator in a hospital. The elevator accelerates upward with constant acceleration for a distance of 100 cm and then starts to slow down. Also, it is known that the force exerted on a passenger by the floor of the elevator does not exceed 1. 8 times the passenger's weight. Now, find the maximum speed of the elevator in SI units: Take g=10ms−2
View SolutionYou are designing an elevator for a hospital. The force exerted on a passenger by the floor of the elevator is not to exceed 1.60 times the passenger's weight. The elevator accelerates upwards with constant acceleration for a distance of 3.0 m and then starts to slow down. What is the maximum speed (in ms−1) of the elevator?
View SolutionA factory worker pushes a 25.0 kg crate downward at an angle of 30∘ below the horizontal a distance of 6.0 m along a level floor.
(a) What magnitude of force must the worker apply to move the crate at constant velocity, if the coefficient of kinetic friction between the crate and floor is 0.3 ?
(b) How much work is done on the crate by this force when the crate is pushed a distance of 6.0 m?
(c) How much work is done on the crate by friction during this displacement ?
(d) How much work is done by the normal force and by gracity ?
(e) What is the total work done on the crate ? Take g=10m/s2View SolutionA girl having mass of 35 kg sits on a trolley of mass 5 kg. The trolley is given an initial velocity of 4m//s by applying a force. The trolley comes to rest after traversig a distance of 16m. (a) How much work is done on the trolley ? (b) How much work is done by the girl ?
View SolutionA 1500 kg cable car moves vertically by means of a cable that connects the ground and the top of a hill. What is the tension in the supporting cable when the cab, originally moving downard at a speed of 9.0 m/s, is brought to rest with constant acceleration in a distance of 38 m.
View Solution

