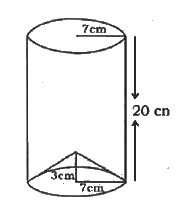
The bottom of a right cylindrical shaped vessel made from metallic sheet is dosed by a cone shaped vessel as r shown in the figure. The radius of the circular base of the cylinder and radius of the circular base of the cone are each is equal to 7 cm. If t~e height of the cylinder is 20 cm and height of cone is 3 cm, calculate the cost of milk to fill completely this vessel at the rate of Rs. 20 per litre.

OR
A hemispherical vessel of radius 14 cm is fully filled with sand . This sand is poured on a level ground . The heap of sand forms a cone shape of height 7 cm . Calculate the area of ground occupied by the circular base of the heap of the sand . Solution in Kannada
Step by step video solution for The bottom of a right cylindrical shaped vessel made from metallic sheet is dosed by a cone shaped vessel as r shown in the figure. The radius of the circular base of the cylinder and radius of the circular base of the cone are each is equal to 7 cm. If t~e height of the cylinder is 20 cm and height of cone is 3 cm, calculate the cost of milk to fill completely this vessel at the rate of Rs. 20 per litre. OR A hemispherical vessel of radius 14 cm is fully filled with sand . This sand is poured on a level ground . The heap of sand forms a cone shape of height 7 cm . Calculate the area of ground occupied by the circular base of the heap of the sand . by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
- SOLVED PAPER (SSLC KARNATAKA APRIL 2019)BOOK - OSWAAL PUBLICATIONCHAPTER - SOLVED PAPER (SSLC KARNATAKA APRIL 2019)EXERCISE - Answer the following :
29 Videos
- REAL NUMBERSBOOK - OSWAAL PUBLICATIONCHAPTER - REAL NUMBERSEXERCISE - TEXTBOOK CORNER ( EXERCISE 8.4 )
12 Videos
- SOLVED PAPER SSLC KARNATAKA JUNE 2020 BOOK - OSWAAL PUBLICATIONCHAPTER - SOLVED PAPER SSLC KARNATAKA JUNE 2020 EXERCISE - Answer the following questions
37 Videos
Similar Questions
The given graph represents a pair of linear equations in two variables. Write how many solutions these pair of equations have.

View SolutionWithout actually performing the long division state whether the following rational numbers will have a terminating decimal expansion or a non-terminating repeating decimal expansion
133125
View SolutionExpress the denominator of 23/20 the form of 2n×5m and state whether the given fraction is terminating or non-terminating repeating decimal.
View SolutionA metallic cylinder of radius 8 cm and height 2 cm is melted and converted into a right circular cone of height 6 cm . The radius of the base of this cone is
View SolutionA right circular cone is made by melting a piece of metal right cylinder of radius 8 cm and height 2 cm . If the height of the cone is 3 times the height of the cylinder , then what will be the curved surface area of the cone ?
View SolutionThe material of a cone is covered into the shape of a cylinder of equal radius. If the height of the cylinder is 5 cm. then height of the cone is ___.
View SolutionThe bottom of a right cylindrical shaped vessel made from metallic sheet is closed by a cone shaped vessel as shown in the figure . The radius of the circular base of the cylinder and radius of the circular base of the cone each os equal to 7 cm . If the height of the cylinder is 20 cm and height of cone is 3 cm calculate the cost of milk to fill completely this vessel at the rate of Rs. 20 per litre.
View SolutionThe material of a cone is covered into the shape of a cylinder of equal radius. If the height of the cylinder is 5 cm. then height of the cone is ___.
View SolutionThe material of a cone is covered into the shape of a cylinder of equal radius. If the height of the cylinder is 8 cm. then height of the cone is ___.
View SolutionThe material of a cone is covered into the shape of a cylinder of equal radius. If the height of the cylinder is 10 cm. then height of the cone is ___.
View SolutionThe material of a cone is covered into the shape of a cylinder of equal radius. If the height of the cylinder is 5 cm. then height of the cone is ___.
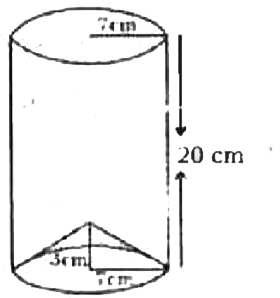
View SolutionThe bottom of a light cylindrical shaped vessel made from metallic sheet is closed by a cone shaped vessel as shown in the figure. The radius of the circular base of the cylinder and radius of the circular base of the cone each is equal to 7 cm . If the height of the cylinder is 20 cm & height of the cone is 3 cm, calculate the cost of milk of fill completely this vessel at the rate of Rs. 20/liter.
View Solutionधातु के बने किसी ठोस शंकु को पिघलाकर एक ठोस बेलन बनाया गया जिसका वृत्तीय आधार शंकु के वृत्तीय आधार के बराबर है। यदि बेलन की ऊँचाई 7 cm हो, तो शंकु की ऊँचाई ज्ञात कीजिए।
View SolutionThe bottom of a straight cylinder figure vessel made of metal foil is completely covered with a cone shaped metal container as shown in the picture. The radius of the circular foot of the cylinder and the radius of the circular foot of the cone are equal to 7 cm. The height of the cylinder is 20 cm. And the height of the cone is 3 cm. Is. A liter of milk can be made available in this container for Rs. Find out how much money you need as a 20.
View SolutionThe material of a cone is converted into the shape of a cylinder of equal radius. If height of the cylinder is 5 cm, then height of the cone is____
View Solutionलम्ब वृत्ताकार शंकु का आयतन लम्ब वृत्ताकार बेलन के आयतन के बराबर है। बेलन की ऊँचाई और त्रिज्या क्रमश: 9 सेमी. और 20 सेमी. हैं। यदि शंकु की ऊँचाई 108 सेमी. है, तो उसकी त्रिज्या कितनी (सेमी में) होगी ?
View Solution
