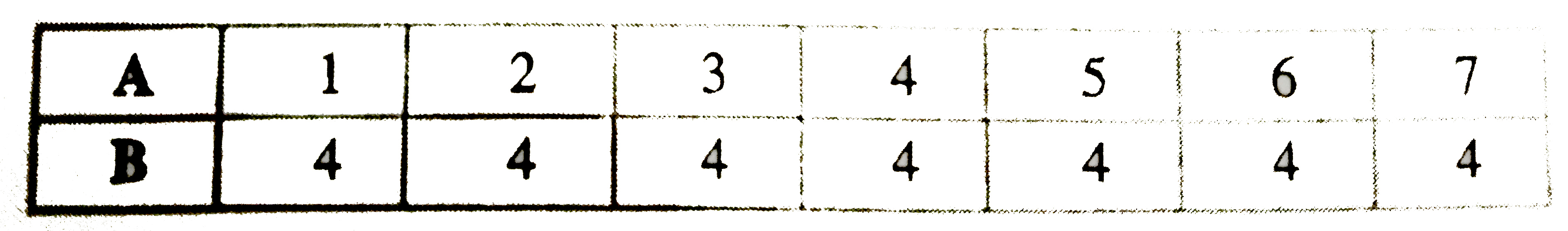The coefficient of correlation when coefficients of regression are 0.2 and 1.8 is
0.36
0.2
0.6
0.9
The correct Answer is:C
To find the coefficient of correlation (r) when the coefficients of regression are given as 0.2 and 1.8, we can follow these steps:
Step 1: Understand the relationship between regression coefficients and correlation coefficient
The coefficient of correlation (r) can be calculated using the formula:
r=√bxy⋅byx
where bxy is the regression coefficient of Y on X and byx is the regression coefficient of X on Y.
Step 2: Identify the given regression coefficients
From the question, we have:
- bxy=0.2
- byx=1.8
Step 3: Substitute the values into the formula
Now we substitute the values into the correlation formula:
r=√0.2⋅1.8
Step 4: Calculate the product
First, we calculate the product:
0.2⋅1.8=0.36
Step 5: Take the square root
Now, we take the square root of the product:
r=√0.36=0.6
Step 6: Determine the sign of the correlation coefficient
Since both regression coefficients (0.2 and 1.8) are positive, the correlation coefficient will also be positive. Thus, we conclude:
r=0.6
Final Answer
The coefficient of correlation is r=0.6.
---
The coefficient of correlation (r) can be calculated using the formula:
r=√bxy⋅byx
where bxy is the regression coefficient of Y on X and byx is the regression coefficient of X on Y.
From the question, we have:
- bxy=0.2
- byx=1.8
Now we substitute the values into the correlation formula:
r=√0.2⋅1.8
First, we calculate the product:
0.2⋅1.8=0.36
Now, we take the square root of the product:
r=√0.36=0.6
Since both regression coefficients (0.2 and 1.8) are positive, the correlation coefficient will also be positive. Thus, we conclude:
r=0.6
The coefficient of correlation is r=0.6.
Topper's Solved these Questions
- SETS, RELATIONS, FUNCTIONS AND NUMBER SYSTEM BOOK - NDA PREVIOUS YEARSCHAPTER - SETS, RELATIONS, FUNCTIONS AND NUMBER SYSTEM EXERCISE - MCQ
271 Videos
- TRIGONOMETRY - RATIO & IDENTITY , TRIGONOMETRIC EQUATIONSBOOK - NDA PREVIOUS YEARSCHAPTER - TRIGONOMETRY - RATIO & IDENTITY , TRIGONOMETRIC EQUATIONSEXERCISE - MCQ
238 Videos
Similar Questions
Knowledge Check
The coefficient of correlation is independent of
Achange of scale onlyBchange of origin onlyCboth change of scale and change of originDneither change of scale nor change or originBOD is measure of
ACentral tendencyBDispersionCBoth central tendency and dispersionDNeither central tendency nor dispersion.Coefficient of correlation is the measure of
Acentral tendencyBdispresionCboth central tendency and dispersionDneither central tendency nor dispersion
Calculate coefficient of correlation between series X and Y.

View SolutionSpearmans Rank Correlation Coefficient
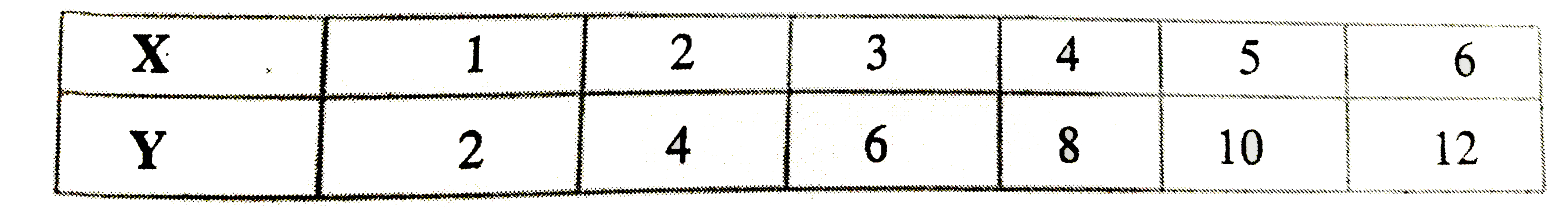
View SolutionCalculate the coefficient of correlation between the two series :
View SolutionIf the regression coefficient of Yon X is-6 and the correlation coefficient between X and Y is -1/2. then the regression coefficient od X on Y would be
View SolutionX and Y are two correlated variables with the same standard deviation and the correlation coefficient r, the correlation coefficient between X and X+Y is
View Solution