If the correlation coefficient between x and y is 0.6, covariance is 27 and variance of y is 25, then what is the variance of x?
95
8125
9
81
The correct Answer is:D
To find the variance of x given the correlation coefficient, covariance, and variance of y, we can use the following relationship:
1. Given Data:
- Correlation coefficient r=0.6
- Covariance Cov(x,y)=27
- Variance of y Var(y)=25
2. Formula for Correlation Coefficient:
The correlation coefficient r can be expressed in terms of covariance and standard deviations:
r=Cov(x,y)σxσy
where σx is the standard deviation of x and σy is the standard deviation of y.
3. Calculate Standard Deviation of y:
The standard deviation σy is the square root of the variance of y:
σy=√Var(y)=√25=5
4. Substituting Values into the Correlation Formula:
Now, substituting the known values into the correlation formula:
0.6=27σx⋅5
5. Rearranging to Find σx:
Rearranging the equation to solve for σx:
σx⋅5=270.6
σx=270.6⋅5
σx=273=9
6. Calculating Variance of x:
The variance of x is the square of the standard deviation:
Var(x)=σ2x=92=81
7. Final Answer:
Therefore, the variance of x is 81.
- Correlation coefficient r=0.6
- Covariance Cov(x,y)=27
- Variance of y Var(y)=25
The correlation coefficient r can be expressed in terms of covariance and standard deviations:
r=Cov(x,y)σxσy
where σx is the standard deviation of x and σy is the standard deviation of y.
The standard deviation σy is the square root of the variance of y:
σy=√Var(y)=√25=5
Now, substituting the known values into the correlation formula:
0.6=27σx⋅5
Rearranging the equation to solve for σx:
σx⋅5=270.6
σx=270.6⋅5
σx=273=9
The variance of x is the square of the standard deviation:
Var(x)=σ2x=92=81
Therefore, the variance of x is 81.
Topper's Solved these Questions
- SETS, RELATIONS, FUNCTIONS AND NUMBER SYSTEM BOOK - NDA PREVIOUS YEARSCHAPTER - SETS, RELATIONS, FUNCTIONS AND NUMBER SYSTEM EXERCISE - MCQ
271 Videos
- TRIGONOMETRY - RATIO & IDENTITY , TRIGONOMETRIC EQUATIONSBOOK - NDA PREVIOUS YEARSCHAPTER - TRIGONOMETRY - RATIO & IDENTITY , TRIGONOMETRIC EQUATIONSEXERCISE - MCQ
238 Videos
Similar Questions
Knowledge Check
If the coefficient of correlation between x and y os 0.6, covariance is 27 and variance of y is 25, then what is the variance x?
A9/5B81/25C9D81If the covariance between x and y is 30, variance of x is 25 and variance of y is 144, then what is the correlation coefficient?
A0.4B0.5C0.6D0.7If the covariance between x and y is 30. variance of A is 25 and variance of y is 144, then what is the correlation coefficient?
A0.4B0.5C0.6D0.7
If AM and coefficient of variation of x is 10 and 40 then what is the variance of (15−2x)
View SolutionLet the correlation coefficient between X and Y be 0.6, random variable Z and W are defined as Z=X+5 and W=y3 What is the correlation coefficient between Z and W
View SolutionLet the correlation coefficient between X and Y be 0.6. Random variables Z and W are defined as Z=X+5andW=Y3. What is the correlation coefficient between Z and W?
View Solution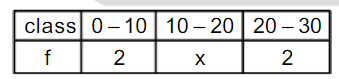
If variance of variable is 50 then x=
View SolutionThe coefficient of correlation between random variables X and Y is 0.28, covariance between X and Y is 7.6 and the variance of X is 9, then the standard deviation of Y series is
View Solution
