Obtain the formula for the electric field due to a along thin wire of uniform linear charge density λ without using Gauss's law.
Consider a long thin wire of length L and having uniform charge density λ. Let P be a point situated at a normal distance r from the wire. Let there be a small length elements situated at a distance y and having length dy as shown in According to Coulomb.s law electric field due to this element at point P has a magnitude.
dE=14π∈0.dq(r2+y2)=14π∈0.λdy(r2+y2)
−→dE may be resolved into 2 components :(i) a component dEx in a direction normal to wire, and (ii) component dEy in a direction parallwl to the wire. Obviously if we find electric field due to whole wire ,then ∑dEy=0 because for every at +y there is corresponding element at -y and
∴ Electric field due to whole conductor at point?
E=∫dEx=∫dEsinθ=λ4π∈0∫dysinθ(r2+y2)
But y=θ√,hence dy =−rcosec2θdθ
∴ E=λ4π∈0−−−→θ=0θ=π∫(r−cosec2θxdθ)sinθ(r2+r2cot2θ)=λ4π∈00∫π−1rsinθdθ
=λ4π∈0[cosθ]0=πλ4π∈0[cos0∘−cosπ]
=λ4π∈0[1−(−1)]=λ2π∈0r
(##U_LIK_SP_PHY_XII_C01_E02_006_S01.png" width="80%">
Topper's Solved these Questions
- ELECTRIC CHARGES AND FIELDS BOOK - U-LIKE SERIESCHAPTER - ELECTRIC CHARGES AND FIELDS EXERCISE - CASE BASED/ SOURCE -BASED INTEGRATED QUESTIONS
14 Videos
- ELECTRIC CHARGES AND FIELDS BOOK - U-LIKE SERIESCHAPTER - ELECTRIC CHARGES AND FIELDS EXERCISE - MULTIPLE CHOICE QUESTIONS
30 Videos
- ELECTRIC CHARGES AND FIELDS BOOK - U-LIKE SERIESCHAPTER - ELECTRIC CHARGES AND FIELDS EXERCISE - SELF ASSESSMENT TEST (SECTION-B) (VERY SHORT ANSWER QUESTIONS)
4 Videos
- DUAL NATURE OF RADIATION AND MATTERBOOK - U-LIKE SERIESCHAPTER - DUAL NATURE OF RADIATION AND MATTEREXERCISE - SELF ASSESSMENT TEST SECTION-C (VERY SHORT ANSWER QUESTIONS)
3 Videos
- ELECTROMAGNETIC WAVESBOOK - U-LIKE SERIESCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - SELF ASSESSMENT TEST (SECTION C)
1 Videos
Similar Questions
Obtain the formula for the electric field due to a long thin wire of uniform linear charge density λ without using Gauss's law. [Hint. use Coulomb's law directly and evaluate the necessary integral].
View SolutionObtain the formula for the electric field due to a long thin wire of uniform linear charge density λ without using Gauss's law. [Hint.use Coulomb's law directly and evaluate the necessary integral ].
View Solution
Knowledge Check
The electric field intensity due to a thin infinity long straight wire of uniform linear charge density λ at O is-
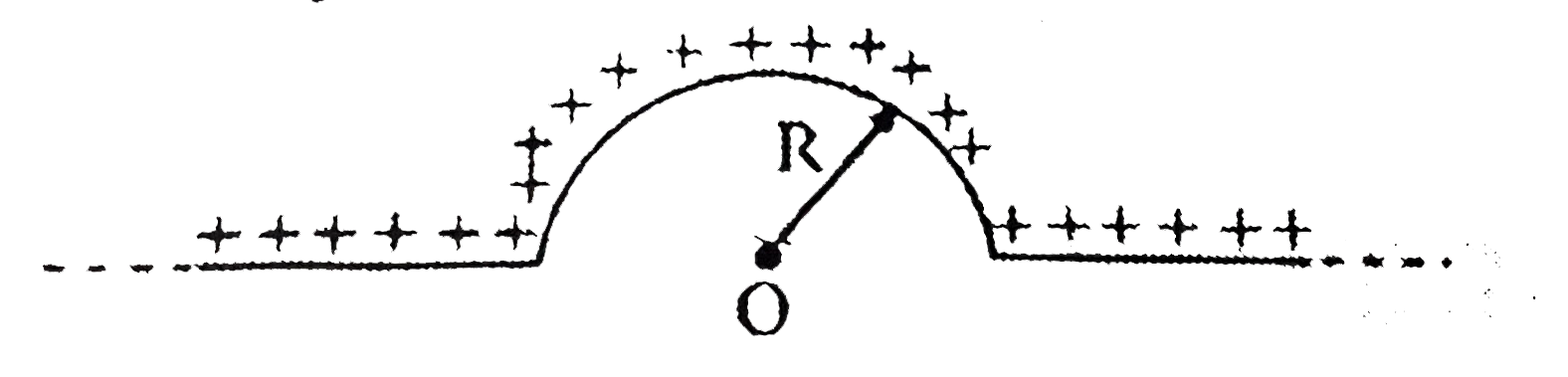 Aλ2πε0RBλ√22πε0RCλ√52πε0RDzero
Aλ2πε0RBλ√22πε0RCλ√52πε0RDzero
State Gauss' law in electrostatic. Using it derive an expression for the electric field due to an infinitely long straight wire of linear charge density λ C/m.
View SolutionState Gauss's law on electrostatics and derive an expression for the electric field due to a long straight thin uniformly charged wire (linear charge density ) at a point lying at a distance r from the wire.
View SolutionState Gauss' law.Using this law obtain the expression for the electric field due to an infinitely long straight conductor of linear charge density λ
(ii) A wire AB of length L has linear charge λ=kx, where x is measured from the end A of the wire. The wire is enclosed by a Gaussian hollow surface.Find the expression for the electric flux through the surface.
View SolutionFind the electric field at the origin due to the line charge (ABCD) of linear charge density λ,

View SolutionState Gauss's law in electrostatics. Use this law to derive an expression for the electric field due to an infinitely long straight wire of linear charge density λcm−1
View SolutionState Gauss's law in electrostatics. Use this law to derive an expression for the electric field due to an infinitely long straight wire of linear charge density λcm−1
View SolutionAnswer the following:
(a) State Gauss' law. Using this law, obtain the expression for the electric field due to an infinitely long straight conductor of linear charge density λ.
(b) A wire AB of length L has linear charge density λ=kx, where x is measured from the end A of the wire.
This wire is enclosed by a Gaussian hollow surface. Find the expression for the electric flux through this surface.
View Solution
