If two particles each of mass $m$ are placed at the two vertices of an equilateral triangle of side $a$ , then the resultant gravitational force on mass $m$ placed at the third vertex is

A

$\frac{\sqrt{3} G m^{2}}{2 r^{2}}$

B

$\frac{\sqrt{3} G m^{2}}{r^{2}}$

C

$\frac{G m^{2}}{r^{2}}$

D

$\frac{G m^{2}}{2 r^{2}}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:B

Gravitational force on particle at A C due to particle at A is

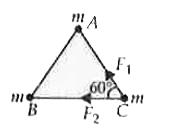
$F_{1} = \frac{G m m}{r^{2}} = \frac{G m^{2}}{r^{2}}$ along CA
Gravitational force on particle at C due to particle at B is
$F_{2} = \frac{G m^{2}}{r^{2}}$ along CB
As ${\to F}_{1}$ and ${\to F}_{2}$ are inclined at an angle $60^{\circ}$ , so the resultant gravitational force on particle at C is
$F = \sqrt{F_{1}^{2} + F_{2}^{2} + 2 F_{1} F_{2} {cos 60}^{\circ}}$
$= \sqrt{F_{1}^{2} + F_{1}^{2} + 2 F_{1} \times F_{1} \times \frac{1}{2}} = \sqrt{3} F_{1} = \frac{\sqrt{3} G m^{2}}{r^{2}} (∵ F_{1} = F_{2})$

|

Updated on:21/07/2023

Class 11 PHYSICS GRAVITATION

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
Four particles each of mass m are placed at the vertices of a square of side $l$ . the potential at the centre of square is
A $- 2 \sqrt{2} \frac{G m}{l}$
B $- 3 \sqrt{2} \frac{G m}{l}$
C $- 2 \frac{G m}{l}$
D $- 4 \sqrt{2} \frac{G m}{l}$
Question 2 - Select One
Three particles each of mass $m$ are palced at the corners of an equilateral triangle of side $b$ . The gravitational potential energy of the system of particle is
A $\frac{- 3 G m^{2}}{2 b}$
B $\frac{- G m^{2}}{2 b}$
C $\frac{- 3 G m^{2}}{b}$
D $\frac{- G m^{2}}{b}$
Question 3 - Select One
Three particles each of mass $m$ are kept at the vertices of an euilateral triangle of side $L$ . The gravitational field at the centre due to these particle is
A $- \frac{3 G M}{L}$
B $- \frac{\sqrt{3} G M}{L}$
C $- \frac{3 \sqrt{3} G M}{L}$
D $- \frac{G M}{3 \sqrt{3} L}$

MTG GUIDE-GRAVITATION-NEET CAFE TOPICWISE PRACTICE QUESTIONS (UNIVERSAL LAW OF GRAVITATION)

Home
Ask Doubt
Profile