Three indentical bodes of mass M are locatd at the verticles of an equailateral triangle of side L they revolve undr the effect of mutual gravitational force in a circular orbit circumscibing the traingle while preserving the equilateral triangle their orbital velocity is

A

$\sqrt{\frac{G M}{L}}$

B

$\sqrt{\frac{3 G M}{2 L}}$

C

$\sqrt{\frac{3 G M}{L}}$

D

$\sqrt{\frac{2 G M}{3 L}}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A

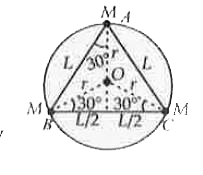
From the figure , $\frac{L}{2} = r {cos 30}^{\circ} = \frac{\sqrt{3}}{2} r or r = \frac{L}{\sqrt{3}}$
The net force of attraction on mass at A due to masses at B and C is
$F_{A} = 2 [\frac{G M^{2}}{L^{2}}] {cos 30}^{\circ} = [\frac{G M^{2}}{L^{2}} \sqrt{3}]$
As this force provides the necessary centripetal force,
$∴ \frac{M v^{2}}{r} = \frac{G M^{2}}{L^{2}} \sqrt{3} or \frac{M v^{2} \sqrt{3}}{L} = \frac{G M^{2} \sqrt{3}}{L^{2}} ∴ v = \sqrt{\frac{G M}{L}}$

|

Updated on:21/07/2023

Class 11 PHYSICS GRAVITATION

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
Three particle each of mass $m$ , are located at the vertices of an equilateral triangle of side a. At what speed must they move if they all revolve under the influence of their gravitational force of attraction in a circular orbit circumscribing the triangle while still preserving the equilateral triangle ?

A $\sqrt{\frac{G m}{a}}$
B $\sqrt{\frac{2 G m}{a}}$
C $\sqrt{\frac{3 G m}{a}}$
D $\sqrt{\frac{4 G m}{a}}$
Question 2 - Select One or More
Three mass ‘m’ each are kept at corner of a equilateral triangle and are rotating under effect of mutual gravitational force -
ARadius of circular path followed by mass is a/2
BVelocity of mass is $\sqrt{\frac{G m}{a}}$
CBinding energy of system is $\frac{1.5 G m^{2}}{a}$
DTime period of mass is $\sqrt{\frac{π a^{3}}{2 G m}}$
Question 3 - Select One
Three particles of equal mass 'm' are situated at the vertices of an equilateral triangle of side $L$ . The work done in increasing the side of the triangle to $2 L$ is
A $\frac{2 G^{2} m}{2 L}$
B $\frac{G m^{2}}{2 L}$
C $\frac{3 G m^{2}}{2} L$
D $\frac{3 G m^{2}}{L}$

MTG GUIDE-GRAVITATION-NEET CAFE TOPICWISE PRACTICE QUESTIONS (UNIVERSAL LAW OF GRAVITATION)

Home
Ask Doubt
Profile