Mass is distributed uniformly over a thin triangular plate and positions of two vertices are given by (1,3) and (2,-4) .What is the position of 3 rd vertex if centre of mass of the plate lies at the origin?
Step by step video & image solution for Mass is distributed uniformly over a thin triangular plate and positions of two vertices are given by (1,3) and (2,-4) .What is the position of 3 rd vertex if centre of mass of the plate lies at the origin? by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Similar Questions
Mass is distributed uniformly over a thin triangular plate and co-ordinates of two vertices are (1m,3m) and (2m,-4m).The co- ordinates of third vertex, if centre of mass of plate lies at origin are
View SolutionWhat is the position vector of centre of mass of two particles of equal masses ?
View Solution
Knowledge Check
- Question 1 - Select One
The coordinates of the positions of particles of mass 7, 4 and 10 gm are (1, 5,-3) , (2, 5, 7) and (3, 3,-1) respectively. Find the position of the center of mass of the system?
A(−157,8517,17)cmB(157,−8517,17)cmC(157,8521,−17)cmD(157,8521,73)cm - Question 2 - Select One
Two particles of masses 1kg and 2kg are located at x=0 and x=3m. Find the position of their centre of mass.
A2m from 1 kgB2m from 2 kgC1m from 1 kgDnone of these - Question 3 - Select One
Mass is non-uniformly distributed over the rod of length l, its linear mass density varies linearly with length as λ=kx2. The position of centre of mass (from lighter end) is given by-
A2l/5B3/lC3l/4D2l/3
Find the position of centre of mass of the T-shaped plate from O, in Fig.
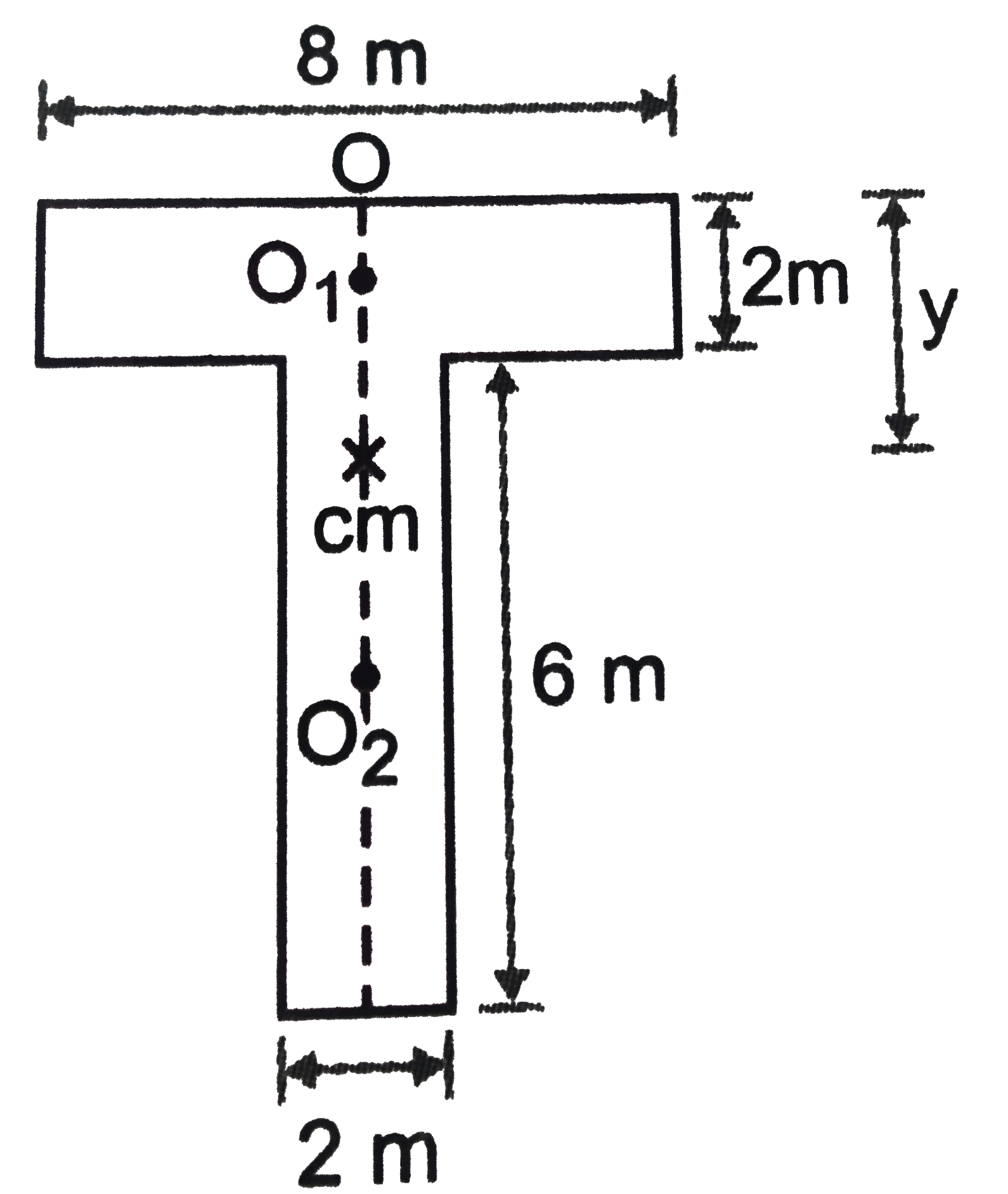 View Solution
View SolutionLocate the position of centre of mass of a uniform plate of Mass m as shown in fig 4E 34
 View Solution
View SolutionThe coordinates of the positions of particles of mass 7, 4 and 10 gm are (1, 5,-3) , (2, 5, 7) and (3, 3,-1) respectively. Find the position of the centre of mass of the system?
View SolutionThe coordinates of the positions of particles of mass 7gm,4gm and 10gm are (1,5,-3)(2,5,7)" and "(3,3,-1)cm respectively.The position of the centre of mass of the system would be:
View SolutionMass of 10 kg is distributed uniformly over a ring of radius 1m. Find the gravitational potential at a point lies on the axis of the ring at a distance of 1m from the centre.
View Solution
