Doubtnut Promotions Sticky

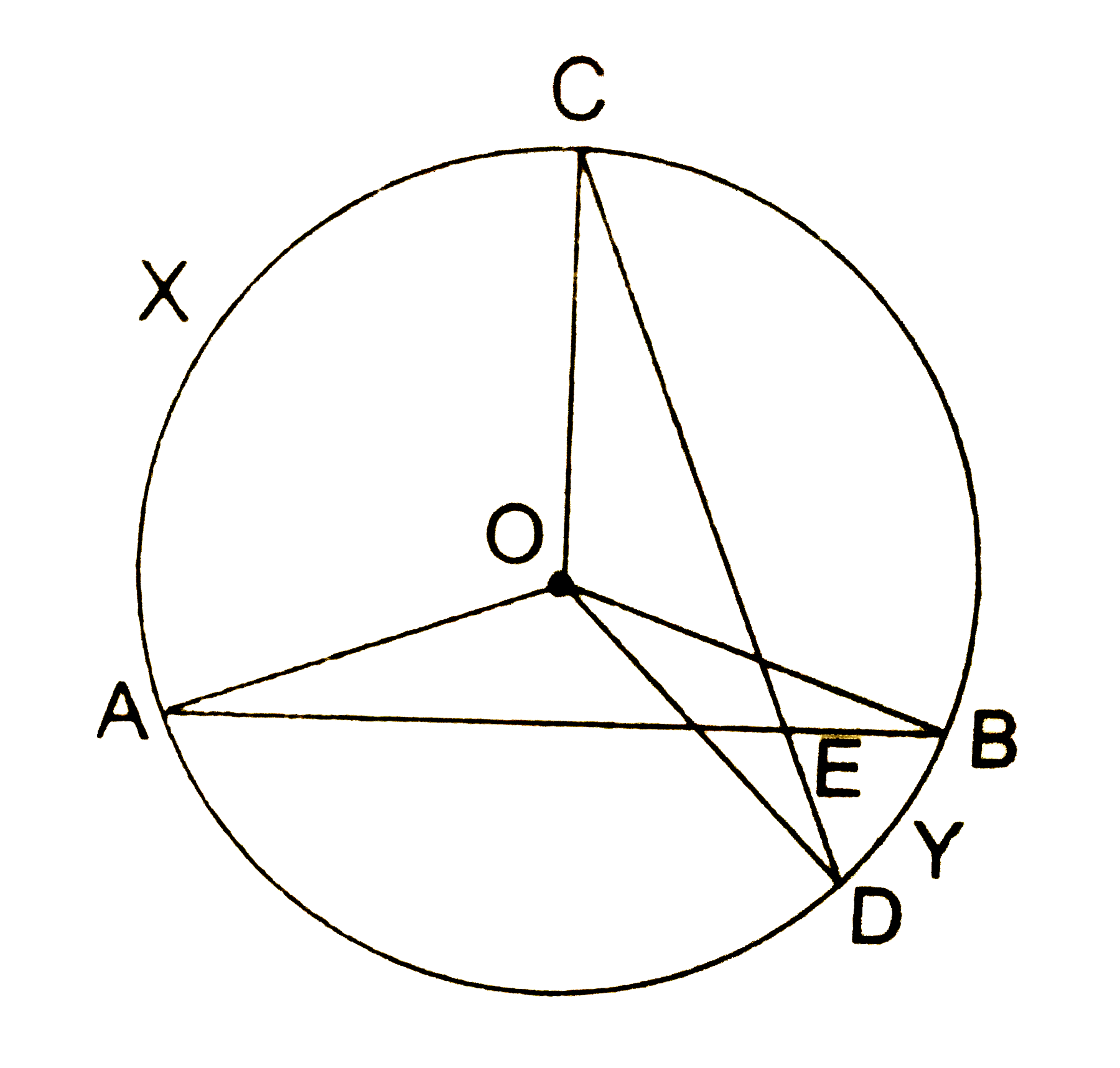
In the given figure, AB and CD are two chords of a circle, intersecting each other at a point E . Prove that
∠AEC=12( angle subtended by arc CXA, at the centre + angle subtended by arc DYB at the centre).
In the given figure, AB and CD are two chords of a circle, intersecting each other at a point E . Prove that / AEC = (1)/(2) ( angle subtended by arc CXA, at the centre + angle subtended by arc DYB at the centre).

Video Solution
Doubtnut Promotions Banner
Text Solution
Verified by Experts

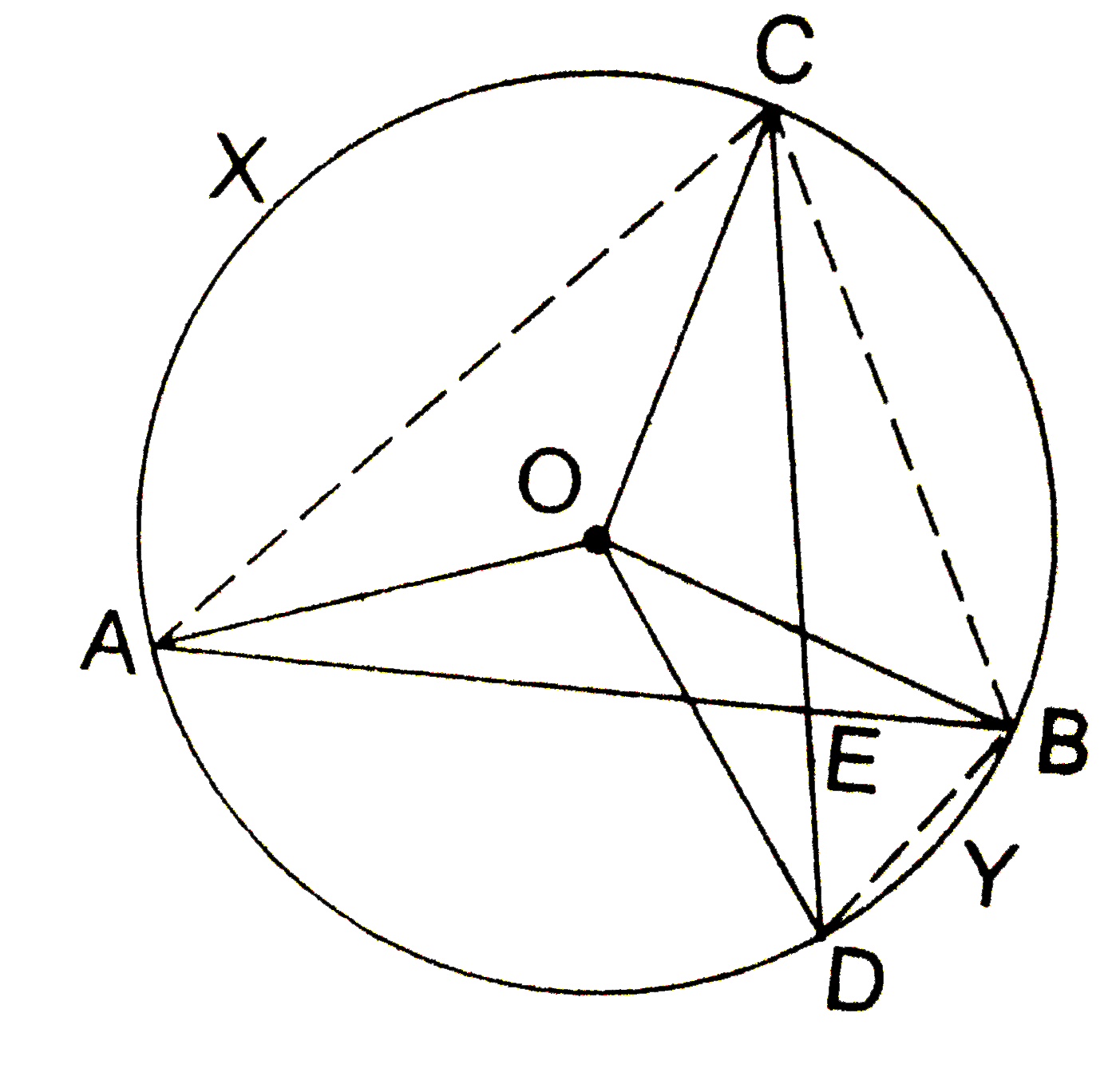
Join AC, BC and BD
Since AC is a chord , we have
∠AOC=2∠ABC ...(i)
Similarly , ∠DOB=2∠DCB. ....(ii)
∴∠AOC+∠DOB=2(∠ABC+∠DCB)=2∠AEC.
Hence, ∠AEC=12(∠AOC+∠DOB)
ocr_image

|
Updated on:21/07/2023
Class 9MATHSCIRCLES

Topper's Solved these Questions

Similar Questions

Knowledge Check

  • Question 1 - Select One

    Which of the following can be the angle subtended by a minor arc at the centre?

    A100∘
    B220∘
    C180∘
    D270∘
  • Question 2 - Select One

    A, B and C are the three points on a circle such that ∠ABC=35∘and∠BAC=85∘. What is the angle (in degrees) subtended by arc AB at the centre of the circle?

    A60
    B90
    C135
    D120
  • Question 3 - Select One

    If 76 is divided into four parts proportional to 7, 5, 3, 4, then the smallest part is:

    AA) 12
    BB) 15
    CB) 15
    DD) 19