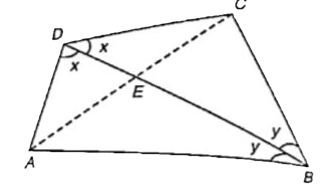
In a quadrilateral ABCD the linesegment bisecting ∠Cand∠D meet at E. Prove that ∠A+∠B=2∠CED.
Video Solution
Text Solution
Let CE and DE be the bisectors of ∠Cand∠D respectively. Then, ∠1=12∠Cand∠2=12∠D.
In ΔDEC, we have ∠1+∠2+∠CED=180∘(sum of the ∠ of a △ is 180∘)
⇒∠CED=180∘−(∠1+∠2). ...(i)
Again, the sum of the angles of a quadrilateral is 360∘.
∴∠A+∠B+∠C+∠D=360∘
⇒12(∠A+∠B)+12∠C+12∠D=180∘
⇒12(∠A+∠B)+∠1+∠2=180∘
⇒12(∠A+∠B)=180∘−(∠1+∠2). ...(ii)
From (i) and (ii), we get 12(∠A+∠B)=∠CED.
Hence, ∠A+∠B=2∠CED.
|
Updated on:21/07/2023
Topper's Solved these Questions
- BOOK - RS AGGARWALCHAPTER - QUADRILATERALSEXERCISE - Exercise 10 A10videos
QUADRILATERALS
- BOOK - RS AGGARWALCHAPTER - QUADRILATERALSEXERCISE - EXERCISE 10B27videos
QUADRILATERALS
- BOOK - RS AGGARWALCHAPTER - QUADRILATERALSEXERCISE - Matching of Columns:2videos
QUADRILATERALS
- BOOK - RS AGGARWALCHAPTER - PROBABILITYEXERCISE - Multiple Choice Questions (Mcq)16videos
PROBABILITY
- BOOK - RS AGGARWALCHAPTER - TRIANGLESEXERCISE - MULTIPLE-CHOICE QUESTIONS (MCQ)10videos
TRIANGLES
Similar Questions
Knowledge Check
- Question 1 - Select One
In the given figure, ABCD is a quadrilateral, the line segments bisecting ∠Cand∠D meet at E. Then 2∠CED is equal to
 A∠A+∠BB∠A+∠CC∠B+∠DD∠C+∠D
A∠A+∠BB∠A+∠CC∠B+∠DD∠C+∠D - Question 2 - Select One
The diagonal BD of a quadrilateral ABCD bisects ∠Band∠D, then :
 AABCD=ADBCBADAB=BCCDCABBC=ADCDDAB=AD×BC
AABCD=ADBCBADAB=BCCDCABBC=ADCDDAB=AD×BC - Question 3 - Select One
If bisectors of ∠Aand∠B of a quadrilateral ABCD intersect each other at P, of ∠Band∠C at Q, of ∠Cand∠D at R and of ∠Dand∠A at S, then PQRS is a
ArectangleBrhombusCparallelogramDquadrilateral whose opposite angles are supplementary
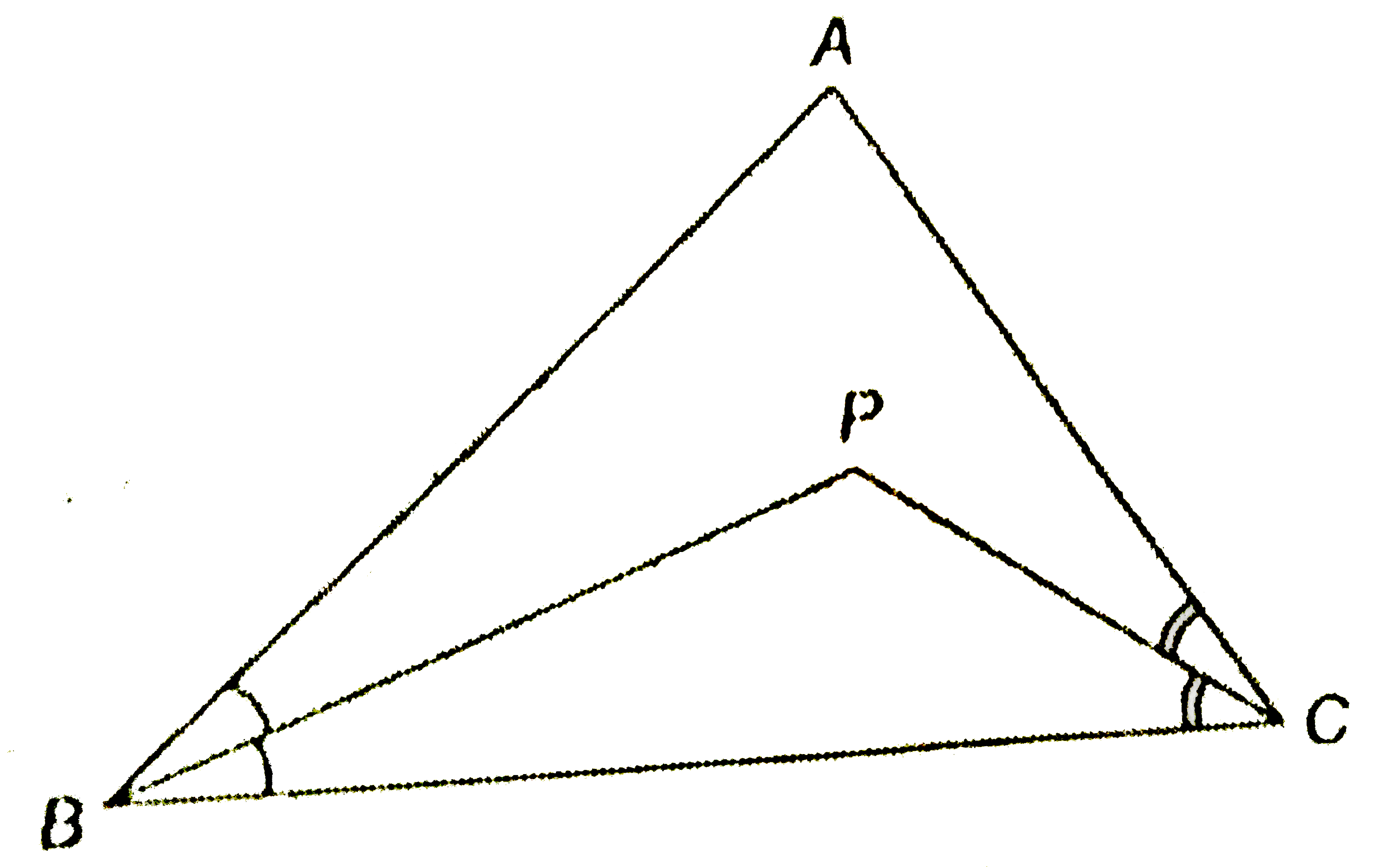
In the adjoining figure, AB>AC and the angle bisectors of ∠B and ∠C meet at point P. Prove that
PB>PC.
 View Solution
View SolutionIn △ABC,∠A=40∘ if bisector of ∠B and ∠C meets at O then prove that ∠BOC=110∘
View SolutionIn ΔABC exterior angle bisectors of ∠Band∠C meets at point P if ∠BAC=80∘ then find ∠BPC.
View SolutionIn a quadrilateral ABCD,∠A+∠C=180∘, then ∠B+∠D is equal to
View SolutionIn a quadrilateral ABCD∠A+∠C is 2 times ∠B+∠D. If ∠A=140∘∠D=60∘, then ∠B=
View Solution

