In the given figure, ABCD is a quadrilateral such that ∠D=90∘. A circle with centre O and radius r, touches the sides AB, BC, CD and DA at P, Q, R and S respectively. If BC=40cm, CD=25cm and BP=28cm, find r.
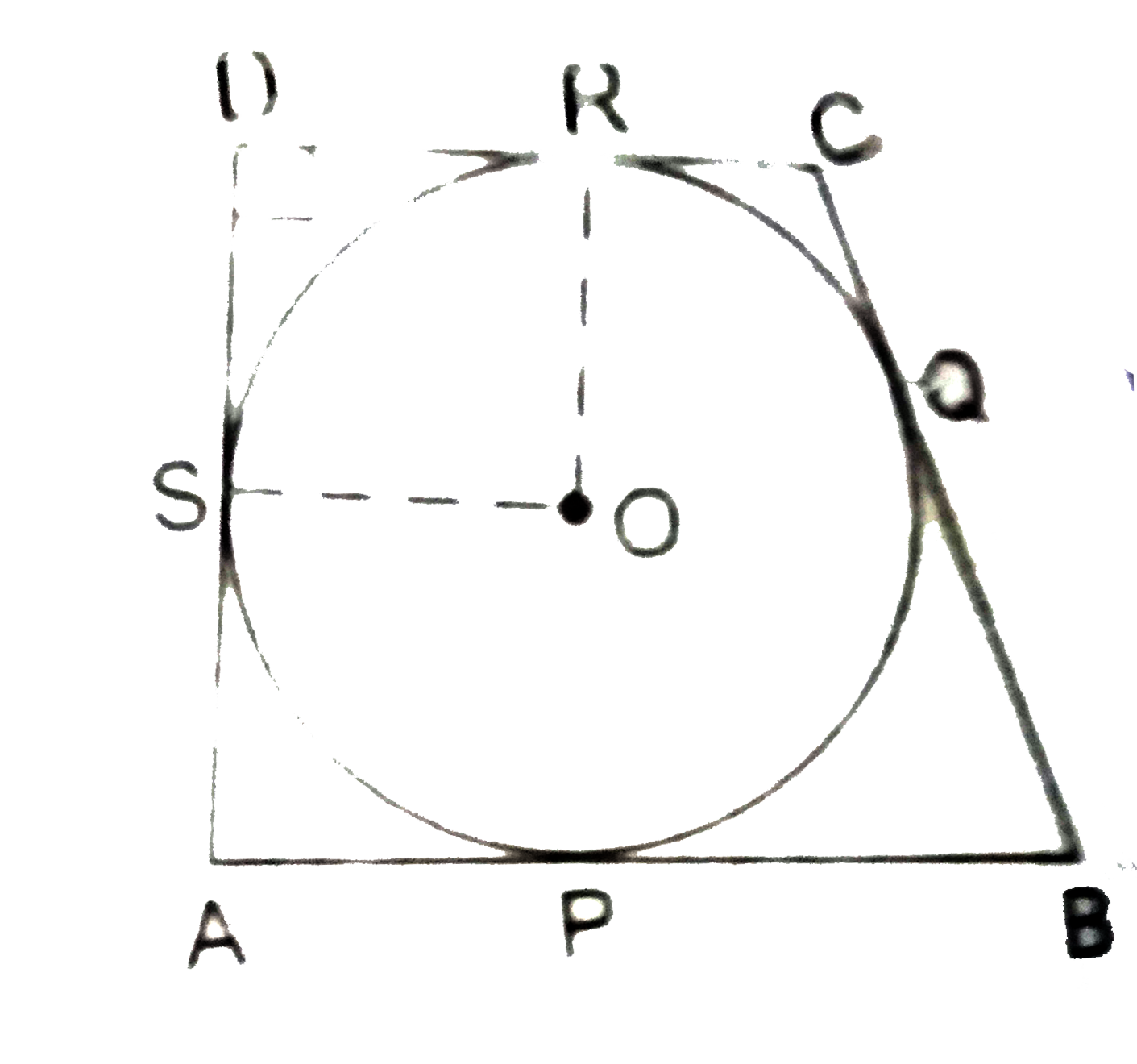
It is given that ∠D=90∘
Also, ∠ORD=∠OSD=90∘. [∵ tangent at a point is perpendicular to the radius through the point of contact]
∴∠ROS=180∘−∠D=90∘. [∵ angle between the tangents from an external point is supplementary to the angle subtended by the line segments joining the points of contact to the centre]
And, OR=OS=r.
∴ROSD is a square and so OR=DR, i.e., r=DR ......(i)
We know that the lengths of tangents drawn from an exterior point to a circle are equal.
∴BP=BQ and CQ=CR
Now, CQ=BC−BQ=BC−BP=(40−28)cm=12cm
And so, DR=CD−CR=CD−CQ=(25−12)cm=13cm
∴r=DR=13cm [using (i)]
Topper's Solved these Questions
- CIRCLESBOOK - RS AGGARWALCHAPTER - CIRCLESEXERCISE - Exercise 8A
16 Videos
- CIRCLESBOOK - RS AGGARWALCHAPTER - CIRCLESEXERCISE - Exercise 8B
15 Videos
- ARITHMETIC PROGRESSIONBOOK - RS AGGARWALCHAPTER - ARITHMETIC PROGRESSIONEXERCISE - Multiple Choice Questions (Mcq)
30 Videos
- CONSTRUCTIONSBOOK - RS AGGARWALCHAPTER - CONSTRUCTIONSEXERCISE - Test Yourself
10 Videos
Similar Questions
ABCD is a quadrilateral such that ∠D=90∘. A circle C of radius r touches the sides AB, BC, CD and DA at P, Q, R and S respectively. If BC = 38 cm, CD = 25 cm and BP = 27 cm. Find r
View SolutionIn the given figure, ABCD is a quadrilateral in which ∠D=90∘. A circle C(O,r) touches the side AB, BC, CD and DA at P,Q,R and S, respectively. If BC= 38 cm, CD = 25 cm and BP = 27 cm, then find the value of r.
View SolutionABCD is a quadrilateral such that ∠D=90∘. A circle with centre O touches the sides AB, BC and CD and DA at P, Q, R and S respectively. If BC = 40 cm, BP = 28 cm and CD = 25 cm, then what is the radius of the circle is:
ABCD एक चतुर्भुज है जैसे ∠D=90∘ । केन्द्र O वाला एक वृत्त क्रमशः AB, BC और CD और DA को P, Q,R और S से छूता है। यदि BC = 40 सेमी, BP = 28 सेमी और CD = 25 सेमी है, तो वृत्त का त्रिज्या क्या है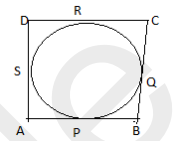
View SolutionIn the given figure, ABCD is a quadrilateral in which ∠D=90. A circle C(O,r) touches the sides AB,BC,CD,DA at P,Q,R and S respectively. If BC=38 cm, CD=25 cm, and BP=27 cm, then find the value of r.
View SolutionIN the given figure, ABCD is a quadrilateral in which ∠ADC=90∘,BC=38cm,CD=28cm and BP=25cm. Find the radius of the circle with centre O.
View SolutionABCD is a quadrilateral such that ∠D=90∘. A circle C of radius r touches the sides AB, BC, CD and DA at P, Q, R and S respectively. If BC = 38 cm, CD = 25 cm and BP = 27 cm. Find r
View SolutionIn Fig.2, a quadrilateral ABCD is drawn to circumscribe a circle, with centre O, in such a way that the sides AB, BC, CD and DA touch the circle at the points P, Q, R and S respectively. Prove that AB + CD = BC + DA.
View SolutionIn the following figure ,a circle with centre O is inscribed in a quadrilateral ABCD such that ,it touches the sides BC, AB, AD and CD at points P,Q , R and S respectively .If AB =29 cm , AD =23 cm ∠B=90∘andDS=5 cm.
Find the value of DR :
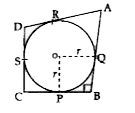
View SolutionIn Fig. 2, a circle with centre O is inscribed in a quadrilateral ABCD such that, it touches the sides BC, AB, AD and CD at points P, Q, R and S respectively. If AB=29 cm, AD-23 cm,∠B=90° and DS = 5 cm, then the radius of the circle (in cm)
View SolutionIn figure, a quadrilateral ABCD is drawn to circumscribe a circle such that its sides AB, BC, CD and AD touch the circle at P, Q, R and S respectively. If AB = x cm, BC = 7 cm, CR = 3cm and AS = 5cm, then x = ______.

View Solution
