Two circles with radii r1andr2,r1>r2≥2, touch other externally. If 'α' be the angle between direct common tangents ,then Solution in Telugu
α=sin−1(r1+r2r1−r2)
α=2sin−1(r1−r2r1+r2)
α=sin−1(r1−r2r1+r2)
α=sin−1(r1r1)
The correct Answer is:B
Step by step video, text & image solution for Two circles with radii r_1 and r_2, r_1 gt r_2 ge 2, touch other externally. If 'alpha' be the angle between direct common tangents ,then by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Topper's Solved these Questions
- BOOK - AAKASH SERIESCHAPTER - REVISION EXERCISE EXERCISE - SYSTEM OF CIRCLES22videos
REVISION EXERCISE
- BOOK - AAKASH SERIESCHAPTER - REVISION EXERCISE EXERCISE - PARABOLA50videos
REVISION EXERCISE
- BOOK - AAKASH SERIESCHAPTER - RANDOM VARIABLESEXERCISE - Exercise 4.49videos
RANDOM VARIABLES
- BOOK - AAKASH SERIESCHAPTER - SEQUENCE AND SERIESEXERCISE - Practice Exercise71videos
SEQUENCE AND SERIES
Similar Questions
The condition that the circles which passes through the points (0,a),(0,−a) and touch the line y=mx+c will cut orthogonally is
04:41View SolutionThe curve describe parametrically by x=t2+t+1,y=t2−t+1 represents
17:15View SolutionA random variable X takes four values with probabilities 1+3x4,(1−x),1+2x4 and 1−4x4. Find the set of values of x for the variable X to have probability distribution.
05:09View SolutionIf sum of the series a−(a+d)+(a+2d)−(a+3d)+….up to 21 terms is
04:02View SolutionC_1 and C_2 are fixed circles of radii r_1 and r_2 touches each other externally. Circle 'C' touches both Circles C_1 and C_2 extemelly. If r_1/r_2=3/2 then the eccentricity of the locus of centre of circles C is
04:04View SolutionTwo circles centres AandB radii r1andr2 respectively. (i) touch each other internally iff |r1−r2|=AB. (ii) Intersect each other at two points iff |r1−r2|<AB<r1r2. (iii) touch each other externally iff r1+r2=AB. (iv) are separated if AB>r1+r2. Number of common tangents to the two circles in case (i), (ii), (iii) and (iv) are 1, 2, 3 and 4 respectively. Number of common tangents to the circles x2+y2−6x=0andx2+y2+2x=0 is (A) 1 (B) 2 (C) 3 (D) 4
04:22View SolutionIf the arcs of the same length in two circles of radii r1andr2 respectively subtend angle π3and120∘ at the centre ,then the value of r1+r2r1−r2 will be
04:15View SolutionWhen two soap bubbles of radii r_1 and r_2 (r_2 lt r_1) adjoin, the radius of curvature of the common surface is
04:49View Solutionदो संकेन्द्रीय पतले गोलों की त्रिज्याएँ R1 तथा R2(R1>R2) हैं तथा इन पर क्रमशःQ1 व Q2 आवेश हैं। तब त्रिज्या r जो R1 व R2 के बीच है, पर विभव 14πεo का कितने गुना होगा?
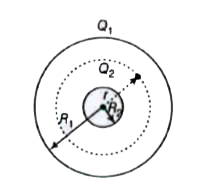View Solutionকোনাে তড়িৎবর্তনীতে e তড়িৎচালক বলবিশিষ্ট দুটি তড়িৎকোশকে শ্রেণি সমবায়ে বহিস্থ R রােধের সাথে যুক্ত করা হল কৌশদ্বয়ের অভ্যন্তরীণ রােধ যথাক্রমে r1এবং r2 | প্রবাহ চলাকালীন যদি প্রথম কোশের বিভবপার্থক্য শূন্য হয় তবে R এবং r1 ও r2 এর মধ্যে সম্পর্ক হবে R=r1+r2 R=r1−r2 R=½(r1+r2) R=½(r1−r2)
02:31View SolutionTwo fixed circles with radii r1andr2,(r1>r2) , respectively, touch each other externally. Then identify the locus of the point of intersection of their direction common tangents.
04:02View SolutionThe magnitudes of the gravitational fields at distances r1 and r2 from the centre of a uniform sphere of radius R and mass M are F1 and F2 respectively then
(a)F1F2=r1r2 if r1<R and r2<R
(b)F1F2=(r2r1)2 if r1>R and r2>R
(c)F1F2=r1r2 if r1>R and r2>R
(d)F1F2=(r1r2)2 if r1<R and r2<R03:55View SolutionTwo concentric thin metallic spheres of radii R_1 and R_2 (R_1 gt R_2) bear charges Q_1 and Q_2 respectively. Then the potential at radius r between R_1 and R_2 will be (k =1//4 pi in_0)
08:02View SolutionWhen two soap bubbles of radii r_1 and r_2 ( r_2>r_1 )coalese, the radius of curvature of common surface is
06:31View SolutionWhen two soap bubbles of radii r1andr2(r2>r1) are in contact, the radius of curvature of common surface is
03:16View Solution