The resistance in the two arms of the meter bridge are 5Ω and Rω respectively. When the resistance R is shunted with an equal resistance, the new balance point is at 1.6l1. The resistance R is

10Ω
15Ω
20Ω
25Ω
The correct Answer is:B
In the first case,
At balance point
5R=l1100−l1 …(i)
In the second caes,
At balance point
5(R/2)=1.6l1100−1.6l1 ...(ii)
Divide eqn. (i) by eqn. (ii), we get
12=100−1.6l11.6(100−l1)
160−1.6l1=200−3.2l1
1.6l1=40orl1=401.6=25cm
Substituting this value in eqn. (i), we get
5R=2575
R=37525Ω=15Ω

Topper's Solved these Questions
- CURRENT ELECTRICITY Book:MTG GUIDEChapter:CURRENT ELECTRICITY Exercise:CHECK YOUR NEET VITALS
Explore 16 Videos
- ATOMS AND NUCLEIBook:MTG GUIDEChapter:ATOMS AND NUCLEIExercise:AIPMT/NEET(MCQs)
Explore 40 Videos
- DUAL NATURE OF MATTER AND RADIATION Book:MTG GUIDEChapter:DUAL NATURE OF MATTER AND RADIATION Exercise:AIPMT/ NEET MCQs
Explore 30 Videos
Similar Questions
The resistance in the two arms of the meter bridge are 5Ω and RΩ, respectively. When the resistance R is shunted with an equal resistance, the new balance point is 1.6l1.
The resistance R is
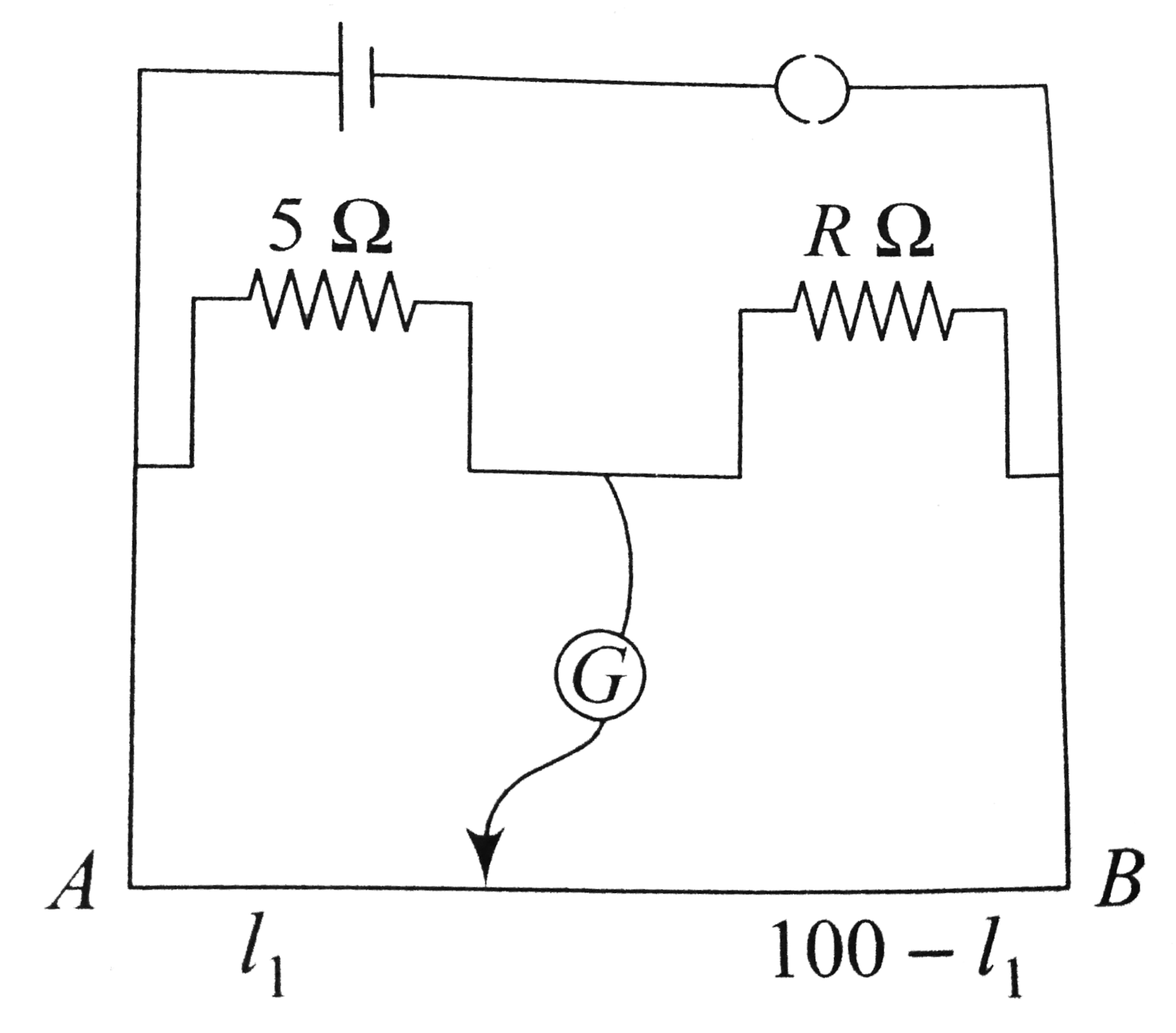
View SolutionThe resistance in the two arms of the meter bridge are 5Ω and RΩ, respectively. When the resistance R is shunted with an equal resistance, the new balance point is 1.6l1.
The resistance R is

View Solution
Knowledge Check
The resistance in the two arms of the meter bridge are 5Ω and RΩ, respectively. When the resistance R is shunted with an equal resistance, the new balance point is 1.6l1.
The resistance R is
 A10ΩB15ΩC20ΩD25Ω
A10ΩB15ΩC20ΩD25ΩThe resistance in the two arms of the meter bridge are 5Ω and RΩ, respectively. When the resistance R is shunted with an equal resistance, the new balance point is 1.6l1.
The resistance R is
 A10ΩB15ΩC20ΩD25Ω
A10ΩB15ΩC20ΩD25ΩResistance in the two gaps of a meter bridge are 10ohm and 30ohm respectively. If the resistances are interchanged the balance point shifts by
A33.3cmB66.67cmC25cmD50cm
What is end error in a metre bridge ? How is it overcome ? The resistances in the two arms of the metre bridge are R=5Ω and S respectively. When the resistance S is shunted with an equal resistance, the new balance length found to be 1.5l1, where l1 is the initial balancing length. Calculate the value of S.
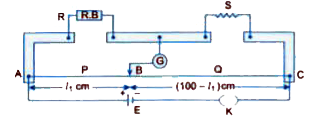
View SolutionWhat is end error in a metre bridge ? How is it overcome ? The resistances in the two arms of the metre bridge are R=5Ω and S respectively.
When the resistance S is shnted with an equal resistance, the new balance length forund to be 1.5 l1, Where l1 is the initial balancing length. Calculate the value of S.
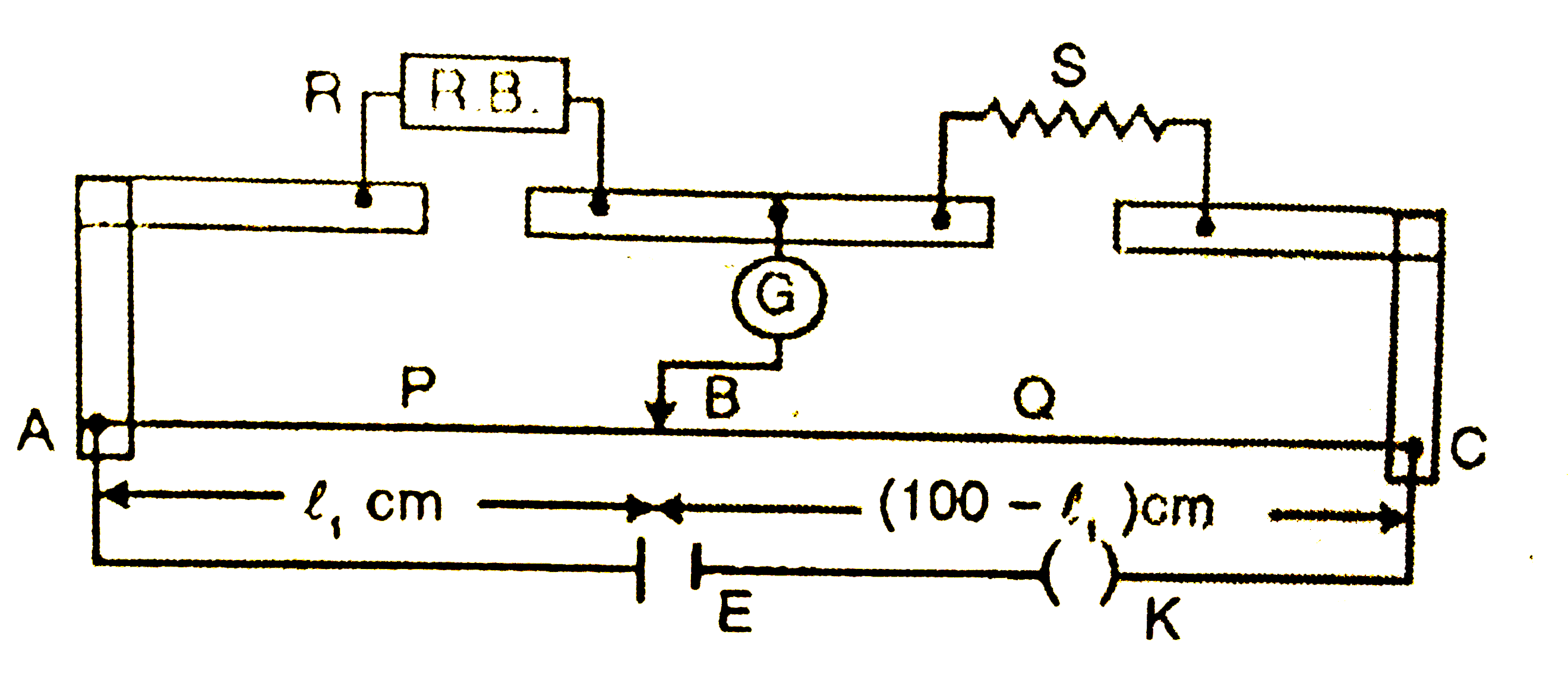
View SolutionThe resistances in the two arms of the metre bridge are R=5Ω and S respectively.
When the resistance S is shnted with an equal resistance, the new balance length forund to be 1.5 i1, Where I1 is the initial balancing length. Calculate the value of S.

View SolutionResistance in the two gaps of a meter bridge are 10ohm and 30ohm respectively. If the resistances are interchanged he balance point shifts by
View SolutionThe resistance in two arms of the meter bridge is 10Ω and x respectively. The balance length is l1from left. Now the resistance of 10Ω is shunted with another resistor of resistance equal to unknown resistance x then new balance point is 0.75l1 The value of resistance x is
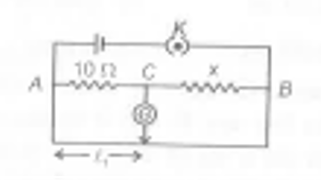
View Solution
