The equivalent resistance of network of three resistors can not be
The correct Answer is:B
To determine the equivalent resistance of a network of three 2Ω resistors, we need to consider the different ways these resistors can be connected: in series and in parallel.
Step 1: Calculate Equivalent Resistance in Series
When resistors are connected in series, the equivalent resistance (R_eq) is simply the sum of their resistances.
Req=R1+R2+R3
Given that each resistor has a resistance of 2Ω:
Req=2Ω+2Ω+2Ω=6Ω
Step 2: Calculate Equivalent Resistance in Parallel
When resistors are connected in parallel, the formula for equivalent resistance is given by:
1Req=1R1+1R2+1R3
Substituting the values of the resistors:
1Req=12Ω+12Ω+12Ω
Calculating this gives:
1Req=32Ω⟹Req=23Ω≈0.67Ω
Step 3: Mixed Combination
Now, let's consider a mixed combination where two resistors are in parallel and one is in series with them.
1. Calculate the equivalent resistance of two resistors in parallel:
1Rparallel=12Ω+12Ω=22Ω=1Ω
2. Now add the third resistor in series:
Rtotal=Rparallel+R3=1Ω+2Ω=3Ω
Conclusion
From the calculations, we have found the following possible equivalent resistances:
- In series: 6Ω
- In parallel: 0.67Ω (or 2/3Ω)
- Mixed combination (2 in parallel, 1 in series): 3Ω
Answer
The equivalent resistance of the network of three 2Ω resistors cannot be equal to any value other than those calculated. Therefore, the equivalent resistance cannot be:
- 1Ω
- 2Ω
- 4Ω
- 5Ω
When resistors are connected in series, the equivalent resistance (R_eq) is simply the sum of their resistances.
When resistors are connected in parallel, the formula for equivalent resistance is given by:
Now, let's consider a mixed combination where two resistors are in parallel and one is in series with them.
From the calculations, we have found the following possible equivalent resistances:
- In series: 6Ω
- In parallel: 0.67Ω (or 2/3Ω)
- Mixed combination (2 in parallel, 1 in series): 3Ω
The equivalent resistance of the network of three 2Ω resistors cannot be equal to any value other than those calculated. Therefore, the equivalent resistance cannot be:
- 1Ω
- 2Ω
- 4Ω
- 5Ω
Topper's Solved these Questions
- BOOK - MCGROW HILL PUBLICATIONCHAPTER - MISCELLANEOUS QUESTIONSEXERCISE - PART-B99videos
MISCELLANEOUS QUESTIONS
- BOOK - MCGROW HILL PUBLICATIONCHAPTER - MEASUREMENTEXERCISE - ELEMENTARY QUESTIONS (HIGHER ORDER THINKING QUESTIONS)13videos
MEASUREMENT
- BOOK - MCGROW HILL PUBLICATIONCHAPTER - MOTIONEXERCISE - HIGHER ORDER THINKING QUESTIONS48videos
MOTION
Similar Questions
Establish a relationship to determine the equivalent resistance R of a combination of three resistors having resistances R1,R2andR3 connected in series.
(b) Calculate the equivalent resistance R of a combination of three resistors of 2Ω,3Ωand6Ω joined in parallel.View SolutionFind an expression for the equivalent resistance of three resistors joined in series.
View Solution
Knowledge Check
- Question 1 - Select One
The equivalent resistance of network of three 4Ω resistors can not be
A12ΩB1.33ΩC6ΩD4Ω - Question 2 - Select One
The equivalent resistance of network of three 4Ω resistors can not be
A12ΩB1.33ΩC6ΩD4Ω - Question 3 - Select One
The equivalent resistance of three equal resistances connected in series is 27Ω. There equivalent resistance when connected in parallel is
A54ΩB270ΩC3ΩD9Ω
For the series combination of three resistors establish the relation :
R=R1+R2+R3
where the symbols have their usual meanings.
Calculate the equivalent resistance of the combination of three resistors of 6Ω,9Ω and 18Ω joined in parallel.View SolutionTwelve equal resistors each R Ω are connected to from the edges of a cube . Find the equivalent resistance of the network.
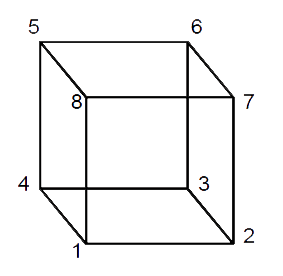
When current enters at 1 and leaves at 2View SolutionTwelve equal resistors each R Ω are connected to from the edges of a cube . Find the equivalent resistance of the network.
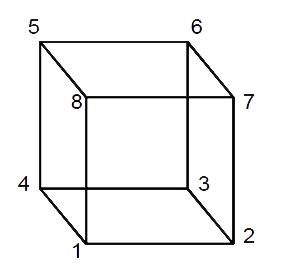
When current enters at 1 and leaves at 3View SolutionFind an expression for equivalent resistance of three resistors joined in parallel.
View SolutionFind the equivalent resistance of the network given in the figure,
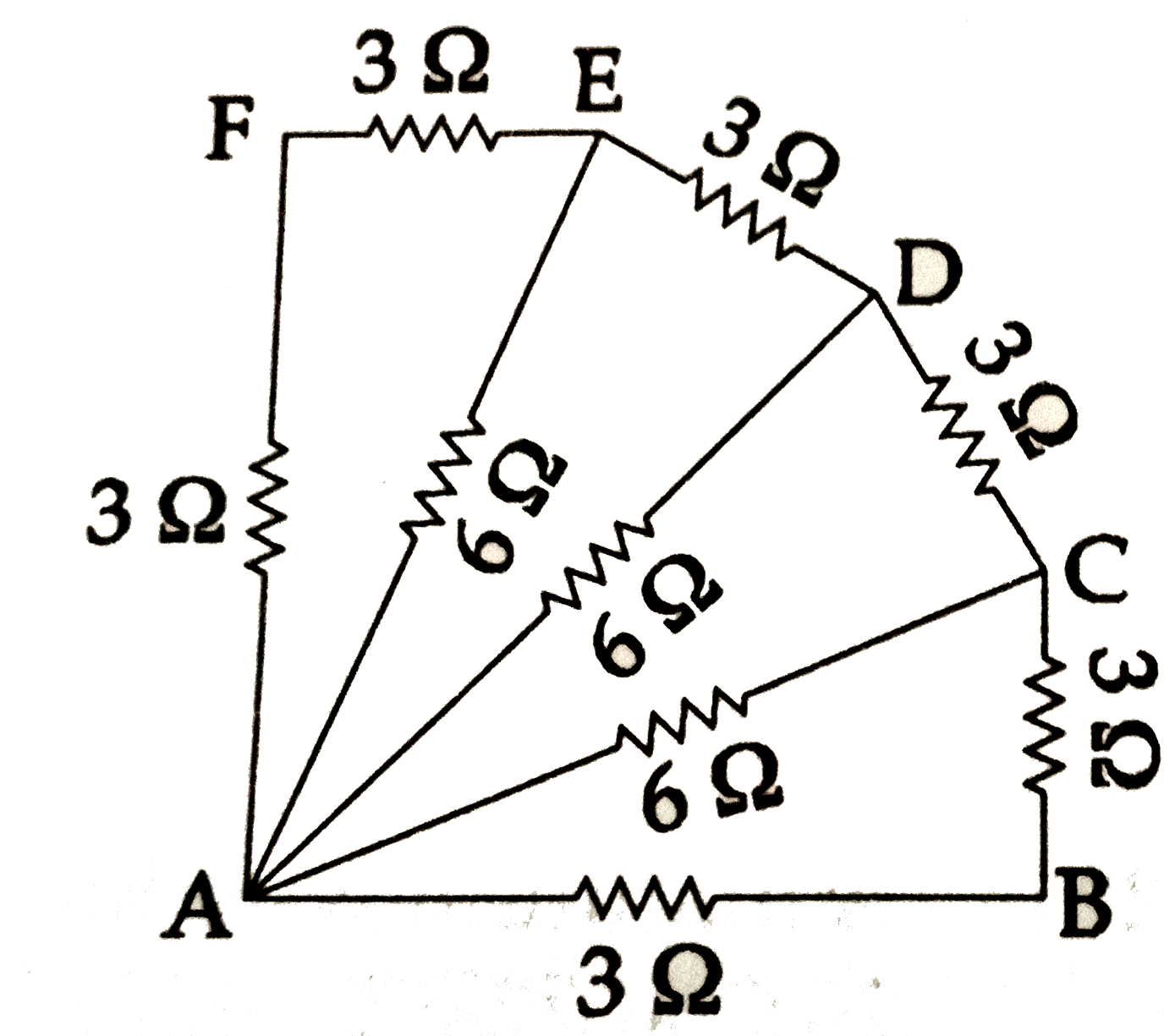 View Solution
View Solution

