A thin convex lens of focal length 25cm is cut into two pieces 0.5cm above the principal axis. The top part is placed at (0,0) and an object placed at (−50cm,0). Find the coordinates of the image.
To solve the problem step by step, we will analyze the situation involving the thin convex lens that has been cut and the object placed in front of it.
Step 1: Understand the setup
We have a thin convex lens with a focal length (f) of 25 cm. It is cut into two pieces, and the top part is placed at the coordinates (0, 0) with a height of 0.5 cm above the principal axis. The object is placed at (-50 cm, 0).
Step 2: Identify the coordinates of the lens
Since the top part of the lens is at (0, 0) and is raised by 0.5 cm, we can denote the optical center of the lens as (0, 0.5).
Step 3: Determine the object distance (u)
The object is located at (-50 cm, 0). The object distance (u) is measured from the optical center of the lens to the object. Since the object is to the left of the lens, we take it as negative:
- u = -50 cm
Step 4: Use the lens formula
The lens formula is given by:
1v−1u=1f
Where:
- v = image distance from the lens
- u = object distance
- f = focal length
Substituting the values:
1v−1−50=125
This simplifies to:
1v+150=125
Step 5: Solve for v
To solve for v, we first find a common denominator:
1v=125−150
Calculating the right side:
125=250
Thus:
1v=250−150=150
So, we have:
v=50 cm
Step 6: Determine the y-coordinate of the image
To find the y-coordinate of the image, we can use the magnification formula:
M=h′h=−vu
Where:
- h' = height of the image
- h = height of the object
- M = magnification
Since the object is at (−50, 0), we can assume its height (h) is 0. The image distance (v) is 50 cm and the object distance (u) is -50 cm:
M=−50−50=1
This means the height of the image is the same as the height of the object, but since the image is formed below the principal axis, it will be negative:
h′=−1 cm
Step 7: Final coordinates of the image
The coordinates of the image can now be determined:
- The x-coordinate is 50 cm (as calculated).
- The y-coordinate is -1 cm (due to the negative height).
Thus, the coordinates of the image are:
(50,−1)
We have a thin convex lens with a focal length (f) of 25 cm. It is cut into two pieces, and the top part is placed at the coordinates (0, 0) with a height of 0.5 cm above the principal axis. The object is placed at (-50 cm, 0).
Since the top part of the lens is at (0, 0) and is raised by 0.5 cm, we can denote the optical center of the lens as (0, 0.5).
The object is located at (-50 cm, 0). The object distance (u) is measured from the optical center of the lens to the object. Since the object is to the left of the lens, we take it as negative:
- u = -50 cm
The lens formula is given by:
1v−1u=1f
Where:
- v = image distance from the lens
- u = object distance
- f = focal length
1v−1−50=125
This simplifies to:
1v+150=125
To solve for v, we first find a common denominator:
1v=125−150
Calculating the right side:
125=250
Thus:
1v=250−150=150
So, we have:
v=50 cm
To find the y-coordinate of the image, we can use the magnification formula:
M=h′h=−vu
Where:
- h' = height of the image
- h = height of the object
- M = magnification
M=−50−50=1
This means the height of the image is the same as the height of the object, but since the image is formed below the principal axis, it will be negative:
h′=−1 cm
The coordinates of the image can now be determined:
- The x-coordinate is 50 cm (as calculated).
- The y-coordinate is -1 cm (due to the negative height).
(50,−1)
Topper's Solved these Questions
- RAY OPTICS AND OPTICAL INSTRUMENTS BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - RAY OPTICS AND OPTICAL INSTRUMENTS EXERCISE - MULTIPLE CHOICE QUESTIONS (MORE THAN ONE OPTIONS )
6 Videos
- NUCLEIBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - NUCLEIEXERCISE - Long answer type question
10 Videos
- SEMICONDUCTOR ELECTRONICS: MATERIAL,DEVICES AND SIMPLE CIRCUITBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - SEMICONDUCTOR ELECTRONICS: MATERIAL,DEVICES AND SIMPLE CIRCUITEXERCISE - Multiplel choice questions
26 Videos
Similar Questions
A converging lens of focal length 20 cm and a converging mirror of focal length 10 cm are placed 60 cm apart with common principal axis. A point source is placed in between the lens and the mirror at a distance of 50 cm from the lens. Find the locations of the images formed.
View SolutionA convex lens has a focal length of 20 cm. It is used to form an image of an object placed 15 cm from lens. The image is
View Solution
Knowledge Check
A convex lens of focal length 15 cm is placed on a plane mirror. An object is placed at 30 cm from the lens. The image is
Areal, at 30 cm in front of the mirrorBreal, at 30 cm behind the mirrorCreal, at 10 cm in front of the mirrorDvirtual, at 10 cm behind the mirror
An optical system consists of a thin convex lens of focal length 30cm and a plane mirror placed 15cm behind the lens. An object is placed 15cm in front of the lens. The distance of the final image from the object is
View SolutionA converging lens of focal length 15 cm and a converging mirror of focal length 10 cm are placed 50 cm apart with common principal axis. A point source is placed in between the lens and the mirror at a distance of 40 cm from the lens. Find the locations of the two images formed.
View SolutionA convex lens of focal length 20cm is placed coaxilly with a convex mirror of radius of curvature 20 cm. The two are kept 15 cm apart. A point object is placed 40 cm in front of the convex lens. Find the position of the image formed by this combination. Draw the ray diagram showing the image formation.
View SolutionA thin biconvex lens of focal length 6.25 cm is made of material of refractive index 1.5. It is cut into two identical pieces perpendicular to its principal axis. One of the pieces is placed in water of refractive index 43. The focal power of the piece immersed in water in diopter is
View SolutionAn object is placed at a distance of 12 cm from a convex lens of focal length 8 cm. Find :
nature of the image
View SolutionAn object is placed at a distance of 10cm to the left on the axis of convex lens A of focal length 20cm. A second convex lens of focal length 10cm is placed coaxially to the right of the lens A at a distance of 5cm from A. Find the position of the final image and its magnification. Trace the path of the rays.
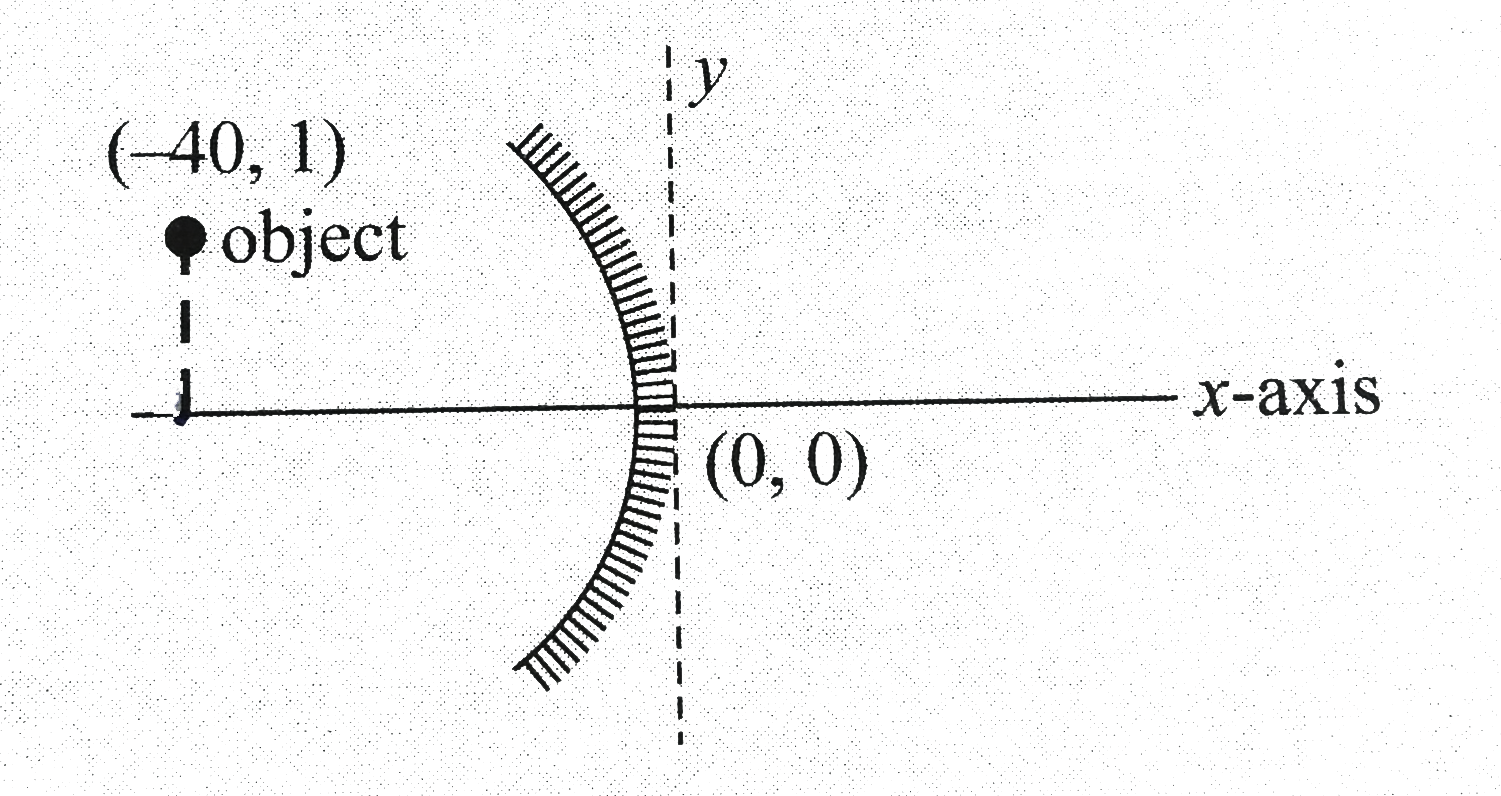
View SolutionFigure shows a shperical concave mirror of focal length f=5cm with its pole at (0,0) and principal axis along x-axis. There is a point object at (-40cm, 1cm), find the postion of image.
View Solution
