Imagine removing one electron from He4 and He3. Their energy levels, as worked out on the basis of Bohr model will be very close. Explain why ?
To understand why the energy levels of He4 and He3 are very close when one electron is removed from each, we can analyze the situation step by step using the Bohr model of the atom.
Step 1: Understanding the Atoms
- Helium-4 (He4) has 2 protons and 2 neutrons in its nucleus, giving it a mass number of 4.
- Helium-3 (He3) has 2 protons and 1 neutron, giving it a mass number of 3.
- Both isotopes have 2 electrons in their neutral state.
Step 2: Removing an Electron
- When we remove one electron from He4, we are left with a He4+ ion, which has 2 protons and 1 electron.
- When we remove one electron from He3, we are left with a He3+ ion, which also has 2 protons and 1 electron.
Step 3: Comparing the Nuclei
- The nucleus of both isotopes (He4 and He3) is significantly heavier than the mass of the electron being removed. The mass of the nucleus is on the order of atomic mass units (u), while the mass of an electron is about 1/1836 of a proton's mass.
- This means that the nucleus's influence on the electron's energy levels is much greater than the effect of the removed electron.
Step 4: Applying the Bohr Model
- According to the Bohr model, the energy levels of an electron in a hydrogen-like atom (which both He4+ and He3+ are after removing one electron) depend primarily on the nuclear charge (number of protons) and the distance of the electron from the nucleus.
- In both cases, the nuclear charge is +2 (due to 2 protons), and the remaining electron experiences a similar effective nuclear charge.
Step 5: Conclusion
- Since the nuclear charge and the effective potential experienced by the remaining electron are very similar in both cases, the energy levels calculated using the Bohr model will also be very close for He4+ and He3+.
- The difference in mass number (4 vs. 3) does not significantly affect the energy levels because the mass of the nucleus is much larger than that of the electron.
Final Answer
The energy levels of He4+ and He3+ are very close because both ions have the same nuclear charge (2 protons) and the mass of the nucleus is significantly larger than the mass of the electron removed. Thus, the energy levels calculated using the Bohr model will be similar.
---
- Helium-4 (He4) has 2 protons and 2 neutrons in its nucleus, giving it a mass number of 4.
- Helium-3 (He3) has 2 protons and 1 neutron, giving it a mass number of 3.
- Both isotopes have 2 electrons in their neutral state.
- When we remove one electron from He4, we are left with a He4+ ion, which has 2 protons and 1 electron.
- When we remove one electron from He3, we are left with a He3+ ion, which also has 2 protons and 1 electron.
- The nucleus of both isotopes (He4 and He3) is significantly heavier than the mass of the electron being removed. The mass of the nucleus is on the order of atomic mass units (u), while the mass of an electron is about 1/1836 of a proton's mass.
- This means that the nucleus's influence on the electron's energy levels is much greater than the effect of the removed electron.
- According to the Bohr model, the energy levels of an electron in a hydrogen-like atom (which both He4+ and He3+ are after removing one electron) depend primarily on the nuclear charge (number of protons) and the distance of the electron from the nucleus.
- In both cases, the nuclear charge is +2 (due to 2 protons), and the remaining electron experiences a similar effective nuclear charge.
- Since the nuclear charge and the effective potential experienced by the remaining electron are very similar in both cases, the energy levels calculated using the Bohr model will also be very close for He4+ and He3+.
- The difference in mass number (4 vs. 3) does not significantly affect the energy levels because the mass of the nucleus is much larger than that of the electron.
The energy levels of He4+ and He3+ are very close because both ions have the same nuclear charge (2 protons) and the mass of the nucleus is significantly larger than the mass of the electron removed. Thus, the energy levels calculated using the Bohr model will be similar.
Topper's Solved these Questions
- ATOMSBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - ATOMSEXERCISE - Short Answer Type
5 Videos
- ATOMSBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - ATOMSEXERCISE - Long Answer Type
6 Videos
- ATOMSBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - ATOMSEXERCISE - Long Answer Type
6 Videos
- ALTERNATING CURRENTBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - ALTERNATING CURRENTEXERCISE - Long Answe types Questions
5 Videos
- COMMUNICATION SYSTEMBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - COMMUNICATION SYSTEMEXERCISE - LONG ANSWER TYPE QUESTIONS
5 Videos
Similar Questions
An electron in an H-atom kept at rest , jumps from the mth shell to the nth shell (m>n). Suppose instead of emitting electromagnetic wave, the energy reased is converted into the kinetic energy of the atom. Assume the Bohr model and conservation of angular momentum are valid. If I is the moment of inertia the angular velocity of the atom about the nucleus is 4(m−n)h/kI. Calculate k
View SolutionSimplified model of electron energy levels for a cartain atom is shown in figure. The atom is bombarded will fast moving electrons. The impact of one of these electrons can cause the removal of electron from K-level , thus, creating a vacancy in the K-level. This vacancy in K-level is filled by an electron from l-level and the energy reased in this transition can either appear as electromagnetic waves or may all be used knock out an electron from M-level of the atom.
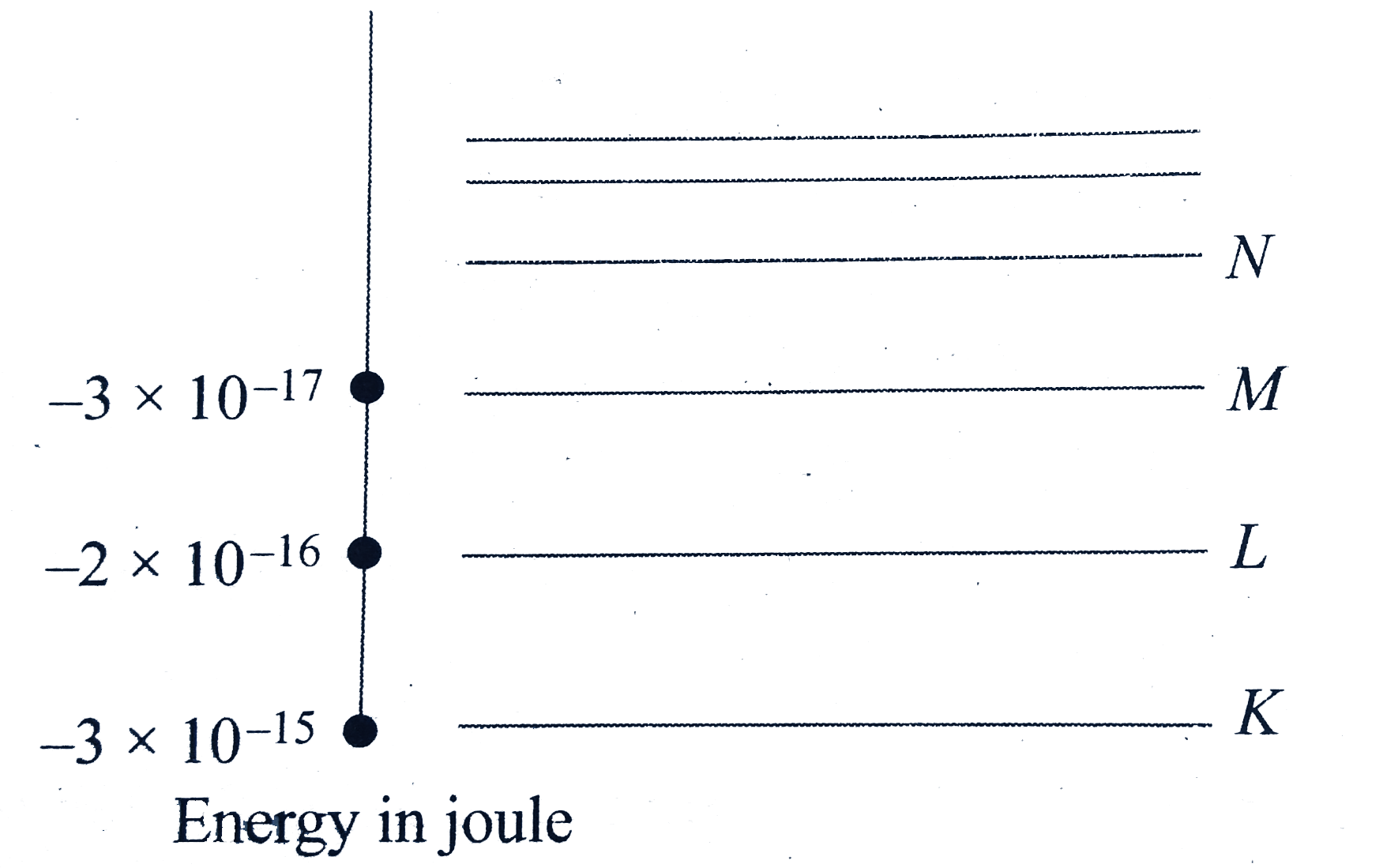
Based on above information, answer the following question:
The KE of the emitted electron from M-level is
View SolutionIf the energy needed to remove the 1st, 2nd and 3rd valence electron from an atom is V, 2V and 3V then the energy required to remove all three in one step will be xV. What is numerical value of x ?
View SolutionHydrogen atom:
The electronic ground state of hydrogen atom contains one electron in the first orbit. If sufficient energy is provided, this electron can be promoted to higher energy levels. The electronic energy of a hydrogen-like species (any atom//ions with nuclear charge Z and one electron) can be given as
En=−RHZ2n2 where RH=Rydberg constant,n=principal quantum number
The energy required to promote the ground state electron of H-atom to the first excited state is:
When an electron returns from a higher energy level to a lower energy level, energy is given out in the form of UV//Visible radiation.
View SolutionHydrogen atom:
The electronic ground state of hydrogen atom contains one electron in the first orbit. If sufficient energy is provided, this electron can be promoted to higher energy levels. The electronic energy of a hydrogen-like species (any atom//ions with nuclear charge Z and one electron) can be given as
En=−RHZ2n2 where RH=Rydberg constant,n=principal quantum number
Calculate the wavelength of light (nm) for the electronic transition of H-atom from the first excited state to ground state.
In the model of hydrogen like atom put forward by Niels Bohr (1913) the electron orbits around the central nucleus. the bohr radius of nth orbit of a hydrogen-like species is given by
r=kn2Z " " where, k is constant
View SolutionHydrogen atom:
The electronic ground state of hydrogen atom contains one electron in the first orbit. If sufficient energy is provided, this electron can be promoted to higher energy levels. The electronic energy of a hydrogen-like species (any atom//ions with nuclear charge Z and one electron) can be given as
En=−RHZ2n2 where RH=Rydberg constant,n=principal quantum number
What is the principal quantum number, n' of the orbit of Be3 that has the same Bohr radius as that of ground state hydrogen atom ?
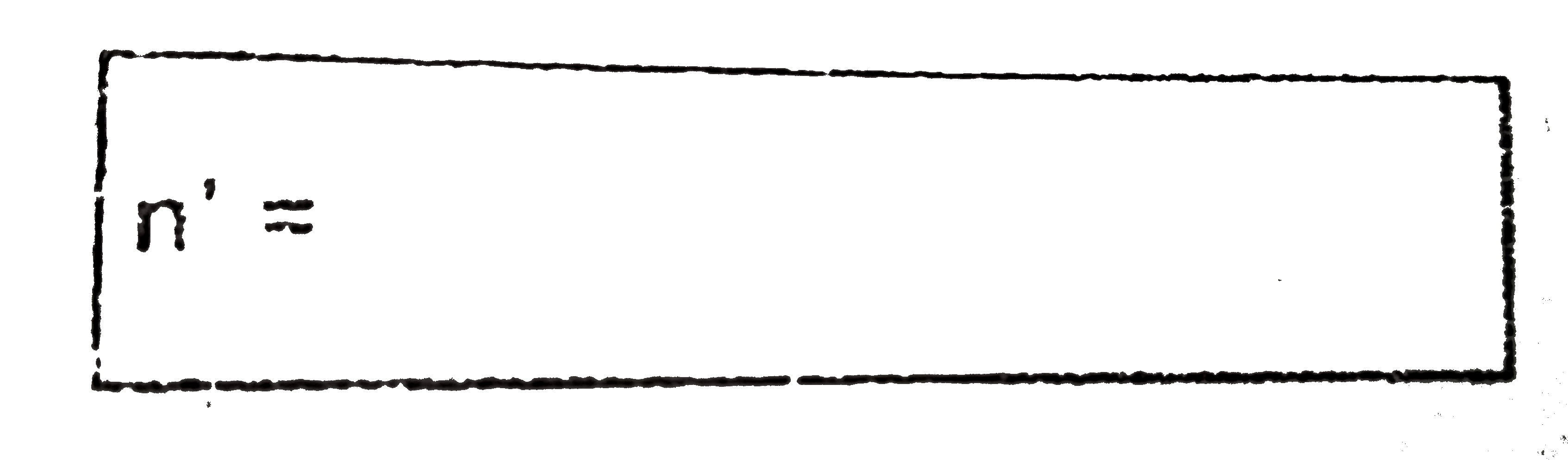
View SolutionHydrogen atom:
The electronic ground state of hydrogen atom contains one electron in the first orbit. If sufficient energy is provided, this electron can be promoted to higher energy levels. The electronic energy of a hydrogen-like species (any atom//ions with nuclear charge Z and one electron) can be given as
En=−RHZ2n2 where RH=Rydberg constant,n=principal quantum number
The ratio of energy of an electron in the ground state Be3− ion to that of ground state H atom is:
The kinetic and potential energies of an electron in the H atoms are given as
K.E.=e24πε02r and P.E.=−14πε0e2r
View SolutionA solid metallic sphere of radius a is surrounded by a conducting spherical shell of radius b(b>a). The solid sphere is given a charge Q. A student measures the potential at the surface of the solid sphere as V and the potential at the surface of spherical shell as Vb. After taking these readings, he decides . to put charge of −4Q on the shell. He then noted the readings of the potential of solid sphere and the shell and found that the potential difference is △V .
He then connected the outer spherical shell to the earth by a conducting wire and found that the charge on the outer surface of the shell as He then decides to remove the earthing connection from the shell and earthed the inner solid sphere. Connecting the inner sphere with the earth he observes the charge on the solid sphere as q2. He then wanted to check what happens if the two are connected by the conducting wire. So he removed the earthing connection and connected a conducting wire between the solid sphere and the spherical shelll. After the connections were made he found the charge on the outer shell as q3.
Potential difference (△CV) measured by the student between the inner solid shere and outer shell after putting a charge −4Q is
View SolutionSimplified model of electron energy levels for a cartain atom is shown in figure. The atom is bombarded will fast moving electrons. The impact of one of these electrons can cause the removal of electron from K-level , thus, creating a vacancy in the K-level. This vacancy in K-level is filled by an electron from l-level and the energy reased in this transition can either appear as electromagnetic waves or may all be used knock out an electron from M-level of the atom.
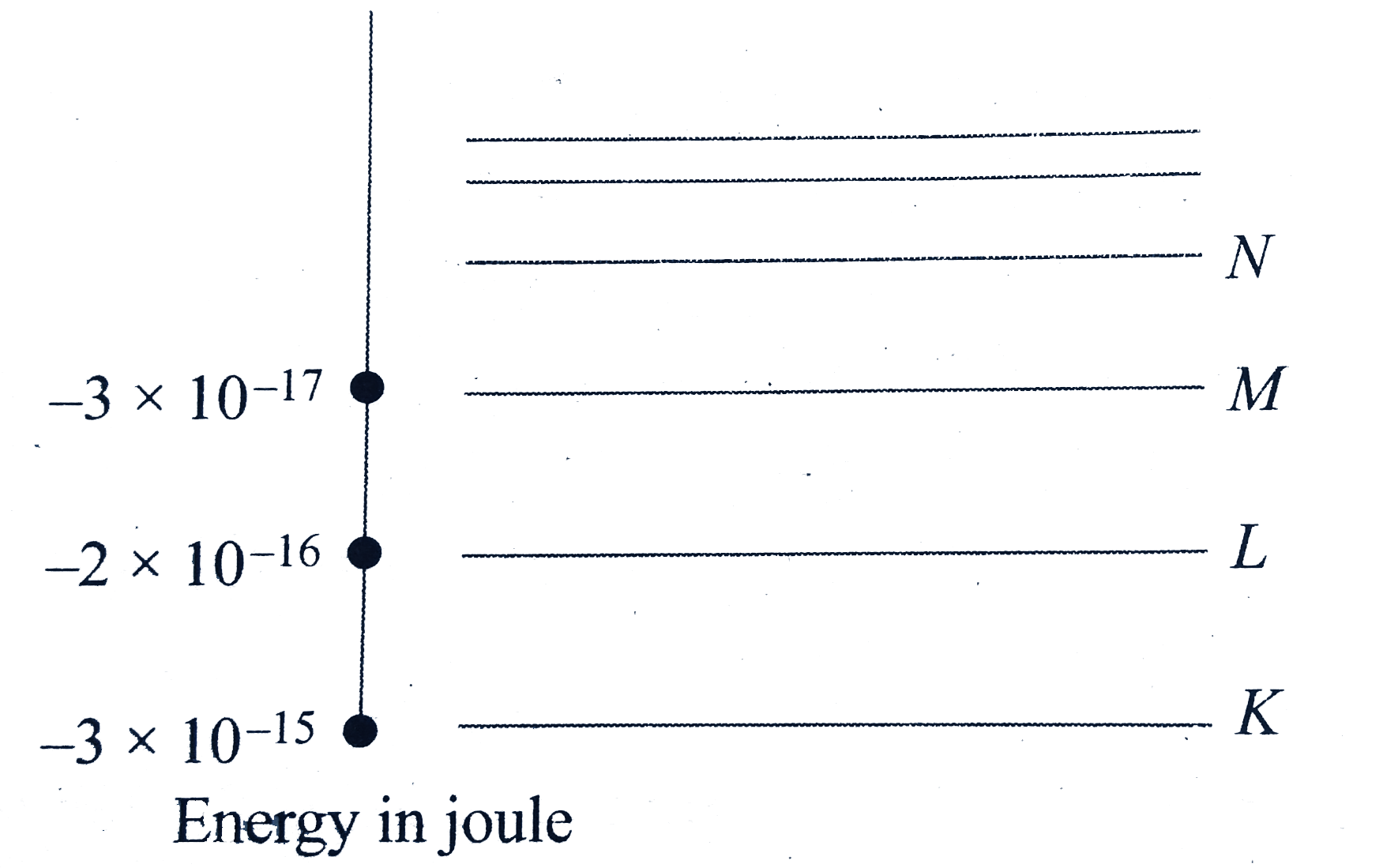
Based on above information, answer the following question:
The minimum potential difference through which bombarding electron beam must be acclerated from rest to cause the ejection of electron from K-level is.
View SolutionSimplified model of electron energy levels for a cartain atom is shown in figure. The atom is bombarded will fast moving electrons. The impact of one of these electrons can cause the removal of electron from K-level , thus, creating a vacancy in the K-level. This vacancy in K-level is filled by an electron from l-level and the energy reased in this transition can either appear as electromagnetic waves or may all be used knock out an electron from M-level of the atom.
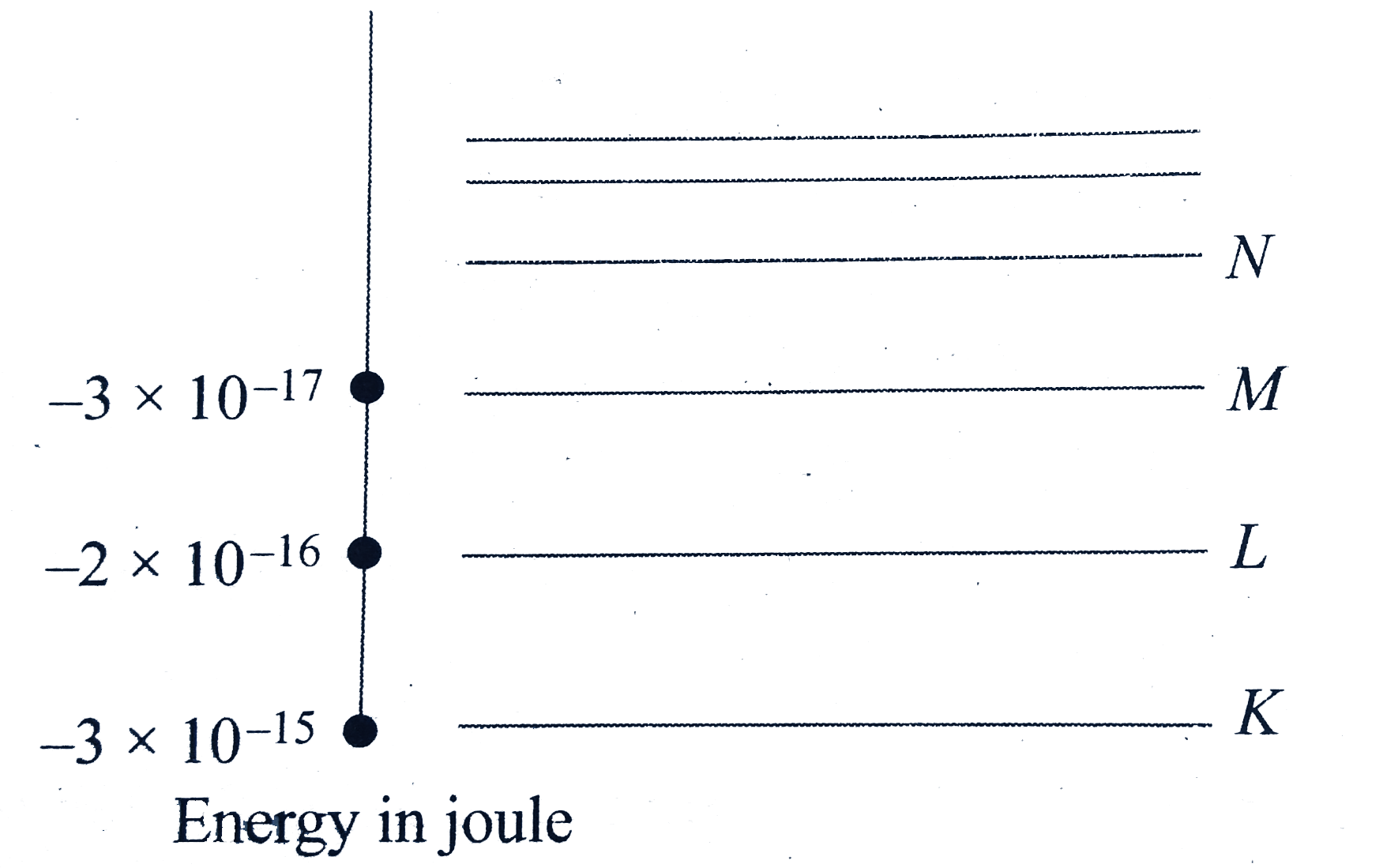
Based on above information, answer the following question:
Wavelength of the electromagnetic waves emitted due to transition from L to K-level is
View Solution
