Two objects of massses m1 and m2 having the same size are dropped simultaneously from hights h1andh2 respectively. Find out the ratio of time they would take in reaching the ground. Will this ratio remain the same if (i)one of the objects is hollow and the other one is solid and (ii)both of them are hollow, size remaining the same in each case. give reason.
To solve the problem, we will follow these steps:
Step 1: Understand the problem
We have two objects with masses m1 and m2 that are dropped from heights h1 and h2 respectively. We need to find the ratio of the time taken by each object to reach the ground.
Step 2: Use the equations of motion
We will use the second equation of motion, which states:
s=ut+12at2
In our case, since the objects are dropped (not thrown), the initial velocity u=0. The distance s will be the height from which the objects are dropped, and the acceleration a will be g (acceleration due to gravity).
Step 3: Set up the equation for each object
For the first object dropped from height h1:
h1=0⋅t1+12gt21
This simplifies to:
h1=12gt21
Rearranging gives:
t21=2h1g
Taking the square root:
t1=√2h1g
For the second object dropped from height h2:
h2=0⋅t2+12gt22
This simplifies to:
h2=12gt22
Rearranging gives:
t22=2h2g
Taking the square root:
t2=√2h2g
Step 4: Find the ratio of time taken
Now, we can find the ratio of the times t1 and t2:
t1t2=√2h1g√2h2g
The √2 and √g cancel out:
t1t2=√h1h2
Step 5: Analyze the effect of mass and shape
Now we need to determine whether this ratio changes if one object is hollow and the other is solid, or if both are hollow.
1. If one object is hollow and the other is solid: The acceleration due to gravity g is independent of the mass and shape of the object. Therefore, the ratio t1t2 will remain the same.
2. If both objects are hollow: Again, since the acceleration due to gravity is independent of the mass and shape, the ratio t1t2 will still remain the same.
Final Answer:
The ratio of the time taken by the two objects to reach the ground is:
t1t2=√h1h2
This ratio remains the same regardless of whether one object is hollow and the other is solid or if both are hollow.
We have two objects with masses m1 and m2 that are dropped from heights h1 and h2 respectively. We need to find the ratio of the time taken by each object to reach the ground.
We will use the second equation of motion, which states:
s=ut+12at2
In our case, since the objects are dropped (not thrown), the initial velocity u=0. The distance s will be the height from which the objects are dropped, and the acceleration a will be g (acceleration due to gravity).
For the first object dropped from height h1:
h1=0⋅t1+12gt21
This simplifies to:
h1=12gt21
Rearranging gives:
t21=2h1g
Taking the square root:
t1=√2h1g
h2=0⋅t2+12gt22
This simplifies to:
h2=12gt22
Rearranging gives:
t22=2h2g
Taking the square root:
t2=√2h2g
Now, we can find the ratio of the times t1 and t2:
t1t2=√2h1g√2h2g
The √2 and √g cancel out:
t1t2=√h1h2
Now we need to determine whether this ratio changes if one object is hollow and the other is solid, or if both are hollow.
The ratio of the time taken by the two objects to reach the ground is:
t1t2=√h1h2
This ratio remains the same regardless of whether one object is hollow and the other is solid or if both are hollow.
Topper's Solved these Questions
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - GRAVITATIONEXERCISE - SHORT ANSWER TYPE QUESTIONS7videos
GRAVITATION
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - FORCE AND LAW OF MOTIONEXERCISE - LONG ANSWER3videos
FORCE AND LAW OF MOTION
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - MOTIONEXERCISE - Long Answer Type Questions6videos
MOTION
Similar Questions
Two bodies of masses m1andm2 fall from heights h1andh2 respectively. The ratio of their velocities when the hit the ground is
View SolutionTwo bodies of masses (m1) and (m2) are droppded from heithts h1 and h2, respectively. They reach the ground after time t1 and t2 and strike the ground with v1 and v2, respectively Choose the correct relations from the following.
View SolutionA solid sphere and a solid cylinder of same mass are rolled down on two inclined planes of heights h1 and h2 respectively. If at the bottom of the plane the two objects have same linear velocities, then the ratio of h1:h2 is
View SolutionThree different objects of masses m1,m2 and m2 are allowed to fall from rest and from the same point O along three different frictionless paths. The speeds of three objects on reaching the ground will be:
View SolutionTwo cars of mass m1andm2 are moving in circle of radii r1andr2 , respectively . Their speeds are such that they make complete circles in the same time t . The ratio of their centripetal acceleration is :
View SolutionTwo cars of mass m1andm2 are moving in circle of radii r1andr2 , respectively . Their speeds are such that they make complete circles in the same time t . The ratio of their centripetal acceleration is :
View SolutionThere are two Cu spheres having same size. One of them is hollow and the other one is solid. Which will expand more if
(i) they are heated through same temperature range.
(ii) they are supplied with same amount of heat.View SolutionTwo racing cars of masses m1 and m2 are moving in circles of radii r1 and r2 respectively. Their speeds are such that each makes a complete circle in the same time t. The ratio of the angular speed of the first to the second car is
View SolutionTwo cars of masses m1 andm2 are moving in circles od radii r1andr2. Their speeds are such that they complete one revolution in the same time. The ratio of their angular speed is :
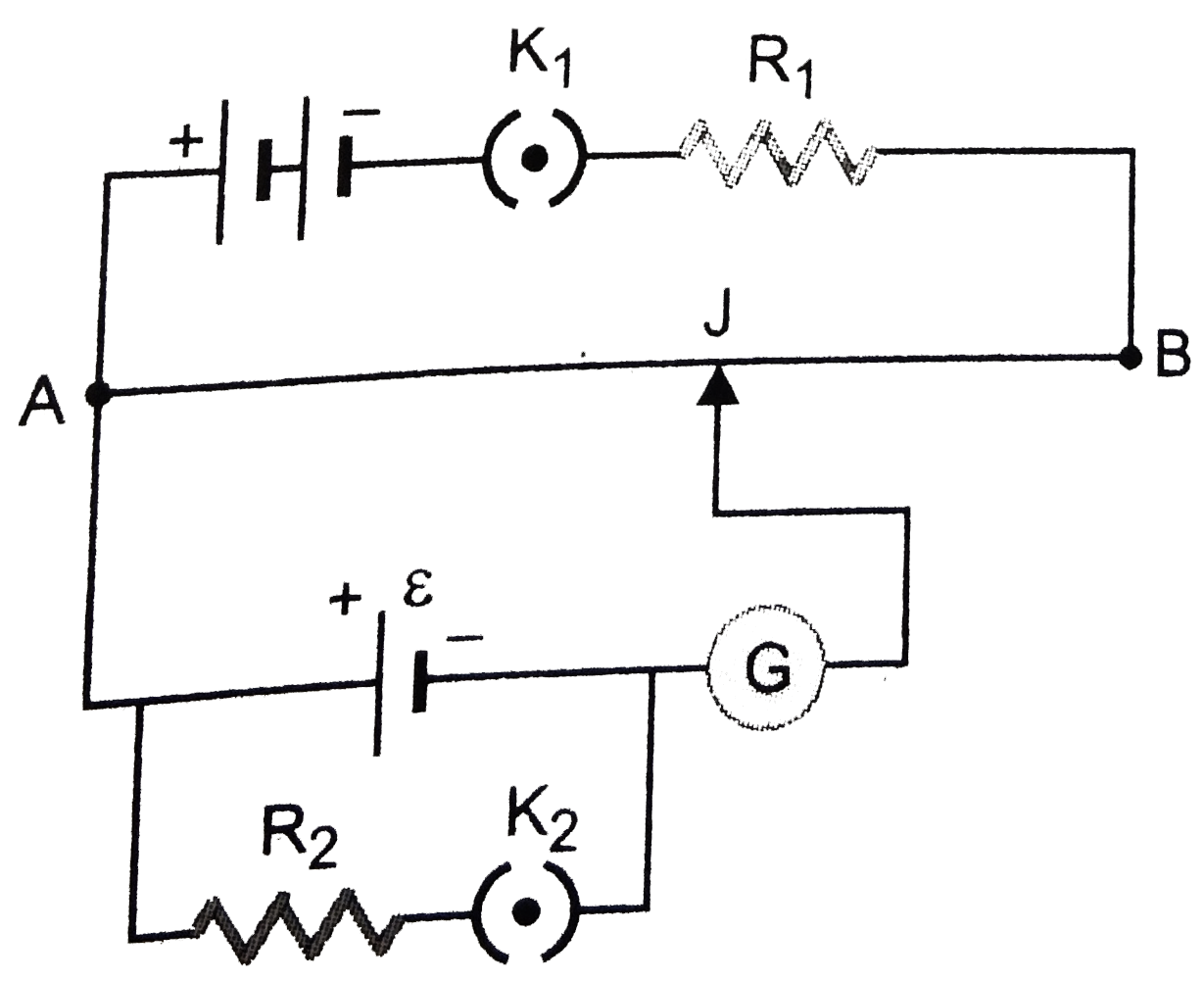
View SolutionFor the circuit would the balancing length increase, decrease or remain the same if
(i) R1 is decreased
(ii) R2 is increased, without any change (in each case) in the rest of the circuit ?
Justify your answer in each case.
 View Solution
View Solution

